 Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Phenomenological thermodynamics and the structure formation mechanism of the CuTi2S4 rhombohedral phase
Michail V.
Talanov
*a,
Vladimir B.
Shirokov
ab and
Valery M.
Talanov
c
aSouth Federal University, Rostov-on-Don, Russia. E-mail: tmikle-man@mail.ru
bSouth Scientific Center, Russian Academy of Sciences, 344006, Rostov-on-Don, Russia
cSouth Russia State Polytechnical University, Novocherkassk Polytechnical Institute, 346400, Novocherkassk, Russia. E-mail: valtalanov@mail.ru
First published on 19th February 2016
Abstract
The theory of structural phase transition in CuTi2S4 is proposed. The symmetry of order parameters, thermodynamics and the mechanism of the atomic structure formation of the rhombohedral Cu–Ti–thiospinel have been studied. The critical order parameter inducing the phase transition has been found. Within the Landau theory of phase transitions, it is shown that the phase state may change from the high-symmetry cubic disordered Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m phase to the low-symmetry ordered rhombohedral R
m phase to the low-symmetry ordered rhombohedral R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m phase as a result of phase transition of the first order close to the second order. It is shown that the rhombohedral structure of CuTi2S4 is formed as a result of the displacements of all types of atoms and the ordering of Cu-atoms (1
m phase as a result of phase transition of the first order close to the second order. It is shown that the rhombohedral structure of CuTi2S4 is formed as a result of the displacements of all types of atoms and the ordering of Cu-atoms (1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 order type in tetrahedral spinel sites), Ti-atoms (1
1 order type in tetrahedral spinel sites), Ti-atoms (1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1
1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6 order type in octahedral spinel sites), and S-atoms (1
6 order type in octahedral spinel sites), and S-atoms (1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1
1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3
3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3 order type). The Cu- and Ti-atoms form metal nanoclusters which are named a “bunch” of dimers. The “bunch” of dimers in CuTi2S4 is a new type of self-organization of atoms in frustrated spinel-like structures. It is shown that Ti-atoms also form other types of metal nanoclusters: trimers and tetrahedra.
3 order type). The Cu- and Ti-atoms form metal nanoclusters which are named a “bunch” of dimers. The “bunch” of dimers in CuTi2S4 is a new type of self-organization of atoms in frustrated spinel-like structures. It is shown that Ti-atoms also form other types of metal nanoclusters: trimers and tetrahedra.
1. Introduction
Copper-containing thiospinels exhibit a wide variety of physical properties that make them interesting from a scientific point of view.1–3 For example, large negative magnetoresistance is observed in the ferromagnetic thiospinel compound CuCrZrS4.4 The other spinels CuRh2S4 and CuRh2Se4 are superconductors.5–9 It is interesting to note that CuRh2S4 is the first compound with the pressure-induced superconductor–insulator transition, which occurs between 5.0 and 5.6 GPa.10 The iridium thiospinel CuIr2S4 shows a temperature-induced metal–insulator transition with simultaneous charge ordering and spin dimerization transition which is a rare phenomenon in three-dimensional compounds.11–14Among copper-thiospinels, CuTi2S4 has attracted great interest due to the metallic behavior and the weak magnetism of both Cu ions at the tetrahedral A-site and Ti ions at the octahedral B-site. The strong crystal field scheme leads the ground state of Cu2+ and Ti3+ to (eg)4(t2g)5 (S = 0) and (t2g)1 (S = 1/2) electronic configurations, respectively.15 Below 5 K, the copper-thiospinel transforms into a spin singlet state.15 The cubic thiospinel CuTi2S4 can be formally described in another way as Cu+Ti3+Ti4+S4 with non-magnetic Cu+ and mixed-valence Ti (Ti3+ and Ti4+).1 The electronic, magnetic and structural properties of this compound are, however, not sufficiently understood at present.
There is another reason for having interest in CuTi2S4: in three dimensional geometrically frustrated magnets with two-dimensional kagome-layers and triangular-sublattices, there has been a recent focus on the presence of a quantum spin liquid and/or heavy fermion-like states. It is assumed that such situation is realized in CuTi2S4.16
The structure of CuTi2S4 depends on the method of synthesis. According to ref. 17 the CuTi2S4 compound has a normal spinel structure at room temperature, where Cu ions occupy the tetrahedral site and Ti ions occupy the octahedral site, which crystallize into a cubic Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m structure. CuTi2S4 shows the Pauli paramagnetism, and there is no structural phase transition down to 8.3 K.17 Hence, according to ref. 18 CuTi2S4 has the low-symmetry rhombohedral spinel modification with the centrosymmetric space group R
m structure. CuTi2S4 shows the Pauli paramagnetism, and there is no structural phase transition down to 8.3 K.17 Hence, according to ref. 18 CuTi2S4 has the low-symmetry rhombohedral spinel modification with the centrosymmetric space group R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m which is irreversibly transformed into the cubic spinel at temperatures above 450 °C.18 Kinetically stabilized and rhombohedrally modified CuTi2S4 has been synthesized by the reaction of the constituent elements using eutectic alkali metal halide fluxes.18 According to the electronic structure calculation based on the density functional theory the rhombohedral thiospinel is energetically preferred, with a lower total energy of 1.6 eV per formula unit.18
m which is irreversibly transformed into the cubic spinel at temperatures above 450 °C.18 Kinetically stabilized and rhombohedrally modified CuTi2S4 has been synthesized by the reaction of the constituent elements using eutectic alkali metal halide fluxes.18 According to the electronic structure calculation based on the density functional theory the rhombohedral thiospinel is energetically preferred, with a lower total energy of 1.6 eV per formula unit.18
However, this rhombohedral modification of CuTi2S4 arouses scientific interest not only as a material with promising physical properties, but also as a crystal with a possible unique structure and electronic organization explained in this thesis. The structure of R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-modification of CuTi2S4 has atom distribution on Wyckoff positions similar to atom distribution in the high-order structure of AlV2O4. This high-order structure comprises complex “molecular” clusters – V-heptamers.19 Large building blocks such as V-heptamers are a consequence of cooperation between charge, spin and orbital degrees of freedom of the V ions.19,20 Hence, it is not clear whether it is possible to expect the formation of unique Ti-heptamers (analogue of V-heptamers) in CuTi2S4 rhombohedral modification as a result of the same type of self-organization of atoms, charges, spins and orbitals.
m-modification of CuTi2S4 has atom distribution on Wyckoff positions similar to atom distribution in the high-order structure of AlV2O4. This high-order structure comprises complex “molecular” clusters – V-heptamers.19 Large building blocks such as V-heptamers are a consequence of cooperation between charge, spin and orbital degrees of freedom of the V ions.19,20 Hence, it is not clear whether it is possible to expect the formation of unique Ti-heptamers (analogue of V-heptamers) in CuTi2S4 rhombohedral modification as a result of the same type of self-organization of atoms, charges, spins and orbitals.
The purpose of this study was to reveal symmetry, structural, and thermodynamic features of the formation of the low-symmetry rhombohedral phase with unusual metal nanoclusters from the cubic CuTi2S4-phase with a spinel structure. In this paper, we have mainly focused on the self-organization of atoms. We are the first to study this question with the help of group-theoretical, thermodynamic and structural methods of the modern theory of phase transitions. These methods were described earlier in detail.21–29 We have also checked the separate results obtained by our methods by means of the ISOTROPY program.30,31 The problem of self-organization of charges, spins and orbitals in the R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-modification of CuTi2S4 is supposed to be considered later.
m-modification of CuTi2S4 is supposed to be considered later.
2. Theory of the structural phase transition in CuTi2S4
2.1. Symmetry of the order parameter
Using the results of group-theoretical analysis of the phase transitions occurring according to one critical irreducible representation (irrep) in the group Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m, which satisfy the Lifshitz criterion,32 we find that the space group R
m, which satisfy the Lifshitz criterion,32 we find that the space group R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m may be generated by three-dimensional irrep k11(τ7)(1) as well as two six-dimensional irreps k10(τ1)(4) and k10(τ3)(4) and two four-dimensional irreps k9(τ1)(8,2) and k9(τ4)(8,2). The indexing of wave vectors and irreps is given according to Kovalev:33kj(τi) – the star of wave vectors kj, i – the number corresponding to irrep τ for a given star j. The multiplication in the primitive cell volumes as a result of phase transitions from the high-symmetry Fd
m may be generated by three-dimensional irrep k11(τ7)(1) as well as two six-dimensional irreps k10(τ1)(4) and k10(τ3)(4) and two four-dimensional irreps k9(τ1)(8,2) and k9(τ4)(8,2). The indexing of wave vectors and irreps is given according to Kovalev:33kj(τi) – the star of wave vectors kj, i – the number corresponding to irrep τ for a given star j. The multiplication in the primitive cell volumes as a result of phase transitions from the high-symmetry Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-phase to the low-symmetry R
m-phase to the low-symmetry R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-phase is shown in parentheses.
m-phase is shown in parentheses.
The results of group-theoretical analysis show that only in the case of the phase generated by irrep k9(τ4) the calculated distribution of the atoms on Wyckoff positions of the R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-phase is consistent with experimental data18 (Table 1). The expressions are explained in this table. For example, the record of 1(8):m + 1(24):2/m means that Wyckoff position 16d of the group Fd
m-phase is consistent with experimental data18 (Table 1). The expressions are explained in this table. For example, the record of 1(8):m + 1(24):2/m means that Wyckoff position 16d of the group Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m in the low-symmetry phase with the space group R
m in the low-symmetry phase with the space group R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m stratifies into one eight-fold Wyckoff position with local symmetry m and one 24-fold Wyckoff position with local symmetry 2/m.
m stratifies into one eight-fold Wyckoff position with local symmetry m and one 24-fold Wyckoff position with local symmetry 2/m.
![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m group symmetry induced low-symmetry rhombohedral R
m group symmetry induced low-symmetry rhombohedral R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-phases. Designations for order parameters: k9 – η, k10 – φ, k11 – ξ, V/V0 is the change in the primitive cell volume as a result of the structural phase transition
m-phases. Designations for order parameters: k9 – η, k10 – φ, k11 – ξ, V/V0 is the change in the primitive cell volume as a result of the structural phase transition
| Irreps | Order parameters | V/V0 | Stratification of the spinel Wyckoff positions in the R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-low-symmetry phases m-low-symmetry phases |
||
|---|---|---|---|---|---|
| 8a | 16d | 32e | |||
| k11(τ7) | ξ ξ ξ | 1 | 1(16):3m | 1(8):m + 1(24):2/m | 1(16):3m + 1(48):m |
| k10(τ1) | φ φ φ φ φ φ | 4 | 1(4):3m + 1(12):m + 1(12):m + 1(12):2 | 1(2):m + 1(6):2/m | 1(4):3m + 3(12):m + 1(24):1 |
| k10(τ3) | φ φ φ φ φ φ | 4 | 1(4):3m + 1(12):m + 1(12):m + 1(12):2 | 1(2):m + 1(6):2/m | 1(4):3m + 3(12):m + 1(24):1 |
| k9(τ1) | 0 η η η | 8 | 2(2):3m + 2(6):m + 2(6):m + 1(12):1 | 2(1):m + 2(3):2/m | 2(2):3m + 6(6):m + 2(12):1 |
| k9(τ1) | η 0 0 0 | 2 | 2(8):3m | 1(8):m + 2(12):2/m | 2(8):3m + 2(24):m |
| k9(τ4) | 0 η η η | 8 | 2(2):3m + 2(6):m | 1(2):+3(6):m + 2(6):2 | 2(2):3m + 6(6):m + 2(12):1 |
| k9(τ4) | 0 0 0 η | 2 | 2(8):3m | 2(4):![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m + 1(24):m m + 1(24):m |
2(8):3m + 2(24):m |
A decisive circumstance in the choice of critical irrep and low-symmetry solution in the description of phase transition in CuTi2S4 is the experimentally established ZB (R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m) = 12, where ZB is the number of formula units in the Bravais cell.18 The basis vectors of the unit cell A1, A2, A3 of the rhombohedral R
m) = 12, where ZB is the number of formula units in the Bravais cell.18 The basis vectors of the unit cell A1, A2, A3 of the rhombohedral R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-phase are connected with the basis vectors of the cubic spinel structure A1sp., A2sp., A3sp. by relations: A1 = 1/2(A1sp. − A3sp.), A2 = 1/2(−A1sp. + A2sp.), A3 = 2(A1sp. + A2sp. + A3sp.). The primitive cubic cell of the spinel contains two formula units; therefore, the primitive cell volume for the rhombohedral phase is larger than the primitive-cell volume for the cubic spinel by a factor of 2, i.e. it contains four formula units. The results are as follows: for the space group Fd
m-phase are connected with the basis vectors of the cubic spinel structure A1sp., A2sp., A3sp. by relations: A1 = 1/2(A1sp. − A3sp.), A2 = 1/2(−A1sp. + A2sp.), A3 = 2(A1sp. + A2sp. + A3sp.). The primitive cubic cell of the spinel contains two formula units; therefore, the primitive cell volume for the rhombohedral phase is larger than the primitive-cell volume for the cubic spinel by a factor of 2, i.e. it contains four formula units. The results are as follows: for the space group Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m ZP = 2, ZB = 8 and for the space group R
m ZP = 2, ZB = 8 and for the space group R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m ZP = 4, ZB = 12. Hereafter, ZP is the number of formula units in the primitive cell. These theoretical results also are consistent with experimental data.18
m ZP = 4, ZB = 12. Hereafter, ZP is the number of formula units in the primitive cell. These theoretical results also are consistent with experimental data.18
Thus, the critical irrep inducing phase transition in CuTi2S4 is the four-dimensional irrep of star k9(τ4). Irrep k9(τ4) corresponds to irrep L2− in notations of Stokes and Hatch, 2007. A one-parameter solution (0 0 0 η) corresponds to the experimentally established phase (Table 2).
![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m. The superscript index in the structural formula means the type of Wyckoff position according to international tables for crystallography
m. The superscript index in the structural formula means the type of Wyckoff position according to international tables for crystallography
| No. | Order parameter | Symbol of space group | V/V0 | Translations of primitive cell of spinel structure | Structural formula |
|---|---|---|---|---|---|
| 1 | (0 0 η η) | Cmcm (N63) | 4 | 2a2 + 2a3 − 2a1, 2a3 + 2a1 − 2a2, 2a1 + 2a2 − 2a3 | (Ac)2Ag2(Bg2)2Bf2Be2(Xg2)4(Xf2)2Xh |
| 2 | (η η η η) |
F![[4 with combining macron]](https://www.rsc.org/images/entities/char_0034_0304.gif) 3m (N216) 3m (N216) |
8 | 2a2 + 2a3 − 2a1, 2a3 + 2a1 − 2a2, 2a1 + 2a2 − 2a3 | Aa(Ae4)2Ag6Ab(Be4)2(Bh12)2(Xe4)4(Xh12)4 |
| 3 | (0 0 0 η) |
R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m (N166) m (N166) |
2 | a 2 − a1, a1, 2a1 + 2a2 + 2a3 | (Ac1/2)2Ba1/4Bb1/4Bh3/2(Xc1/2)2(Xh3/2)2 |
| 4 | (0 η η η) |
R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m (N166) m (N166) |
8 | 2a3, −2a2, 6a2 − 2a1 − 2a3 | (Ac1/8)2(Ah3/8)2(Bh3/8)3Bc1/8Bg3/8Bf3/8(Xh3/8)6Xc1/8Xc3/8(Xi6/8)2 |
| 5 | (0 η1η1η2) | C2/m (N12) | 8 | 4a2 − 2a3, 2a3, 2a1 − 2a2 | (Ai1/8)4(Aj2/8)2(Bj2/8)4(Bi1/8)4Bh1/8Bg1/8(Xj2/8)12(Xi1/8)8 |
| 6 | (η1η2η2η2) | R3m (N160) | 8 | 2a1, 2a1 − 2a3, 6a2 − 2a1 − 2a3 | (Aa1/16)4(Ab3/16)4(Bb3/16)6(Ba1/16)2(Bc6/16)2(Xb3/16)12(Xa1/16)4(Xc6/16)4 |
| 7 | (η1η1η2η2) | Imm2 (N44) | 8 | 2a3, 2a1 − 2a2, 2a1 + 2a2 − 2a3 | (Aa1/16)2(Ac2/16)2Ae4/16(Ad2/16)2(Ab1/16)2(Bc2/16)4(Bd2/16)4(Be4/16)4(Xc2/16)8(Xd2/16)8(Xe4/16)8 |
| 8 | (0 0 η1η2) | C21/m (N11) | 4 | a 1 + a2, a2 − a1, 2a3 − a1 − a2 | (Ae1/4)4(Be1/4)4(Bf2/4)2(Xe1/4)8(Xf2/4)4 |
| 9 | (η1η2η2η3) | Cm (N8) | 8 | 4a2 − 2a3, 2a3, 2a1 − 2a2 | (Aa1/16)8(Ab2/16)4(Bb2/16)12(Ba1/16)8(Xb2/16)24(Xa1/16)16 |
| 10 | (0 η1η2η3) | Pi (N2) | 8 | 2a1, 2a2, 2a3 | (Ai1/8)8(Bi1/8)16(Xi1/8)32 |
| 11 | (η1η2η3η4) | P1 (N1) | 8 | 2a1, 2a2, 2a3 | (Aa1/16)16(Ba1/16)32(Xa1/16)64 |
The four-component order parameter (OP), transformed according to irrep k9(τ4) of Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m group symmetry, forms a point group of order 384 in four-dimensional space. The OP transformation properties are given by the following generator matrices:
m group symmetry, forms a point group of order 384 in four-dimensional space. The OP transformation properties are given by the following generator matrices:
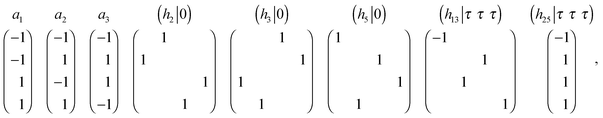 | (1) |
![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m group, τi is the accompanying nontrivial translation of hi, and a1, a2, a3 are basic vectors of the primitive cell. In eqn (1) the generators of irrep k9(τ4) of Fd
m group, τi is the accompanying nontrivial translation of hi, and a1, a2, a3 are basic vectors of the primitive cell. In eqn (1) the generators of irrep k9(τ4) of Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m group symmetry are given. The remaining irrep k9(τ4) matrices corresponding to other elements of Fd
m group symmetry are given. The remaining irrep k9(τ4) matrices corresponding to other elements of Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m group symmetry can be obtained as a result of multiplication of the above-mentioned matrices. Designations for symmetry elements of the Fd
m group symmetry can be obtained as a result of multiplication of the above-mentioned matrices. Designations for symmetry elements of the Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m space group are given according to Kovalev.33
m space group are given according to Kovalev.33
Table 2 lists the space groups of all possible low-symmetry phases induced by irrep k9(τ4), and corresponding components of the four-dimensional OP. The multiplication of primitive cell volumes as a result of the structural phase transitions (V0/V), the vectors of primitive cell translations of low-symmetry phases (a1, a2, a3) and the structure formulas of low-symmetry phases are also presented. All these types of solutions are necessary for constructing possible phase diagrams, for establishing the thermodynamic nature of the phase transition under study, and for predicting new possible phase states in CuTi2S4 and structurally related materials. The list of low-symmetry phases (Table 2) is consistent with the results obtained in ref. 34.
It is interesting to note that among the 11 low-symmetrical phases, there are two one-parameter phases with the same space group R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m. However, the structures of these isosymmetrical phases differ significantly.
m. However, the structures of these isosymmetrical phases differ significantly.
2.2. Phase diagram
The basis of invariants of the thermodynamic potential consists of 43 monomials that do not exceed the eighth degree:| J1 = η12 + η22 + η32 + η42, |
| J2 = η12η22 + η12η32 + η12η42 + η22η32 + η22η42 + η32η42, |
| J3 = η12η22η32 + η12η22η42 + η12η32η42 + η22η32η42, |
| J4 = η12η22η32η42 | (2) |
The invariant basis (2) was constructed using the algorithm described in ref. 35. A phenomenological thermodynamic model of phase transitions will be built taking into account the thermodynamic potential stability.36–39 The term “stable” refers to the potential that allows us to construct a phase diagram that does not change due to a small external perturbation to the potential. A small perturbation should lead to only small quantitative changes without changing the type, the number of phases and the topology of the phase diagrams.
To build a “stable” thermodynamic potential the type of multi-critical point should be specified.37 The “stable” thermodynamic potential of the sixth degree is only possible if the critical point is determined by the lack of invariants J1 and J12. A stable potential has the form:
 | (3) |
![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-phase.
m-phase.
As in thermodynamic potential (3) there is no invariant of the third degree on components of the OP, any phenomenological model of a phase transition with the OP which has symmetry (1) will describe only phase transitions of the second order between the high-symmetry phase and low-symmetry phases bordering it.
The point a1 = a2 = 0 in the phase diagram (Fig. 1) is a tricritical point (TCP). At this point, the line of phase transitions of the second order continuously passes through the line of phase transitions of the first order. It is important to emphasize that the first order of the phase transition from the Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-phase to the R
m-phase to the R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-phase at a2 < 0 is caused by the sixth degree of order parameter components but not by the symmetry of the system. The irreversibility of the phase transition in CuTi2S4 established experimentally18 indicates that in this substance there is a phase transition Fd
m-phase at a2 < 0 is caused by the sixth degree of order parameter components but not by the symmetry of the system. The irreversibility of the phase transition in CuTi2S4 established experimentally18 indicates that in this substance there is a phase transition Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m → R
m → R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m of the first order in the vicinity of the TCP in the region of the phase diagram a2 < 0.
m of the first order in the vicinity of the TCP in the region of the phase diagram a2 < 0.
 | ||
| Fig. 1 Phase diagram described by thermodynamic potential (3). The diagram in the vicinity of the tricritical point (TCP) for the case where b2 > 0 and b12 > 0. The lines of the first- and second-order transitions are indicated by solid and dashed lines, respectively. The region of the phase diagram decorated with two colors is the two-phase region. | ||
2.3. Structural mechanism of rhombohedral modification formation
The structural mechanism of formation of low-symmetry modifications of crystals is determined by the ordering of atoms and their displacements in the initial phase structure.The Cu-atoms occupy Wyckoff position 8a (site symmetry ![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m), Ti – Wyckoff position 16d (site symmetry
m), Ti – Wyckoff position 16d (site symmetry ![[4 with combining macron]](https://www.rsc.org/images/entities/char_0034_0304.gif) 3m), sulfur – Wyckoff position 32e (site symmetry 3m) in the cubic Fd
3m), sulfur – Wyckoff position 32e (site symmetry 3m) in the cubic Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-phase of a normal spinel. The structural formula of the cubic Fd
m-phase of a normal spinel. The structural formula of the cubic Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-spinel is (Cu)8a[Ti2]16dS432e.
m-spinel is (Cu)8a[Ti2]16dS432e.
The critical irrep k9(τ4) enters into the mechanical representation and permutation representation of the spinel structure on Wyckoff positions 8a, 16d and 32e.21–24,27 This means that the low-symmetry phase formation is connected with displacements of tetrahedral and octahedral cations and anions and also with the ordering of all atom types. Group-theoretic analysis showed that the formation of the CuTi2S4 rhombohedral phase is accompanied by the following types of atom ordering:
– binary tetrahedral cation ordering (order type 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1);
1);
– ternary octahedral cation ordering (order type 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1
1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6);
6);
– quaternary anion ordering (order type 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1
1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3
3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3).
3).
As a result, the theoretical structure formula of a low-symmetry rhombohedral R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-spinel modification should be A2c1/2A2c1/2B1a1/4B1b1/4B6h3/2X2c1/2X2c1/2X6h3/2X6h3/2 (rhombohedral presentation) or A6c1/2A′6c1/2B3a1/4B3b1/4B18h3/2X6c1/2X′6c1/2X18h3/2X′18h3/2 (hexahedral presentation). For CuTi2S4 the latest structural formula is Cu(1)6c1/2Cu(2)6c1/2Ti(1)3a1/4Ti(2)3b1/4Ti(3)18h3/2S(1)6c1/2S(2)6c1/2S(3)18h3/2S(4)18h3/2. Our theoretical structural formula agrees with experimental data.18
m-spinel modification should be A2c1/2A2c1/2B1a1/4B1b1/4B6h3/2X2c1/2X2c1/2X6h3/2X6h3/2 (rhombohedral presentation) or A6c1/2A′6c1/2B3a1/4B3b1/4B18h3/2X6c1/2X′6c1/2X18h3/2X′18h3/2 (hexahedral presentation). For CuTi2S4 the latest structural formula is Cu(1)6c1/2Cu(2)6c1/2Ti(1)3a1/4Ti(2)3b1/4Ti(3)18h3/2S(1)6c1/2S(2)6c1/2S(3)18h3/2S(4)18h3/2. Our theoretical structural formula agrees with experimental data.18
Note that the CuTi2S4 compound under consideration can have charge ordering in principle, because copper and titanium atoms, being in Wyckoff positions 8a and 16d of the spinel structure, occupy some different Wyckoff positions in rhombohedral spinel modification.
We have built the scalar and vector basic functions of irrep k9(τ4) which allowed us to deduce the rhombohedral R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-spinel structure from the spinel structure. We used the value of the free parameters for Wyckoff positions of atoms in rhombohedral spinel modification (which were taken from ref. 18) in order to build the R
m-spinel structure from the spinel structure. We used the value of the free parameters for Wyckoff positions of atoms in rhombohedral spinel modification (which were taken from ref. 18) in order to build the R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-spinel structure. The results of theoretical calculations of the CuTi2S4 atom structure are given below (Fig. 2).
m-spinel structure. The results of theoretical calculations of the CuTi2S4 atom structure are given below (Fig. 2).
2.4. Metal clusters in the structure of CuTi2S4
There are two independent Wyckoff positions for Cu, three for Ti (two Ti atoms are located in the triangular lattice [Ti(1) and Ti(2)], and one Ti atom [Ti(3)] is in the kagome lattice), and four for S in the rhombohedral R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m-spinel structure of the title compound (Fig. 2). This compound is isostructural with CuZr1.86(1)S4,40 with rhombohedral modification of AlV2O420 and with phase of high pressure of LiV2O4.41
m-spinel structure of the title compound (Fig. 2). This compound is isostructural with CuZr1.86(1)S4,40 with rhombohedral modification of AlV2O420 and with phase of high pressure of LiV2O4.41
A peculiar feature of the rhombohedral structure is metal clustering.
In contrast to the AlV2O4 rhombohedral structure, in which the formation of heptamers occurs by the ordering of vanadium atoms, located in octahedral sites of the initial cubic spinel, in the formation of metal clusters in the CuTi2S4 rhombohedral structure not only the octahedral titanium atoms but also tetrahedral atoms of copper take part (Fig. 3).
Atoms of Cu(1) and Ti(3) form a “bunch” of dimers (Fig. 3 and 4). Each “bunch” consists of three [Cu(1)–Ti(3)]-dimers which are joined by the common Cu(1)-atom. Each Cu(1) atom is surrounded by three Ti(3) atoms, resulting in three Cu(1)–Ti(3) interatomic distances of 2.876 Å. The shortest Cu–Ti distance in the cubic spinel is much longer and equal to 4.148 Å. This distance is too large for a significant interaction, while it is the shortest among all the bond lengths of the metal–metal bonds in the rhombohedral form.
On the kagome lattice, there are two kinds of Ti(3)–Ti(3) bonds (titanium dimers [Ti(3)2]) with different bond lengths: the shorter (dark blue) is 3.419 Å and the longer (red) is 3.606 Å (Fig. 5a and b). These dimers alternate in the kagome lattice (Fig. 5a). The distance Ti–Ti in the cubic form of CuTi2S4 is equal to 3.538 Å.
Ti(3)-atoms, belonging to the “bunch”, form two types of [Ti(3)3] trimers with interatomic distances Ti(3)–Ti(3) = 3.419 Å (Fig. 5a) and Ti(3)–Ti(3) = 3.606 Å (Fig. 3 and 5b).
Alternating trimers, having short and long bonds Ti(3)–Ti(3), form a kagome lattice (Fig. 5a). These trimers are at the basis of two types of tetrahedra: [Cu(1)Ti(3)3] and [Ti(1)Ti(3)3]. They have the opposite orientation (antiparallel) relative to the kagome-plane (Fig. 5b).
Each trimer, formed by bonds Ti(3)–Ti(3), is the basis for the metal tetrahedron with the shortest bonds: [Cu(1)Ti(3)]3 and [S(2)Cu(2)Ti(3)3] (Fig. 5c). Each trimer, formed by more longer bonds Ti(3)–Ti(3), is the basis for the metal tetrahedron [Ti(1)Ti(3)3]. Each [Cu(1)Ti(3)3]-tetrahedron through the Cu(1)-atom is surrounded by three S(1)-atoms and by [S(1)Cu(1)Ti(3)3]-tetrahedron (Fig. 5c).
In the rhombohedral structure of CuTi2S4 it is possible to distinguish Ti(1)-heptamers, which are analogs of V-heptamers in the structure of AlV2O419 (Fig. 6a). Indeed, there are two inequivalent triangular-lattice layers, composed of Ti(1)-atoms and Ti(2)-atoms, respectively. Here, all the Ti(1) atoms are sandwiched by two [Ti(3)3]-trimers (Fig. 6a), but none of the Ti(2) atoms are. Ti(2)-atoms are sandwiched by two [S(4)3]-trimers and S(4) atoms also constitute also one heptamer [Ti(2)S(4)6] (Fig. 6b). But these S(4)-heptamers are not analogs of V-heptamers in the structure of AlV2O4.
Binding between adjacent kagome-layers is carried out by using heptamers [Ti(1)Ti(3)6] (Fig. 6a, c and d) and sulfur atoms (Fig. 6c and d).
Previously, the authors18 suggested that the valence states of atoms in the CuTi2S4 rhombohedral form should be (Cu+)4Ti(1)4+Ti(2)3+Ti(3)3.5+6(S2−)16 according to the crystal-chemical analysis of the crystal bond lengths and electronic structure calculations. This formula means that the Ti(1)4+ ion has no t2g orbitals, i.e. there are no chemical bonding between Ti(1) and Ti(3) atoms. Therefore, the formation of Ti-heptamers is unlikely. Besides, the calculated densities of states of rhombohedral modifications show that Cu(1)–Ti(3) and Ti(3)–Ti(3) interactions in the “bunch” of dimers and [Ti(3)3] trimers (with interatomic distances Ti(3)–Ti(3) = 3.419 Å) have a bonding character.18
3. Results and discussion
For the first time we investigated the OP symmetry, thermodynamics, and structural mechanism of formation of the low-symmetry CuTi2S4 phase within a unified approach based on the Landau phenomenological theory of phase transitions.Based on the hypothesis of one critical representation, we calculated stratification of the Wyckoff positions 8a, 16d, and 32e of the initial phase with a cubic spinel structure upon transition to the low-symmetry rhombohedral modification. We also showed that the calculated structure of the CuTi2S4 rhombohedral phase is formed due to displacements of all types of atoms and the ordering of Cu-atoms (1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 order type in tetrahedral spinel sites), Ti-atoms (1
1 order type in tetrahedral spinel sites), Ti-atoms (1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1
1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 6 order type in octahedral spinel sites), and S-atoms (1
6 order type in octahedral spinel sites), and S-atoms (1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1
1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3
3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3 order type).
3 order type).
Crystallochemical features of the structure of the ordered rhombohedral modification were investigated theoretically, and the structural motifs of atomic and polyhedral short- and long-range orders were found. Our theoretical structural results agree well with the experimental data.18 We found that in the CuTi2S4 rhombohedral structure there are only one type of Ti-heptamers such as V-heptamers in AlV2O4.20 But these heptamers have very large bond lengths and therefore cannot be considered as metal “molecules” in the CuTi2S4 rhombohedral structure.
We first established the existence of a “bunch” of [Cu(1)–Ti(3)]-dimers, two types of Ti(3)-trimers [Ti(3)3] and two types tetrahedral clusters ([Cu(1)Ti(3)3] and [Ti(1)Ti(3)3]). Of particular interest are metal nanostructures: [Cu(1)–Ti(3)]-dimers, [Ti(3)3] trimers with the shorter bond lengths and a “bunch” of [Cu(1)–Ti(3)]-dimers. The metallic dimers and trimers in geometrically frustrated structures (for example dimers: MgTi2O4,43–45 CuTe2O5,46,47 VO2,48 CuGeO3;49 trimers: LiVO2,50 LiVS2,51 NaV6O10,52 BaV10O15,53–56 SrV8Ga4O19,57 AV13O18 (A = Ba, Sr),58 A2V13O22 (A = Ba, Sr),59 Ba4Ru3O1060) have long been known. The formation of a “bunch” of [Cu(1)–Ti(3)]-dimers, which are molecular-like clusters (metal “molecules”), in CuTi2S4 can be regarded as a new type of self-organization of atoms in the crystals.
The global pattern of changes in the phase states was considered within the model taking into account the terms in the free energy up to the sixth degree in the OP components in the Landau theory of phase transitions. It was shown first that phase transitions between different phases (Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m and R
m and R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m) may occur as a result of both second and first-order phase transitions in the vicinity of the tricritical point. In the case of CuTi2S4, we propose that phase transitions of the first order close to the second order in the vicinity of the TCP take place. At such structural transitions the thermodynamic state changes by jumping (and therefore they are phase transitions of the first order), but fundamental spinel structural transformation does not occur.42 These results are based on our calculations of the phase diagram and the experimental fact of irreversibility of the phase transition.18
m) may occur as a result of both second and first-order phase transitions in the vicinity of the tricritical point. In the case of CuTi2S4, we propose that phase transitions of the first order close to the second order in the vicinity of the TCP take place. At such structural transitions the thermodynamic state changes by jumping (and therefore they are phase transitions of the first order), but fundamental spinel structural transformation does not occur.42 These results are based on our calculations of the phase diagram and the experimental fact of irreversibility of the phase transition.18
It is interesting to note that the magnetization of some spinels normally takes place along the [111] direction and therefore could be responsible for a rhombohedral distortion. But, magnetic interactions are not the driving force for the phase transformation of the “cubic-rhombohedral” spinel in the CuTi2S4 case as both phases are Pauli paramagnets.18 The Pauli susceptibility of the R![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m form is larger than that of the thiospinel in quantitative agreement with the LMTO-ASA band structure calculations.18 The authors18 point out that a transition to the ferromagnetic state might occur below the temperature range investigated (2–300 K). The magnetic structure of CuTi2S4 near 2 K remains unknown.
m form is larger than that of the thiospinel in quantitative agreement with the LMTO-ASA band structure calculations.18 The authors18 point out that a transition to the ferromagnetic state might occur below the temperature range investigated (2–300 K). The magnetic structure of CuTi2S4 near 2 K remains unknown.
4. Conclusions
We first proposed a theory of the structural phase transition in CuTi2S4, built possible phase diagrams and established a structural mechanism of formation of the low-symmetry rhombohedral modification from the high-symmetry cubic spinel phase. The most important and unexpected result is the discovery of metal nanoclusters, which are different from the V-heptamers in the AlV2O4 structure.We believe that further studies will be connected with specifying the conditions for stability of metallic nanoclusters. For this it is necessary to perform detailed quantum mechanical calculations of the Cu and Ti valence states as well as the calculations of the spin and orbital ordering in the rhombohedral phase of CuTi2S4.
Acknowledgements
The authors thank the anonymous referees for valuable remarks. They are also very grateful to Professor Dr Katsuhiko Ariga for his kind interest and encouragement. The reported study was funded by RFBR, according to research project no. 16-32-60025 mol_a_dk (Talanov M. V.).References
- R. P. Van Stapele, Ferro-magnetic Materials, A Handbook on the properties of magnetically ordered Substances, Amsterdam, North-Hooland, 1982, vol. 3 Search PubMed.
- F. Hulliger, Structure and Bonding V.4., Springer, Berlin, Crystal Chemistry of the Chalcogenides and Pnictides of the Transition Elements, 1968, p. 83 Search PubMed.
- V. M. Talanov, Energetic Crystallochemistry of Multisublattice Crystals, Rostov-on-Don, RSU, 1986 Search PubMed.
- T. Furubayashi, H. Suzuki, N. Kobayashi and S. Nagata, Solid State Commun., 2004, 131, 505 CrossRef CAS.
- T. Bitoh, T. Hgino, Y. Seki, S. Chikazawa and S. Nagata, J. Phys. Soc. Jpn., 1992, 61, 3011 CrossRef CAS.
- T. Hagino, Y. Seki, N. Wada, S. Tsuji, T. Shirane, K. I. Kumagai and S. Nagata, Phys. Rev. B: Condens. Matter Mater. Phys., 1995, 51, 12673 CrossRef CAS.
- N. H. Van Maaren, G. M. Schaeffer and F. K. Lotgering, Phys. Lett., 1967, 25, 238 CrossRef.
- R. N. Shelton, D. C. Jhonston and H. Adrian, Solid State Commun., 1976, 20, 1077 CrossRef CAS.
- T. Shirane, T. Hgino, Y. Seki, S. Chikazawa and S. Nagata, J. Phys. Soc. Jpn., 1993, 62, 374 CrossRef CAS.
- M. Ito, J. Hori, H. Kurisaki, H. Okada, A. J. Perez Kuroki, N. Ogita, M. Udagawa, H. Fujii, F. Nakamura, T. Fijita and T. Suzuki, Phys. Rev. Lett., 2003, 91, 077001 CrossRef CAS PubMed.
- S. Nagata, T. Hagino, Y. Seki and T. Bitoh, Physica B, 1995, 194–196, 12673 Search PubMed.
- P. G. Radaelli, Y. Horibe, M. J. Gutmann, H. Ishibashi, C. H. Chen, R. M. Ibberson, Y. Koyama, Y.-S. Hor, V. Kiryukhin and S.-W. Cheong, Nature, 2002, 416, 155 CrossRef CAS PubMed.
- T. Furubayashi, T. Matsumoto, T. Hagino and S. Nagata, J. Phys. Soc. Jpn., 1994, 63, 3333 CrossRef CAS.
- N. Matsumoto, T. Hagino, K. Taniguchi, S. Chikazawa and S. Nagata, Physica B, 2000, 284–288, 1978 CrossRef CAS.
- T. Koyama, H. Sugita, S. Wada, K. Miyatani, T. Tanaka and M. Ishikawa, Physica B, 2000, 284–288, 1513 CrossRef CAS.
- M. Isoda and S. Mori, J. Phys. Soc. Jpn., 2000, 69, 1509 CrossRef CAS.
- H. Okada, K. Koyama, K. Watanabe and J. Alloys, Compounds, 2005, 403, 34 CrossRef CAS.
- N. Soheilnia, K. M. Kleinke, E. Dashjav, H. L. Cuthbert, J. E. Greedan and H. Kleinke, Inorg. Chem., 2004, 43, 6473 CrossRef CAS PubMed.
- Y. Horibe, M. Shingu, K. Kurushima, H. Ishibashi, N. Ikeda, K. Kato, Y. Motome, N. Furukawa, S. Mori and T. Katsufuji, Phys. Rev. Lett., 2006, 96, 086406 CrossRef CAS PubMed.
- M. Croft, V. Kiryukhin, Y. Horibe and S.-W. Cheong, New J. Phys., 2007, 9, 1 CrossRef.
- V. M. Talanov and V. B. Shirokov, Acta Crystallogr., Sect. A: Found. Crystallogr., 2012, 68, 595 CrossRef CAS PubMed.
- V. M. Talanov and V. B. Shirokov, Acta Crystallogr., Sect. A: Found. Crystallogr., 2014, 70, 49 CrossRef CAS PubMed.
- V. M. Talanov, V. B. Shirokov and M. V. Talanov, Acta Crystallogr., Sect. A: Found. Crystallogr., 2015, 71, 301 CrossRef CAS PubMed.
- V. M. Talanov, M. V. Talanov and V. B. Shirokov, Crystallogr. Rep., 2014, 59, 650 CrossRef CAS.
- V. M. Talanov and A. A. Mukovnin, Eur. Phys. J. B, 2013, 86, 448 CrossRef.
- A. A. Mukovnin and V. M. Talanov, Eur. Phys. J. B, 2014, 87, 34 CrossRef.
- V. P. Sahnenko, V. M. Talanov and G. M. Chechin, Fiz. Met. Metalloved., 1986, 62, 847 Search PubMed.
- V. M. Talanov and V. B. Shirokov, Kristallographiya, 2013, 58, 296 CrossRef.
- V. M. Talanov and V. B. Shirokov, Crystallogr. Rep., 2013, 58, 314 CrossRef CAS.
- H. T. Stokes and D. M. Hatch, 2007, ISOTROPY, http://stokes.byu.edu/iso/isotropy.html.
- H. T. Stokes, E. H. Kisi, D. M. Hatch and C. J. Howard, Acta Crystallogr., Sect. B: Struct. Sci., Cryst. Eng. Mater., 2002, 58, 934 CrossRef.
- L. D. Landau and E. M. Lifshitz, Statistical Physics, Part 1, Oxford, Pergamon, 1980 Search PubMed.
- O. V. Kovalev, in Representations of Crystallographic Space Groups. Irreducible Representations, Induced Representations and Co-representations, ed. H. T. Stokes and D. M. Hatch, Taylor and Francis Ltd, London, 1992, p. 349 Search PubMed.
- V. P. Sahnenko, V. M. Talanov and G. M. Chechin, Tomsk, Dep. VINITI No. 638-82, 1982, 25.
- V. B. Shirokov, Crystallogr. Rep., 2011, 56, 475 CrossRef CAS.
- A. M. Prokhorov, Yu. M. Gufan, E. S. Larin, E. G. Rudashevsry and V. B. Shirokov, DAN SSSR, 1984, 227, 1369 Search PubMed.
- E. I. Kut'in, V. L. Lorman and S. V. Pavlov, Usp. Fiz. Nauk, 1991, 34, 497 Search PubMed.
- T. Poston and I. Stewart, Catastrophe Theory and its Applications, Pitman, London-San Francisco-Melbourne, 1978 Search PubMed.
- V. I. Arnold, Catastrophe Theory, Springer-Verlag, Berlin, 3rd edn, 1992 Search PubMed.
- Y. Dong, M. A. McGuire, H. Yun and F. J. DiSalvo, J. Solid State Chem., 2010, 183, 606 CrossRef CAS.
- K. Takeda, H. Hidaka, H. Kotegawa, T. C. Kobayashi, K. Shimizu, H. Harima, K. Fujiwara, K. Miyoshi, J. Takeuchi, Y. Ohishi, T. Adachi, M. Takata, E. Nishibori, M. Sakata, T. Watanuki and O. Shimomura, Physica B, 2005, 359–361, 1312 CrossRef CAS.
- Yu. M. Gufan, Structural Phase Transitions, Nauka, Moscow, 1982, p. 304 Search PubMed.
- M. Schmidt, W. Ratcliff, P. G. Radaelli, K. Refson, N. M. Harrison and S.-W. Cheong, Phys. Rev. Lett., 2004, 92, 056402 CrossRef CAS PubMed.
- V. M. Talanov, V. B. Shirokov, V. V. Ivanov and M. V. Talanov, Crystallogr. Rep., 2015, 60(1), 101 CrossRef CAS.
- V. V. Ivanov, V. M. Talanov, V. B. Shirokov and M. V. Talanov, Inorg. Mater., 2011, 47(9), 990 CrossRef CAS.
- K. Hanke, V. Kupcik and O. Lindqvist, Acta Crystallogr., Sect. B: Struct. Sci., Cryst. Eng. Mater., 1973, 29, 963 CrossRef CAS.
- A. V. Ushakov and S. V. Streltsov, J. Phys.: Condens. Matter, 2009, 21, 305501 CrossRef CAS PubMed.
- M. Imada, A. Fujimori and Y. Tokura, Rev. Mod. Phys., 1998, 70, 1039 CrossRef CAS.
- M. Hase, I. Terasaki and K. Uchinokura, Phys. Rev. Lett., 1998, 70, 3651 CrossRef PubMed.
- H. F. Pen, L. H. Tjeng, E. Pellegrin, F. M. F. de Groot, G. A. Sawatzky, M. A. van Veenendaal and C. T. Chen, Phys. Rev. B: Condens. Matter Mater. Phys., 1997, 55, 15500 CrossRef CAS.
- N. Katayama, M. Uchida, D. Hashizume, S. Niitaka, J. Matsuno, D. Matsumura, Y. Nishihata, J. Mizuki, N. Takeshita, A. Gauzzi, M. Nohara and H. Takagi, Phys. Rev. Lett., 2009, 103, 146405 CrossRef CAS PubMed.
- H. Kato, M. Kato, K. Yoshimura and K. Kosuge, J. Phys. Soc. Jpn., 2001, 70, 1404 CrossRef CAS.
- G. Liu and J. E. Greedan, J. Solid State Chem., 1996, 122, 416 CrossRef CAS.
- C. A. Bridges, J. E. Greedan and H. Kleinke, J. Solid State Chem., 2004, 177, 4516 CrossRef CAS.
- A. Bridges and J. E. Greedan, J. Solid State Chem., 2004, 177, 1098 CrossRef.
- T. Kajita, T. Kanzaki, T. Suzuki, J. E. Kim, K. Kato, M. Takata and T. Katsufuji, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 81, 060405 CrossRef.
- J. Miyazaki, T. Sonehara, D. Akahoshi, H. Kuwahara, J. E. Kim, K. Kato, M. Takata and T. Katsufuji, Phys. Rev. B: Condens. Matter Mater. Phys., 2009, 79, 180410(R) CrossRef.
- M. Ikeda, T. Okuda, K. Kato, M. Takata and T. Katsufuji, Phys. Rev. B: Condens. Matter Mater. Phys., 2011, 83, 134417 CrossRef.
- J. Miyazaki, K. Matsudaira, Y. Shimizu, M. Itoh, Y. Nagamine, S. Mori, J. E. Kim, K. Kato, M. Takata and T. Katsufuji, Phys. Rev. Lett., 2010, 104, 207201 CrossRef CAS PubMed.
- S. V. Streltsov and D. I. Khomskii, Phys. Rev. B: Condens. Matter Mater. Phys., 2012, 86, 064429 CrossRef.
| This journal is © the Owner Societies 2016 |





