Solubility of dl-serine and dl-phenylalanine in aqueous mixtures of dimethyl sulfoxide and solvation thermodynamics

Abstract

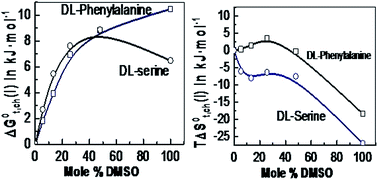
The standard free energies (ΔG⁰_t(i)) and entropies (ΔS⁰_t(i)) of transfer of DL-serine and DL-phenylalanine from water (1) to aqueous mixtures of dimethylsulfoxide (DMSO) (2) at 298.15 K are reported in the present study. The transfer energies have been determined from solubility measurements of the amino acids. The solubility is measured at different temperatures i.e. from 288.15 to 308.15 K by an ‘analytical gravimetric method’. The chemical parts of the free energies (ΔG⁰_t,ch(i)) and entropies (TΔS⁰_t,ch(i)) of transfer of the amino acids have been computed by subtracting the cavity effects and dipole–dipole interaction effects from the total transfer energies. The characteristics of the solvation thermodynamics of the amino acids in an aqua-organic solvent system are studied and discussed in the present manuscript.