Electrical conductivity equations derived with the rate process theory and free volume concept
Tian Hao
15905 Tanberry Dr., Chino Hills, CA 91709, USA. E-mail: haotian9@gmail.com
First published on 23rd April 2015
Abstract
Inspired by the Marcus theory of electron transfer, electrical conductivity equations without reference to any specific materials are derived on the basis of Eyring’s rate process theory and the free volume concept. The basic assumptions are that electrons are assumed to have a spherical physical shape with an imaginary effective radius inferred from the latest experimental evidence; electrons traveling from one equilibrium position to another obey Eyring’s rate process theory; and the traveling distance is governed by the free volume available for electrons to transport. The derived equations fit very well with experimental data, and seem to trend consistently with the currently observed experimental phenomena, too. The obtained equations predict that superconductivity happens only when electrons form certain structures of a small coordinate number like electron pairs, with the coordinate number equal to 1 at low temperatures, which is in line with the popular Cooper pairs concept in the BCS theory for superconductivity. The current work may provide new insights into the rich conductive behaviors at low temperatures.
I. Introduction
The electrical conductivities of various materials have been extensively addressed for many decades. The rich and distinctive conductive phenomena have led to many different theories and/or models for unveiling the underlying physical mechanisms. From pure crystalline materials like metals, semi-conductive metal oxides, amorphous solids, to superconductors, each class of materials gives remarkably different conductive behaviors, and thus researchers have to modify the theories continuously with more complications added in. What we don’t know seems to grow proportionally with what we have already learned so far, especially after the discoveries of the Kondo effect, Mott transition, Anderson transition, high temperature superconductors, ferropnictide superconductors, topological insulators, and so on. As scientific history has demonstrated repeatedly, we always start with simple and idealized models, enjoy the success of those achievements for some period of time, are surprised and puzzled with new experimental discoveries that cannot be explained with the current mechanisms/models, become excited with new theories that seem to work perfectly for everything we have observed, and then start a new cycle again. A good example is Einstein’s photoelectric theory, published in 1905. At that time, almost all physicists believed Maxwell’s wave theory of light and strongly opposed Einstein’s particle theory. Nevertheless, Einstein was confirmed to be correct many years later and we have no choice but to adopt the important wave-particle duality principle for elementary entities like photons and electrons.Electrons have particle and wave duality properties. An external electric field may “induce” electrons to show “particle” properties more often, especially when the temperatures are low at the same time. The electron was discovered by Thomson in 1897 due to its “particle” properties under an electric field.1 However, the “wave” rather than the “particle” properties of electrons have been paid a huge amount of attention and explored exhaustively during the last century; the “particle” properties of electrons seem not to have been weighed and investigated equally in a similar profound manner. The negligence of the “particle” properties of electrons may be due to the fact that the electron was identified as a particle before quantum mechanics was well established and the abundant wave properties were really overwhelming.
Electrons play a paramount role in the conductive behaviors of many materials. Although there are many conductivity problems that we don’t understand, the following facts seem widely accepted: (1) the conductivity results from the movements of charge carriers like electrons and ions; (2) the inter-electron interactions cannot be overlooked even in metallic materials; the resistivity comes from not only electron–lattice interactions, but also electron–electron interactions; (3) at low temperatures close to zero Kelvin, electrons may tend to condense, forming pairs as suggested in BCS theory2 and even crystal lattice structures as predicted by Wigner.3 Recent experimental evidence may confirm the existence of Cooper pairs,4 the body-centered cubic (bcc) structure in 3D,5 and triangular lattice structure in 2D,6 those unique electron packing structures. An improved precise measurement of the shape of electrons indicates that an electron is a perfect sphere7 and the electrical dipole moment of an electron determined very recently is indeed very small due to its perfect spherical shape.8 All the facts listed above may imply that electrons could be reasonably assumed to be negatively charged spherical particles with a “physical” radius. They may analogously behave like colloidal particles, as colloidal particles usually carry charges, interact repulsively with each other, and can form various crystal lattice structures, too.9–11
Similar to electron systems containing a huge amount of fast-moving electrons, colloidal suspensions contain thousands of thousands of particles that are moving non-stop due to Brownian motion. Both systems have complicated many-body problems, and the exact solution to the total interaction energy between particles/electrons is hard to estimate. In colloidal suspensions, we know that the inter-particle forces are important to the physical properties of the whole system. For example, the viscosities and the micro-structures of colloidal suspensions are strongly dependent on how strong the inter-particle forces are;12 if an external electric or a magnetic field were applied to a colloidal suspension, the particles would be polarized; the polarization will generate an additional amount of charge on the particle surfaces and dramatically enhance the inter-particle forces, leading to a viscosity increase of several orders of magnitude. Those suspensions are called electrorheological fluids13 or magnetorheological fluids,14 and have been explored extensively within the last several decades. The dramatic increases of the rheological properties are attributed to the crystallized bcc lattice structure formed by the polarized particles and induced by the applied external field. The conductive mechanism of electrorheological fluids obeys the variable range hopping model.15 The particle volume fractions, which scale how crowded the particles are in a suspension, can induce a very similar viscosity increase, due to a percolation type phase transition.16 Analogous to charged colloidal particles, the electrons, if they have a physical shape, can be easily polarized under either an electric or magnetic field and might easily form a crystallized structure, which may strongly control the conductivity or even superconductivity properties of materials.
Eyring’s rate process theory is based on quantum mechanics,17 and has been widely used in many fields since it was first introduced in 1935.18–20 In the article titled “Quantum statistical theory of rate process” published in 1972 by Eyring,17 many rate processes like diffusion, dielectric relaxation, electron transfer reactions, nonradioactive decay, resonance energy transfer, and many other thermally activated motions, were generically treated with a quantum mechanical approach, and the obtained rate constant equations have a very similar form to the original rate constant equations obtained from the absolute reaction rate theory.18 Although Eyring’s simple and elegant treatment approaches have been fiercely criticized from day one, the success in resolving many fundamental problems like chemical reaction rate, viscosity and diffusion, electrochemical processes, and biophysics, etc., has demonstrated again that the truth is always simple and his approaches are powerful and accurate, indeed. Especially, Eyring’s rate process theory has been confirmed to work not only for classical systems like molecular diffusions but also quantum mechanical electrons like electron transfer reactions.21 When dealing with electron transfer or transport from one species to another, which plays a significant role in all respiration, photosynthesis, and biochemical reactions, the Marcus theory of electron transfer clearly shows that the electron transport rate obeys essentially the same equation as described with Eyring’s rate process theory.22–24 The quantum mechanical treatments of electron transfer reach the same exponential term in the rate equation as predicted by the classical Marcus model,25 indicating that rate process theory can be effectively employed to describe electron transport, although the quantum tunneling effect is recognized to make functional contributions in certain biological processes.26–29 Marcus’ electron transfer theory further supports that the rate process theory holds for quantum mechanical electrons.
The free volume concept has been widely employed in chemistry and physics fields with great success in determining various equilibrium properties of both liquids30 and solids.31 The free volume is the space unoccupied by atoms in materials or particles in colloidal suspensions, providing freedom for atoms or particles to relax or re-orientate under stimulation. Successful examples include explaining the location of the melting transition,32 the glass transition temperatures of polymers,33 and the yield stress of electrorheological fluids under an external electric field,34 etc. Together with Eyring’s rate process theory, the free volume theory is successfully utilized to describe the viscosity of colloidal suspensions with and without an electric field.35 The idea is very simple: the free volume unoccupied by the particles in suspensions determines the free “walking” distance of particles, and the process of particles traveling from one equilibrium position to another is considered to obey Eyring’s rate process theory;36 the viscosity of the whole system is inversely proportional to the available free volume and the rate of particle motion. Instead of trying to figure out the exact multiple-particle interaction forces, the impact of the surrounding particles is grouped into the average “free volume” available to each particle. If many random, disordered, and multi-body systems can be well treated with Eyring’s rate process theory, especially for quantum mechanical electron transfers between different species under Marcus’ framework, the electrical conductivity that frequently involves electron transport could be treated with Eyring’s rate process theory, too. Such an attempt is made in this article with the aid of the free volume concept. The obtained conductivity equations seem able to describe many distinctive conductive behaviors, especially superconductivity, at low temperatures.
The electrical conductive behaviors of various materials, especially at low temperatures, are extremely rich and fascinating. There are many models or theories proposed so far to understand the puzzling conductive phenomena, and one of the most successful microscopic theories is the BCS theory for superconductivity proposed in 1957.2,37 However, as indicated in the literature,38,39 the BCS theory only can apply for the very special case of electron–phonon coupling, limiting it to the lowest temperature superconductors called conventional superconductors. The conductive behaviors of high temperature superconductors, the iron-based superconductors, and topological insulators/conductors, etc., classified as unconventional superconductors, need many new theoretical frameworks to explain, as each requires a different physical picture to describe. There is no single theory that can provide a reasonable explanation for all those fascinating conductive behaviors, which prompts us to utilize an unconventional approach for deriving conductive equations from a more generic and fundamental basis.
II. Theory
The conductivity, σ, can be expressed as:40
 | (1) |
 | (2) |
 is the specific velocity rate in any direction for the undisturbed system, kB is the Boltzmann constant, ΔG is the standard Gibbs free energy of the activation process, R is the gas constant, h is the Planck constant, and λ is the distance between the initial equilibrium position and the final position. In analogy, the number of electrons crossing the energy barrier in unit time in the reverse direction should be given as:
is the specific velocity rate in any direction for the undisturbed system, kB is the Boltzmann constant, ΔG is the standard Gibbs free energy of the activation process, R is the gas constant, h is the Planck constant, and λ is the distance between the initial equilibrium position and the final position. In analogy, the number of electrons crossing the energy barrier in unit time in the reverse direction should be given as:
 | (3) |
The net rate, the velocity of an electron in the direction of an applied electric field, is thus written as:
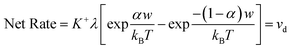 | (4) |
By the definition, the work needed to move an electron a distance λ under an electric field, E, may be expressed as:
| w = eλE | (5) |
Combining eqn (4) and (5), one may obtain the velocity of an electron:
 | (6) |
Now, one may need to determine λ, the distance that an electron can travel from one equilibrium position to the next. It should be related to how much free volume is available for a conduction electron in the system. According to standard solid state physics, the Fermi wavevector (radius) kF can be expressed as:40
 | (7) |
| Vc = Vu − VF | (8) |
 | (9) |
Thus eqn (8) can be expressed as:
 | (10) |
If the number of unit cells in a material is Nu, and the total volume of the material under consideration is Vm, the total free volume Vf is given as:
 | (11) |
 | (12) |
 | (13) |
Eqn (13) gives the free volume of each individual conduction electron. If the number of conduction electrons in the whole system is Nc, then the total free volume is:
 | (14) |
Note that eqn (11) should be identical to eqn (14), one thus can obtain the effective radius of an electron:
 | (15) |
Since an electron may move to both the left and right sides with the distance of the IPS, the equilibrium distance that an electron can travel, λ, may be expressed as:
 | (16) |
Now that we have the velocity of the conduction electrons as shown in eqn (6), and the distance that a conduction electron can travel as shown in eqn (16), one may easily obtain the conductivity equation based on eqn (1):
 | (17) |
As one may see, the conductivity has complicated relationships with the number of valence electrons in the unit cell, Nv; the number of conduction electrons in the whole system, Nc; the volume of the material under study, Vm; a parameter related to the structures that electrons may form, α; the standard Gibbs free energy, ΔG; and most importantly, the temperature, T. In thermodynamics, the Gibbs free energy, ΔG, has a relationship with the equilibrium constant, Keq:
−ΔG = RT![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) ln ln![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Keq Keq
| (18) |
 | (19) |
We may be able to use the equilibrium constant Keq to replace the Gibbs free energy term in eqn (17). As we mentioned earlier, in condensed systems, α is related to the coordinate number of an electron in the system, cn, with the simple relationship:
 | (20) |
 | (21) |
 | (22) |
 | (23) |
We will evaluate all these situations in a more generic manner in next section, for the purpose of extracting what are the generic trends/correlations between the conductivity and all the parameters in the equations.
III. Results
It would be insightful to compare the obtained equations with the experimental data for demonstrating the applicability of the derived equations in a quantitative manner. An iron-based superconductor is selected for the reason that this type of superconductors is still surprising even nine years after the initial discovery.47–50 The resistivity of α-FeSe against temperature shown in Figure 3 of ref. 51 is selected for comparison, only because this article is widely cited and readily available. The experimental data points and the regression computed with eqn (21) are shown in Fig. 2. Since the superconductivity is centered in this comparison, eqn (21) with α = 1 is used for regression. The best fit comes with the assumptions that α = 1, , and
, and  . Note that only the experimental data at the transition area is focused on for concisely demonstrating how eqn (21) would fit the experimental data; the inset in Fig. 2 shows the same data points at an even smaller scale. The purpose is to provide as clear a comparison as possible, and the y-axis range thus has been kept small. We have to say that eqn (21) fits the experimental data very well.
. Note that only the experimental data at the transition area is focused on for concisely demonstrating how eqn (21) would fit the experimental data; the inset in Fig. 2 shows the same data points at an even smaller scale. The purpose is to provide as clear a comparison as possible, and the y-axis range thus has been kept small. We have to say that eqn (21) fits the experimental data very well.
 | ||
Fig. 2 Conductivity of α-FeSe vs. temperature obtained experimentally and predicted with eqn (21). α = 1,  is assumed to be 104. The term is assumed to be 104. The term  is assumed to be 18. The experimental data points are taken from Figure 3 in ref. 51. is assumed to be 18. The experimental data points are taken from Figure 3 in ref. 51. | ||
For the purpose of an illustrative comparison, one may need to further simplify the conductivity equations listed in Section II based on some approximations. The low temperature regions will be focused on due to the rich and fascinating conductive behaviors. First, let’s concentrate on temperature dependence. The electron traveling distance, λ, should vary with temperature, on the basis of the Fermi–Dirac distribution function with the relationships Nv ∝ T3/2, NcNv ∝ T3, and λ ∝ T−1/2 approximately.52 Since the temperature range in the evaluation below is very narrow, it would be reasonable to assume that the electron traveling distance is independent of temperature for the purposes of simplicity and easy evaluation, assigned as about 100 nanometers as a starting point. The sole purpose is to present some ideas on how these equations work. So eEλ/kB is about 10 for a small electric field, 10 V mm−1. The term ekB/Eh is in the order of 10−13. The number of conduction electrons per unit volume is ∼1023, about one conduction electron per atom. The equilibrium constant  is usually a fairly large number, typically ∼104. So the term
is usually a fairly large number, typically ∼104. So the term  is ∼105. The term
is ∼105. The term  is ∼10. Under those approximations, the conductivity equation may be written as:
is ∼10. Under those approximations, the conductivity equation may be written as:
 | (24) |
 | ||
| Fig. 3 Conductivity vs. temperature at different lattice structures predicted with eqn (24) under an assumption that the electron traveling distance is 100 nm. The parameter A is ∼105 but A = 1 is assumed in the plot for simplification reasons. The coordinate number is 1 for pair structures, 4 for tetrahedron structures, and 8 for Wigner crystal (bcc) lattice structures. | ||
To further elucidate the trends, 3D plots are used to illustrate the conductivity vs. both temperature and α, first under the presumption that the electrons can still travel 100 nm from one equilibrium position to another, computed with eqn (24) and shown in Fig. 4; second under the presumption that the electrons may travel a smaller distance, 10 nm, shown in Fig. 5 and computed with the same equation, eqn (24). As clearly demonstrated in Fig. 4, a sharp conductivity increase that may represent the superconductivity transition occurs at low temperature regions and at α about 1; when temperatures are relatively high, the conductivity is flat and low, independent of the value of α. If the electron traveling distance is as low as 10 nm, as shown in Fig. 5, the conductivity only increases a little bit when the temperature is low and in addition α is about 1; the conductivity increment under such conditions is very low in comparison with that when the electron traveling distance is 100 nm. An intriguing thing happens when both temperature and α are low: the conductivity actually decreases sharply instead of increasing, which is opposite to the superconductivity transition. As one may know, the Mott transition results from the Coulombic repulsion among electrons and the degree of electron localization (band width).54,55 Low α means that each electron may have a high coordinate number and associate with many other electrons in certain crystalline bonding structures. Electrons can be localized if the “crystallinity energy” created by other associated electrons is strong enough. The conductivity thus should decrease dramatically, as electrons are trapped and unable to move freely. The phenomenon shown in Fig. 5 seems to correspond to the Mott transitions observed in many transition metal oxides at low temperatures.54
 | ||
| Fig. 4 Conductivity vs. both temperature and the structure related parameter α at an electron traveling distance of about 100 nm. The prediction is based on eqn (24). The parameter A is ∼105 but A = 1 is assumed in the plot for simplification reasons. | ||
 | ||
| Fig. 5 Conductivity vs. both temperature and the structure related parameter α at an electron traveling distance of about 10 nm. The prediction is based on eqn (24). The parameter A is ∼105 but A = 1 is assumed in the plot for simplification reasons. | ||
In the light of the physical size and quantum mechanical nature of electrons, 10 nm is still a huge distance for electrons to travel. However, the resulting conductivity already starts to sharply decrease as demonstrated in Fig. 5 at such a long traveling distance for electrons. What happens if the electron traveling distance further goes down to 1 nm? The illustrative graph computed with eqn (24) is shown in Fig. 6. In this case, the conductivity goes down substantially even when α = 1. As one may already realize, under such conditions electrons only form pair structures and the obstruction to electron movement from the “crystallinity energy” created by another associated electron should be pretty weak, but it is apparently strong enough to localize electrons. This seems to contradict what one may conclude from Fig. 5: the electron–electron interaction or the so-called electron confounding/confinement effect only becomes strong enough when each electron has a relatively large coordinate number, and then the formed “crystalline structures” may be able to trap or localize electrons, preventing electrons from transporting freely. In contrast, what is demonstrated in Fig. 6 is that everything seems to completely stop, probably not due to the strong localization resulting from the other coordinated electrons, but something else like strong interferences between paired electrons due to the quantum mechanical nature of electrons. Such a kind of localization seems to be very similar to the Anderson localization that has been explored extensively in disordered materials since it was first proposed in 1958.56–58 If what we postulated above is correct, one may come to the conclusion that the Mott localization may result from crystalline structures formed by electrons at low temperatures, corresponding to the low α region in both Fig. 5 and 6, while the Anderson localization may result from the strong interference between electrons at high α regions as shown in Fig. 6.
 | ||
| Fig. 6 Conductivity vs. both temperature and the structure related parameter α at an electron traveling distance of about 1 nm. The prediction is based on eqn (24). The parameter A is ∼105 but A = 1 is assumed in the plot for simplification reasons. | ||
Another intriguing phenomenon shown in Fig. 6 is that when electrons form pair structures at α = 1, the conductivity seems to slowly increase as the temperature approaches zero, go through a maximum, and then suddenly drop off; the conductivity peak could become much more pronounced if the electrons only can travel an even smaller distance, less than 1 nm. In low α regions, there is no such kind of conductivity bump. This phenomenon seems to correspond to the Kondo effect observed in metallic alloys doped with magnetic impurities59 and result from a strong spin–spin coupling between two electrons;60,61 once again, the interaction or interference resulted from the electron pair structures. Theoretically, the Anderson localization model at low temperatures is found to be equivalent to what has been discussed for the Kondo model,62,63 and the similarity seems to be demonstrated in Fig. 6 at low temperature, low traveling distance, and high α for electrons.
As demonstrated earlier, the electron pair structures usually favor superconductivity when electrons are assumed to travel a relatively long distance as shown in Fig. 4, while it could induce Kondo transition when electrons can only travel a very short distance as shown in Fig. 6. The Cooper pair that is the essence of the BCS theory of superconductivity and the spin–spin coupling that is the main culprit of the Kondo effect seem to contradict each other, but are harmoniously correlated in our current conductivity equations: the same electron pair structure but a different traveling distance could produce remarkable, even opposite, conductive behaviors. If this coincidence is true, it may imply that Kondo insulators could potentially be very good superconductors as well, as the electron pair structures are already formed and the only thing needed is to let the electrons travel a relatively long distance; on material surfaces, the electrons could potentially travel a much longer distance than the electrons in the interior. The reason may be pretty simple: there is only half the amount of atomic sites and thus half the amount of electrons available on material surfaces as in the interiors; the electrons thus have less chances of being hindered or localized and transport more freely on surfaces. Therefore, the Kondo insulators and superconductors could potentially co-exist together on a material, with superconductive surfaces and Kondo insulator interiors. The theoretically predicted and experimentally confirmed existence of topological insulators,64–66 where the surfaces show high conductivity properties and the interior is a Kondo insulator, may support what we have just postulated. The very recent evidence indicates that superconductivity does exist on the surfaces of topological insulators both theoretically67,68 and experimentally,69 further supporting this surprising postulation. In the interior of topological insulators, all paired electrons are strongly localized, probably due to the strong electron–phonon interactions; however, on the surfaces the electron–phonon interactions may become weak due to only half the amount of atomic sites being available to hinder the electron movements. If this physical picture is true, a material containing an element with a high electro-negativity or high ionization energy, like oxygen and fluorine, and an element with a low electro-negativity or low ionization energy like caesium, barium, and francium, etc., may form a good topological insulator. An element with a high electro-negativity will ensure that the electrons can be strongly pulled together to form pairs, while an element with a low electro-negativity will not hinder the electron movement too much, so the electrons can travel a relatively long distance, and the surfaces will show superconductivity. The ratio between these two elements could be critical, too, as we don’t want too many atoms with a high electro-negativity in the systems to restrict the electron movement. Additionally, since Kondo insulators are potentially good superconductors, any magnetic element like iron, nickel, and cobalt, that has unpaired electrons and can induce Kondo insulator behavior as a dopant, could be a very good superconductor candidate once it is bonded with other proper elements of low electro-negativity. The discovery of iron-based superconductors called ferropnictides,47,70–72which usually contain iron and other elements of a high electro-negativity or high ionization energy like oxygen and fluorine, supports the statements above derived from the conductivity equations.
The electron traveling distance seems to play a critical role in conductivity. It would be interesting to see how conductivity changes with the electron traveling distance, λ, especially when the electrons form pair structures, as this is the most favorable condition for superconductivity.
The conductivity predicted with eqn (24) is plotted against both temperature and electron traveling distance and shown in Fig. 7, under the assumption that electrons form pair structures. As expected, the conductivity increases with the increase of λ, and superconductivity appears when the temperature is low and the electrons can travel a long distance, such as 100 nm. Note that the superconductivity transition temperatures seem to shift to higher temperature regions when the electrons are able to travel a long distance, which may give some clues on what drives high temperature superconductivity. In other words, if the electrons can form pair structures and are able to travel a relatively long distance, superconductivity may appear at higher temperatures. This is an important prediction, clearly revealing why some superconductors have a high transition temperature and which physical parameter contributes to this. It may suggest that in searching for high temperature superconductors, attention should be placed on materials of high electron velocity.
 | ||
| Fig. 7 Conductivity vs. both temperature and electron traveling distance, λ, when electrons are paired with each other. The prediction is based on eqn (24). The parameter A is ∼105 but A = 1 is assumed in the plot for simplification reasons. | ||
For curiosity, what happens if a Wigner crystal forms and the electron traveling distance λ is large? The graph computed with eqn (24) is shown in Fig. 8. Again, the parameter A is assumed to be 1. Unsurprisingly, the conductivity becomes higher when λ increases, but becomes lower when the temperature goes down. A remarkable decrease occurs at higher λ and low temperature, probably implying that electron interaction or interference may become more pronounced at low temperature and higher traveling distance.
 | ||
| Fig. 8 Conductivity vs. both temperature and electron traveling distance, λ, when a Wigner crystal forms. The prediction is based on eqn (24). The parameter A is ∼105 but A = 1 is assumed in the plot for simplification reasons. | ||
The conductivity obviously has something to do with the volume of a material, as demonstrated in eqn (17). We will use the conductivity equation, eqn (21), to evaluate the relationship between the conductivity and the volume of the material, still under the assumption that the electrons form pair structures, as this condition will give more relevant information on superconductivity. The conductivity dependence on both the temperature and the volume of a material is shown in Fig. 9. The conductivity increases very slightly when the volume of the material becomes large in high temperature regions, and increases quite substantially at low temperature regions. High external pressure, which typically shrinks materials, may lower the conductivity, foreseeably lowering the superconductivity transition temperature too. However, under extremely high pressures, the electron density must increase to maintain the stability of chemical bonds; such an increase in electron density comes laterally from the region normal to the bond,73,74 providing more valence electrons and potentially bringing the superconductivity transition temperature higher. Those physical scenarios are qualitatively consistent with the experimental observations of how an external pressure changes the superconductivity transition temperatures:75,76 most times high pressures initially lower the superconductivity transition temperatures and then raise them as the pressures increase further. The consistency shown here further demonstrates again that our equations may truly grasp the underlying physical mechanisms of conductivities.
 | ||
Fig. 9 Conductivity vs. both the temperature and the volume of a material. The prediction is based on eqn (21). The term  is assumed to be 1, and the term is assumed to be 1, and the term  is assumed to be 0.2 in the plot for simplification reasons. is assumed to be 0.2 in the plot for simplification reasons. | ||
The conductivity may have a complicated relationship with the number of conduction electrons and valence electrons. The impact of the number of both the valence electrons in the unit cell and the conduction electrons in the whole system is shown in Fig. 10, computed with eqn (21). The number of conduction electrons has a deep impact on the conductivity as shown in Fig. 10a: the conductivity dramatically increases when the number of conduction electrons reaches a certain level, a phenomenon similar to the percolation transition observed in amorphous systems. In contrast, the valence electrons only show a huge impact on conductivity at low temperature regions very close to zero temperature in a gradual manner, but almost no impact at higher temperature regions, see Fig. 10b. This may imply that at very low temperatures the valence electrons may participate in the conduction as well.
 | ||
Fig. 10 (a) Conductivity vs. both the temperature and number of conduction electrons, Nc; the term  is assumed to be 1, and the term is assumed to be 1, and the term  is assumed to be 5; (b) conductivity vs. both the temperature and number of valence electrons, Nv. The term is assumed to be 5; (b) conductivity vs. both the temperature and number of valence electrons, Nv. The term  is assumed to be 1, the term is assumed to be 1, the term  is estimated to be 0.142. All predictions are based on eqn (21). is estimated to be 0.142. All predictions are based on eqn (21). | ||
The important question is of course how we can have a superconductor with a higher transition temperature and what implications we may obtain from the newly derived conductivity equations. From all figures shown in Fig. 3 to 10, one may easily conclude that a longer traveling distance for electrons, a larger number of conduction electrons, a larger number of valence electrons, and most importantly an electron pair structure, will probably favor superconductors with higher transition temperatures. From Fig. 9, one may conclude that by a decrease in the volume of a material, such as that from the bulk to a thin film, the transition temperature could be lowered, which has been confirmed experimentally.77–79 We may not be able to control the electron condensation structure at this moment, but we may be able to do it in the future with new technologies that can help us better manipulate and monitor. If we can increase the numbers of both conduction and valence electrons using an extremely thin epitaxy film deposited on a substrate, we may be able to increase the superconductivity transition temperatures, as confirmed experimentally.80,81 The negative impact from the material volume decrease is possibly well compensated by the dramatic conductivity increase from the greater amount of conduction and valence electrons.
IV. Discussion
The main conductivity equation derived in this article is shown in eqn (17), and other the equations shown in eqn (21)–(23) are just special cases of eqn (17). Eqn (24) is an approximated form of eqn (17), and is used for illustrative purposes for the readers to gain some ideas of how the obtained equations look. All the conductivity equations indicate that the temperature cannot be zero, otherwise the predicted conductivity will be divergent, which contradicts eqn (1). On the basis of the third law of thermodynamics, we can never reach the absolute zero temperature, and the predicted conductivity from all the equations can never be infinite. All the conductivity equations in this article thus automatically carry a condition of T ≠ 0. So in reality, there is no divergence, and the predicted conductivity may just be very high at low temperatures due to the low values of T. There is no contradiction to the basic thermodynamic law and to the experimental results.It is worth emphasizing again that electrons have particle and wave duality properties. Most researchers are paying a huge amount of attention to the “wave” properties rather than the “particle” properties, likely due to the rich and overwhelming wave properties of electrons, just discovered after the electrons were identified as particles initially. The extremely low electrical dipole moment determined recently58 and the perfect spherical shape of an electron precisely measured a few years ago7 may indicate that an electron may be reasonably assumed as a spherical particle with a physical shape. The implication that the extremely small electrical dipole moment would infer a “spherical” symmetrical shape of electrons is directly from the literature.7,8 Any question on the duality principle or the shape of the electron is out of the scope of this article. I simply borrow these results and concepts from other physicists’ work and cautiously use them as assumptions in this article. Even if these experimental results are later found untrue, the assumption that an electron has a physical shape with an effective radius is just an alternative convenient way for calculating the free volume. That is all.
Eyring’s rate process theory has been examined for over 80 years. It holds not only for classical molecular thermal systems but also quantum mechanical electrons as evidenced in the literature.17,37 Marcus’ electron transfer theory has a very similar mathematical form to Eyring’s, further supporting that Eyring’s rate process theory holds for quantum mechanical electrons and thus can be used to describe rich conductive behaviors mostly involving electron transport.
Free volume theory always employs some odd assumptions like holes in the liquids to explain multi-body phenomena, for example to explain the glass transitions in polymers and many other phenomena in amorphous solids. It is borrowed in this article to treat conductivity issues for estimating the free traveling distance of conduction electrons, due to its impressive power in dealing with multi-body phenomena. The final equations don’t contain anything related to the electron radius.
Our conductive equations present some kinds of hints about all novel conductive phenomena, such as topological insulators, Cooper pairs, Kondo effect, Mott transition, Anderson localization, and so on, as demonstrated from the illustrative graphs from Fig. 3–10. For example, from Fig. 3–6 the superconductivity transition is observed to only and preferably happen when the electrons form pair structures. There is no choice but to attribute this observation to the Cooper pair concept in BCS theory proposed more than a half century ago. The same things happen for the Anderson transition, Mott transition, Kondo effect, topological insulators, etc. I need to find some terms that can be easily understood by the condensed matter physics community and in the same time can match what is observed from the derived equations. Discussing these novel phenomena in this article is not merely because they are important in understanding conductivity behaviors. Instead, they are addressed solely because the illustrative plots from my equations suggest to me to do so; the connections between what has been already explored and discovered in conductivity areas and what can be deduced from our equations are clearly there, indeed.
In my personal discretion, two criteria may be employed to judge a theory right or wrong: (1) is the theory consistent with the currently observed experimental phenomena? (2) Does the theory predict something new and provide new insights to the puzzles we have now? As demonstrated in Section III, our equations basically provide hints about all the novel phenomena observed so far and can fit the experimental data very well, too. Regarding the predictions, I will present one example inferred from the plots shown in Fig. 3–6: a Kondo insulator and a superconductor may co-exist together, as both Kondo insulators and superconductors share the same physical origin—the electron pair structures. The only difference between those two is that in superconductors the electrons can travel a long distance such as 100 nm, in comparison with a very short distance such as 1 nm in Kondo insulators. If certain conditions allow, like the criteria hypothesized in Section III, the superconductivity presented on the surfaces and Kondo insulation preserved in the interior could be realized experimentally. This is a prediction directly coming from our equations. It is something even unexpected to the author; however, it appears to be true, with new evidence revealed very recently, both theoretically67,68 and experimentally.69 The study shown in ref. 69 claims that “by doping the topological insulator, bismuth selenide, with copper, it’s possible to make the topologically ordered electrons superconducting, dropping electrical resistance in the surface states all the way to zero”.
Conductivity, especially superconductivity, is a very complicated issue. It is unlikely that an equation that is very powerful but physically baseless would be obtained by coincidence . Our initial motivation was to derive a generic conductivity equation that holds for more materials. However, after the equations were obtained and plotted out at various conditions, I really was surprised but excited to see that the obtained equations are in line with many conductive phenomena at low temperatures. With many supportive experimental results and phenomena dug out from the literature, I became more confident in the assumptions used in the article.
Somebody may argue that it is the electronic structures of materials and the quantum transport nature of electrons that dominate the conductive properties of materials. Yes, I agree. Many researchers have followed this direction and developed many different theories to explain the rich and distinctive conductive behaviors. However, my great concern is that each class of materials needs a different theory to cover its conductive behaviors. A theory truly reflecting the underlying physical mechanisms would work not only for Kondo insulators but also for Mott insulators or topological insulators. A theory that only works for certain types of low temperature superconductors but doesn’t work for pnictides or high temperature superconductors may miss something important, as nature doesn’t typically operate in this manner. A theory that works for the conductive behaviors of all materials is anticipated. It is time to retreat back and think differently from more a fundamental basis, which is what I have attempted in this article.
The obtained equations directly suggest that a material containing an element with a high electro-negativity or high ionization energy and an element with a low electro-negativity or low ionization energy may form a good topological insulator: an element with a high electro-negativity could ensure that the electrons can be strongly pulled together to form pairs, while an element with a low electro-negativity may not hinder the electron movements too much, so the electrons can travel a relatively long distance and the surfaces will show conductivity, even superconductivity. Any magnetic element, like iron, nickel, and cobalt, that has unpaired electrons and can induce the Kondo effect as a dopant, could be a very good superconductor candidate once it is bonded with other proper elements of low electro-negativity. A typical example is pnictide superconductors. A material of high electron velocity containing chemical elements with high electro-negativity and unpaired electrons may show superconductivity at higher temperature regions. Again, the ratio between the chemical elements of high and low electro-negativities seems to be very important, as a high electro-negativity usually means a low electron velocity. Both the Kondo effect and superconductivity seem to share the same physical origin – the electrons form pair structures but travel different distances in those two cases. The electron pair structures are already formed in Kondo insulators and the only thing needed is to let the electrons travel a relatively long distance. The topological insulators are naturally expected to exist, with surfaces with conductive or even superconductive properties and interiors with insulating properties. The reason may be pretty simple: there are only half the amount of atomic sites and thus half the amount of electrons available on material surfaces as in interiors; the electrons thus have less chances of being hindered or localized and may transport more freely on surfaces. Therefore, the Kondo insulators and superconductors could potentially co-exist together on a material. Many of these predictions/suggestions need to be confirmed experimentally.
Although this article is little bit leaning to the low temperature conductive behaviors in Section III, the obtained equations don’t have such a limitation, as demonstrated in Section II. There is no assumption in the derivation procedures that may limit the applicability. Eqn (17) and (21)–(23), should work for both low and high temperature regions.
The particle properties of electrons are paid more attention in this article. However, this by no means indicates that the wave properties of electrons are unimportant. Many phenomena can still be explained with the wave properties of electrons, and both the particle and wave properties of electrons should be equally weighed.
V. Conclusion
Inspired by the Marcus theory of electron transfer, Eyring’s rate process theory and the free volume concept are used to come up with conductivity equations under three major assumptions: (1) electrons are assumed to have a spherical physical shape with an imaginary effective radius; (2) the electron traveling rate is assumed to obey Eyring’s rate process theory; (3) the available free volume for each electron is assumed to determine how far electrons may travel from one equilibrium position to the other.The obtained conductivity equations show that there is a complicated relationship between the conductivity and the electron condensation structure, the electron traveling distance, the numbers of both the conduction and the valence electrons, the volume of the material under investigation, and the most importantly, the temperature. When electrons form pair structures, the predicted conductivity increases with the decrease of temperature, and superconductivity occurs when temperature is below a critical point, while when electrons form tetrahedron or Wigner crystal lattice structures, the predicted conductivity decreases when the temperature decreases. Even when electrons form pair structures, the predicted conductivity could sharply decrease rather than increase, if the electrons can only travel a short distance, such as 1 nm. Additionally, at low temperature and high α regions, the predicted conductivity may go through a peak, which may correspond to the Kondo effect. The Anderson localization seems to have a lot of similarities to the Kondo effect, such as electron pair structures and low traveling distances at low temperatures.
As expected, the conductivity increases with the electron traveling distance, no matter which structures electrons may form: pair, tetrahedron, or Wigner crystal. It increases with the volume of the material and the numbers of both the conduction and the valence electrons. Anything that can change the volume of the material, such as pressure and thin film, which may change the number of conduction and valence electrons, could potentially change the conductivity as well. To obtain a superconductor with a higher transition temperature, it may be better for electrons to form pair structures and be capable of traveling a relatively long distance.
In summary, the derived conductivity equations can fit the experimental data well, provide hints about many novel low temperature conductive phenomena, and offer new insights into the mechanisms of conductivity at low temperatures.
Acknowledgements
The author sincerely appreciate many colleagues’ and reviewers’ feedback and comments for substantially improving the readability and rationality of this article.References
- J. J. Thomson, Cathode Rays, Electrician, 1987, 39, 104–109 Search PubMed.
- J. Bardeen, L. N. Cooper and J. R. Schrieffer, Microscopic Theory of Superconductivity, Phys. Rev., 1957, 106, 162–164 CrossRef CAS.
- E. Wigner, On the Interaction of Electrons in Metals, Phys. Rev., 1934, 46, 1002 CrossRef CAS.
- J. S. Van Dyke, F. Massee, M. P. Allan, J. C. S. Davis, C. Petrovic and D. K. Morr, Direct Evidence for a Magnetic f-electron-mediated Pairing Mechanism of Heavy-fermion Superconductivity, Proc. Natl. Acad. Sci. U. S. A., 2014, 111, 11663 CrossRef CAS PubMed.
- B. A. Poit, Z. Jiang, C. R. Dean, L. W. Engel, G. Gervais, L. N. Pfeiffer and K. W. West, Wigner Crystallization in Quasi-three-dimensional Electronic System, Nat. Phys., 2008, 4, 936 CrossRef PubMed.
- D. Zhang, X. Huang, W. Dietsche, K. von Klitzing and J. H. Smet, Signatures for Wigner Crystal Formation in the Chemical Potential of a Two-Dimensional Electron System, Phys. Rev. Lett., 2014, 113, 076804 CrossRef.
- J. J. Hudson, D. M. Kara, I. J. Smallman, B. E. Sauer, M. R. Tarbutt and E. A. Hinds, Improved Measurement of the Shape of the Electron, Nature, 2011, 473, 493–496 CrossRef CAS PubMed.
- J. Baron, W. C. Campbell, D. DeMille, J. M. Doyle, G. Gabrielse, Y. V. Gurevich, P. W. Hess, N. R. Hutzler, E. Kirilov, I. Kozyryev, B. R. O’Leary, C. D. Panda, M. F. Parsons, E. S. Petrik, B. Spaun, A. C. Vutha and A. D. West, The ACME Collaboration, Order of Magnitude Smaller Limit on the Electric Dipole Moment of the Electron, Science, 2014, 343, 269–272 CrossRef CAS PubMed.
- T. Chen, R. N. Zitter and R. Tao, Laser Diffraction Determination of the Crystalline Structure of Electrorheological Fluids, Phys. Rev. Lett., 1992, 68, 2555 CrossRef CAS.
- A. Yethiraj and A. van Blaaderen, A Colloidal Model System with an Interaction Tunable Hard Sphere to Soft and Dipolar, Nature, 2003, 421, 513 CrossRef CAS PubMed.
- Y. Kim, A. A. Shah and M. J. Solomon, Spatially and Temporally Reconfigurable Assembly of Colloidal Crystals, Nat. Commun., 2014, 5, 3676 CAS.
- W. B. Russel, D. A. Saville and W. R. Schowalter, Colloidal Dispersions (Cambridge Monographs on Mechanics), Cambridge University Press, March 27, 1992 Search PubMed.
- T. Hao, Electrorheological Fluids: The Non-aqueous Suspensions, Elsevier, December 19, 2005 Search PubMed.
- Magnetorheology: Advances and Applications, ed. N. M. Wereley, Royal Society of Chemistry, RSC Smart Materials, Cambridge, UK, 2014 Search PubMed.
- T. Hao and Y. Xu, Conductive Behaviors of Polymer-Based Electrorheological Fluid under Zero and Oscillatory Mechanical Fields, J. Colloid Interface Sci., 1996, 181, 581 CrossRef CAS.
- T. Hao, Y. Chen, Z. Xu, Y. Xu and Y. Huang, Percolation Transition in Electrorheological Fluids, Chin. J. Polym. Sci., 1994, 12, 97 CAS.
- S. H. Lin and H. Eyring, Quantum Statistical Theory of Rate Process, Proc. Natl. Acad. Sci. U. S. A., 1972, 69, 3191–3194 Search PubMed.
- H. Eyring, The Activated Complex in Chemical Reactions, J. Chem. Phys., 1935, 3, 107 CrossRef CAS PubMed.
- P. Hanggi, P. Talkner and M. Borkvec, Reaction-rate Theory: Fifty Years after Kramers, Rev. Mod. Phys., 1990, 62, 251 CrossRef.
- K. J. Laidler and M. C. Klng, The Development of Transition-State Theory, J. Phys. Chem., 1983, 87, 2657–2664 CrossRef CAS.
- P. P. Schmidt, Theory of Electron Transfer Reactions in Simple Fluids, J. Chem. Phys., 1972, 56, 2775–2787 CrossRef CAS PubMed.
- R. A. Marcus, On theTheory of Oxidation-Reduction Reactions Involving Electron Transfer. I, J. Chem. Phys., 1956, 24, 966–978 CrossRef CAS PubMed.
- R. A. Marcus, On the Theory of Oxidation–Reduction Reactions Involving Electron Transfer. II: Applications to Data on the Rates of Isotopic Exchange Reactions, J. Chem. Phys., 1957, 26, 867–871 CrossRef CAS PubMed.
- D. Matyushov, Electron Transfer Reactions: Theory, Encyclopedia of Inorganic and Bioinorganic Chemistry, John Wiley and Sons, Inc., 2011 Search PubMed.
- P. Ceroni and V. Balzani, Photoinduced Energy and Electron Transfer Processes, The Exploration of Supramolecular Systems and Nanostructures by Photochemical Techniques, Lecture Notes in Chemistry 78, ed. P. Ceroni, Springer Science+Business Media B.V, 2012 Search PubMed.
- J. S. Bader, R. A. Kuharski and D. Chandler, Role of nuclear tunneling in aqueous ferrousferric electron transfer, J. Chem. Phys., 1990, 93, 230–236 CrossRef CAS PubMed.
- X. Song and R. A. Marcus, Quantum correction for electron transfer rates. Comparison of polarizable versus nonpolarizable descriptions of solvent, J. Chem. Phys., 1993, 99, 7768–7773 CrossRef CAS PubMed.
- R. Dorner, J. Goold, L. Heaney, T. Farrow and V. Vedral, Effects of quantum coherence in metalloprotein electron transfer, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2012, 86, 031922 CrossRef.
- A. de la Lande, N. S. Babcock, J. Řezáč, B. Lvy, B. C. Sanders and D. R. Salahub, Quantum effects in biological electron transfer, Phys. Chem. Chem. Phys., 2012, 14, 5902–5918 RSC.
- J. A. Barker, Lattice Theories of the Liquid State, The International Encyclopedia of Physical Chemistry and Chemical Physics, Pergamon Press, Oxford, 1963, vol. 1 Search PubMed.
- F. H. Stillinger Jr, Z. W. Salsburg and R. L. Kornegay, Rigid Disks at High Density, J. Chem. Phys., 1965, 43, 932–943 CrossRef PubMed.
- H. Kawamura, A simple theory of hard disk transition, Prog. Theor. Phys., 1979, 61, 1584–1596 CrossRef CAS.
- T. G. Fox and P. J. Flory, Second-order transition temperatures and related properties of polystyrene, J. Appl. Phys., 1950, 21, 581–591 CrossRef CAS PubMed.
- T. Hao, A. Kawai and F. Ikazaki, The yield stress equation for the electrorheological fluids, Langmuir, 2000, 16, 3058–3066 CrossRef CAS.
- T. Hao, Viscosities of Liquids, Colloidal Suspensions, and Polymeric Systems under Zero or Non-zero Electric Field, Adv. Colloid Interface Sci., 2008, 142, 1–19 CrossRef CAS PubMed.
- S. Glasstone, K. Laidler and H. Eyring, The Theory of Rate Processes, McGraw Hill, 1st edn, 1941 Search PubMed.
- J. Schmalian, Failed theories of superconductivity, in Bardeen Cooper and Schrieffer: 50 YEARS, ed. L. N. Cooper and D. Feldman, World Scientific Publishing Company, 1st edn, June 1, 2010 Search PubMed.
- J. E. Hirsch, BCS theory of superconductivity: it is time to question its validity, Phys. Scr., 2009, 80, 035702 CrossRef.
- J. P. Hague, Failure of conventional superconductivity theory for optical-phonon mediated d-wave pairing, J. Phys.: Conf. Ser., 2007, 92, 012119 CrossRef.
- C. Kittel, Introduction to Solid State Physics, Wiley, November 11, 2004 Search PubMed.
- F. H. Ree, T. Ree and H. Eyring, The Relaxation Theory of Transport Problems in Condensed Systems, Ind. Eng. Chem., 1958, 50, 1036 CrossRef CAS.
- T. Hao and R. E. Riman, Calculation of Interparticle Spacing in Colloidal Systems, J. Colloid Interface Sci., 2006, 297, 374–377 CrossRef CAS PubMed.
- S. Kuwabara, The Forces Experienced by Randomly Distributed Parallel Circular Cylinders or Spheres in a Viscous Flow at Small Reynolds Numbers, J. Phys. Soc. Jpn., 1959, 14, 527–532 CrossRef.
- M. W. Kozak and E. J. Davis, Electrokinetic Phenomena in Fibrous Porous Media, J. Colloid Interface Sci., 1986, 112, 403–411 CrossRef CAS.
- S. Levine and G. H. Neale, The Prediction of Electrokinetic Phenomena within Multiparticle Systems. I. Electrophoresis and Electroosmosis, J. Colloid Interface Sci., 1974, 47, 520–529 CrossRef.
- H. Ohshima, Dynamic Electrophoretic Mobility of Spherical Colloidal Particles in Concentrated Suspensions, J. Colloid Interface Sci., 1997, 195, 137–148 CrossRef CAS.
- Y. Kamihara, H. Hiramatsu, M. Hirano, R. Kawamura, H. Yanagi, T. Kamiya and H. Hosono, Iron-Based Layered Superconductor: LaOFeP, J. Am. Chem. Soc., 2006, 128(31), 10012–10013 CrossRef CAS PubMed.
- Y. Kamihara, T. Watanabe, M. Hirano and H. Hosono, Iron-Based Layered Superconductor La[O1−xFx]FeAs (x = 0.05–0.12) with Tc = 26 K, J. Am. Chem. Soc., 2008, 130, 3296–3297 CrossRef CAS PubMed.
- P. M. Aswathy, J. B. Anooja, P. M. Sarun and U. Syamaprasad, An overview on iron based superconductors, Supercond. Sci. Technol., 2010, 23, 073001 CrossRef.
- X. Chen, P. Dai, D. Feng, T. Xiang and F. Zhang, Iron-based high transition temperature superconductors, Natl. Sci. Rev., 2014, 1, 371–395 Search PubMed.
- F. Hsu, J. Luo, K. Yeh, T. Chen, T. Huang, P. M. Wu, Y. Lee, Y. Huang, Y. Chu, D. Yan and M. Wu, Superconductivity in the PbO-type structure α-FeSe, Proc. Natl. Acad. Sci. U. S. A., 2008, 105, 14262–14264 CrossRef CAS PubMed.
- J. Colinge, and C. A. Colinge, Physics of Semiconductor Devices, Kluwer Academic Publishers, 2002 Search PubMed.
- D. Kondepudi, Introduction to Modern Thermodynamics, Wiley, Chichester, 2008 Search PubMed.
- N. F. Mott, Metal-insulator Transitions, Taylor and Francis, 2nd edn, 1990 Search PubMed.
- M. Imada, A. Fujimori and Y. Tokura, Metal-insulator transitions, Rev. Mod. Phys., 1998, 70, 1039 CrossRef CAS.
- P. W. Anderson, Absence of Diffusion in Certain Random Lattices, Phys. Rev., 1958, 109(5), 14921505 CrossRef.
- A. Lagendijk, B. van Tiggelen and D. S. Wiersma, Fifty Years of Anderson Localization, Phys. Today, 2009, 62(8), 24–29 CrossRef CAS PubMed.
- J. Billy, V. Josse, Z. Zuo, A. Bernard, B. Hambrecht, P. Lugan, D. Clment, L. Sanchez-Palencia, P. Bouyer and A. Aspect, Direct Observation of Anderson Localization of Matter Waves in a Controlled Disorder, Nature, 2008, 453, 891–894 CrossRef CAS PubMed.
- J. Kondo, Resistance Minimum in Dilute Magnetic Alloys, Prog. Theor. Phys., 1964, 32(1), 37–49 CrossRef CAS.
- A. C. Hewson, The Kondo Problem to Heavy Fermions, Cambridge University Press, Cambridge, 1997 Search PubMed.
- Y. Zhang, S. Kahle, T. Herden, C. Stroh, M. Mayor, U. Schlickum, M. Ternes, P. Wahl and K. Kern, Temperature and Magnetic Field Dependence of a Kondo System in the Weak Coupling Regime, Nat. Commun., 2013, 4, 2110 Search PubMed.
- J. R. Schrieffer and P. A. Wolff, Relation between the Anderson and Kondo Hamiltonians, Phys. Rev., 1966, 149, 491 CrossRef CAS.
- B. Mhlschlegel, Relation between the Anderson and Kondo Hamiltonians for the Case of Degenerate Impurity Orbitals, Z. Phys., 1968, 208, 94–103 CrossRef.
- C. L. Kane and E. J. Mele, Z2 Topological Order and the Quantum Spin Hall Effect, Phys. Rev. Lett., 2005, 95, 146802 CrossRef CAS.
- M. Knig, S. Wiedmann, C. Brne, A. Roth, H. Buhmann, L. W. Molenkamp, X. Qi and S. Zhang, Quantum Spin Hall Insulator State in HgTe Quantum Wells, Science, 2007, 318, 766–770 CrossRef PubMed.
- J. E. Moore, The Birth of Topological Insulators, Nature, 2010, 464, 194–198 CrossRef CAS PubMed.
- J. She and A. V. Balatsky, Negative-U Superconductivity on the Surface of Topological Insulators, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 90, 104517 CrossRef.
- N. Hao and J. Hu, Topological Phases in the Single-Layer FeSe, Phys. Rev. X, 2014, 4, 031053 Search PubMed.
- http://www-als.lbl.gov/index.php/contact/484-superconducting-topological-insulators.html, their studies showed that by doping the topological insulator, bismuth selenide, with copper, it’s possible to make the topologically ordered electrons superconducting, dropping electrical resistance in the surface states all the way to zero.
- J. C. Davis and P. J. Hirschfeld, Iron-Based Superconductors: Enigmatic Nematic, Nat. Phys., 2014, 10, 184–185 CrossRef CAS PubMed.
- E. P. Rosenthal, E. F. Andrade, C. J. Arguello, R. M. Fernandes, L. Y. Xing, X. C. Wang, C. Q. Jin, A. J. Millis and A. N. Pasupathy, Visualization of Electron Nematicity and Unidirectional Antiferroic Fluctuations at High Temperatures in NaFeAs, Nat. Phys., 2014, 10, 225–232 CrossRef CAS PubMed.
- Y. Luo, L. Pourovskii, S. E. Rowley, Y. Li, C. Feng, A. Georges, J. Dai, G. Cao, Z. Xu, Q. Si and N. P. Ong, Heavy Fermion Quantum Criticality and Destruction of the Kondo Effect in a Nickel Oxypnictide, Nat. Mater., 2014, 13, 777 CrossRef CAS PubMed.
- G. V. Gibbs, J. W. Downs and M. B. Boisen, The Elusive SiO bond, in Silica: Physical Behavior, Geochemistry, and Materials Applications, ed. P. J. Heaney, C. T. Prewiit and G. V. Gibbs, Rev. Mineral., 1994, vol. 29, pp. 331–368 Search PubMed.
- C. T. Prewitt and R. T. Downs, High-Pressure Crystal Chemistry, Reviews in Mineralogy, 37, Ultrahigh-Pressure Mineralogy: Physics and Chemistry of the Earth’s Deep Interior, ed. R. J. Hemley, Mineralogical Society of America, Washington, DC, 1998, pp. 283–317 Search PubMed.
- B. Lorenz and C. W. Chu, High Pressure Effects on Superconductivity, Front. Supercond. Mater., 2005, 459–497 CAS.
- S. Gabni, I. Takov, G. Prist, E. Gao, K. Flachbart, T. Mori, D. Braithwaite, M. Mek, K. V. Kamenev, M. Hanfland and P. Samuely, High-pressure Effect on the Superconductivity of YB6, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 90, 045136 CrossRef.
- D. G. Naugle, R. E. Glover and W. Moormann, Thickness Dependence of the Transition Temperature of Superconducting Films, Physica, 1971, 55, 250–255 CrossRef CAS.
- O. Crauste, C. A. Marrache-Kikuchi, L. Berge, D. Stanescu and L. Dumoulin, Thickness Dependence of the Superconductivity in Thin Disordered NbSi Films, J. Phys.: Conf. Ser., 2009, 150, 042019 CrossRef.
- Z.-L. Guan, Y.-X. Ning, C.-L. Song, J. Wang, J.-F. Jia, X. Chen, Q.-K. Xue and X. Ma, Sample-size Dependence of the Superconducting Transition of Ribbon-shaped Pb Nanocrystals Studied by Scanning Tunneling Spectroscopy, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 81, 054516 CrossRef.
- A. T. Bollinger, G. Dubuis, J. Yoon, D. Pavuna, J. Misewich and I. Boovi, Superconductor–insulator Transition in La2−xSrxCuO4 at the Pair Quantum Resistance, Nature, 2011, 472, 458–460 CrossRef CAS PubMed.
- Y. Ivry, C.-S. Kim, A. E. Dane, D. De Fazio, A. McCaughan, K. A. Sunter, Q. Zhao and K. K. Berggren, Universal Scaling of the Critical Temperature for Thin Films near the Superconducting-to-insulating Transition, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 90, 214515 CrossRef.
| This journal is © The Royal Society of Chemistry 2015 |

