Secondary channels in the thermal decomposition of monomethylhydrazine (CH3NHNH2)†
Peng Zhang*a,
Stephen J. Klippenstein*b,
Lawrence B. Hardingb,
Hongyan Sunc and
Chung K. Lawc
aDepartment of Mechanical Engineering, The Hong Kong Polytechnic University, Hong Kong. E-mail: pengzhang.zhang@polyu.edu.hk
bChemical Sciences and Engineering Division, Argonne National Laboratory, Argonne, Illinois 60439, USA. E-mail: sjk@anl.gov
cDepartment of Mechanical and Aerospace Engineering, Princeton University, Princeton, New Jersey 08544, USA
First published on 12th November 2014
Abstract
Mass spectrometric observations in a very low pressure pyrolysis study (Golden et al., Int. J. Chem. Kinet., 1972, 4, 433–448) of the decomposition of the prototypical rocket fuel monomethylhydrazine (MMH) indicated a dominant role for the molecular channels producing NH3 and H2 and their coproducts. In contrast, a recent ab initio transition state theory based master equation theoretical study (Zhang et al., Proc. Combust. Inst., 2011, 33, 425–432) indicated that simple N–N and C–N bond fissions dominate the kinetics. The possible role of molecular decomposition channels in MMH is explored further through additional investigations of the potential energy surface. These investigations consider the role of triplet channels, of roaming radical channels, and of some previously unexplored pathways for molecular decomposition. New ab initio transition state theory based master equation calculations provide revised predictions for the temperature and pressure dependence of the MMH decomposition kinetics that are in excellent agreement with recent shock tube measurements (Li et al., Comb. Flame, 2014, 161, 16–22). These calculations continue to suggest only a very limited contribution from the molecular elimination channels. A roaming pathway is suggested to provide the dominant route for direct formation of ammonia. The possible role of secondary abstraction reactions in the very-low-pressure pyrolysis experiments is briefly discussed.
1. Introduction
Monomethylhydrazine (MMH) is a commonly used hypergolic propellant in rocket engines.1,2 Since MMH tends to exothermically decompose upon contact with a hot surface or an oxidizer, its thermal decomposition is an important consideration related to fuel stability and storability. Moreover, the decomposition of MMH is a necessary component of the detailed kinetic mechanism for MMH oxidization, which is required for the numerical simulation of rocket engine combustion.A reaction mechanism for the thermal decomposition of MMH was developed by Sun and Law3,4 and then used to model the overall thermal decomposition rates of Eberstein and Glassman5 at 750–1500 K and 1 atm. The mechanism was also extended to model the shock tube experimental data for MMH pyrolysis.4,6,7 Based on these studies, the N–N and C–N bond fission reactions,
| CH3NHNH2 → NH2 + CH3NH | (1) |
| CH3NHNH2 → CH3 + NHNH2 | (2) |
Subsequently, we provided a detailed theoretical kinetics analysis for the reactions (1) and (2), and the related reverse barrierless radical–radical association reactions,8
| NH2 + CH3NH → CH3NHNH2 | (3) |
| CH3 + NHNH2 → CH3NHNH2 | (4) |
In this analysis, the capture rates for (3) and (4) were evaluated with variable reaction coordinate transition state theory (VRC-TST)9–11 employing interaction energies determined directly from multireference electronic structure calculations. Predictions for the pressure dependence and product branching in the dissociation of CH3NHNH2 were then obtained by solving the master equation while incorporating the transition state information from the VRC-TST calculations.
These theoretical predictions, which employed an expression for the collisional energy transfer parameter 〈ΔEdown〉 of 200(T/300)0.85 cm−1, agreed well with the experimental data of Kerr et al.12 A recent shock tube study of Li et al.,7 which measured NH2 time profiles, found that the theoretical rates of Zhang et al.8 are in reasonable agreement with their experimental data at various temperatures and pressures, but that a reduction of the rate of (1) by 40% (perhaps due to an overestimate of 〈ΔEdown〉) would effect a closer match. However, there are significant discrepancies between the theoretical results and the experimental data of Eberstein and Glassman5 for the overall decomposition rates, which suggests that either additional decomposition channels or secondary reactions may play a role. Furthermore, the very low pressure pyrolysis study of Golden et al.13 suggests a dominant role for formation of NH3 and H2.
The prior study of Sun and Law3 provided a fairly complete CBS-QB3 based study of the potential energy surface (PES) for the decomposition of MMH. However, there are a few other possible channels that may have an effect on the overall decomposition rate of MMH and/or on the formation of products such as H2, NH3, and CH4. Of particular interest are channels producing triplet products via intersystem crossing from the ground singlet state. Also, roaming radical channels in the N–N and C–N bond fissions would produce NH3 and CH4, respectively. Meanwhile, roaming radical channels in C–H and N–H bond fissions would yield H2.
The motivation of the present study is to first explore such new reaction channels on the PES for MMH decomposition with ab initio electronic structure theory and then, as appropriate, predict the rate coefficients for these channels with transition state theory. These calculations allow us to further consider the interpretation of the available experimental data, such as those of Golden et al.13
In the following text, the theoretical methods employed in the present electronic structure, transition state theory, and master equation calculations will be summarized in Section 2. This summary will be followed by presentation and discussion of the results in Section 3.
2. Theoretical methods
2.1 Potential energy surface
The geometric structures, vibrational frequencies, and zero-point energy (ZPE) for the primary stationary points on the PES were obtained via density functional theory, employing the Becke three-parameter functional and the Lee–Yang–Parr correlation functional (B3LYP) with the 6-311++G(d,p) basis set.14,15 The corresponding intrinsic reaction paths for the transition states were also examined at the B3LYP/6-311++G(d,p) level. Higher level stationary point energies were obtained from restricted QCISD(T) (quadratic configuration interaction with singles doubles and perturbative inclusion of triples) calculations. These restricted QCISD(T) calculations employed the correlation-consistent, polarized-valence, triple-ζ (cc-pVTZ) and quadruple-ζ (cc-pVQZ) basis sets of Dunning16,17 and were extrapolated to the complete basis set limit (CBS)18 via the expression E[QCISD(T)/∞] = E[QCISD(T)/cc-pVQZ] + {E[QCISD(T)/cc-pVQZ] − E[QCISD(T)/cc-pVTZ]} × 0.6938. The B3LYP/6-311++G(d,p) vibrational frequencies were employed to determine the zero-point energy corrections. This QCISD(T)/CBS//B3LYP/6-311++G(d,p) method is also employed in the analysis of the torsional minima and barriers for all the stationary points.The spin-forbidden reaction paths to CH3NH2 + 3NH and NH3 + 3NCH3, have enthalpies of reaction that are much lower than that of (1), but were not considered in previous studies of the PES. One primary focus of the present work involves the determination of the kinetic relevance of these two spin-forbidden pathways. To form either of these sets of spin forbidden products, a hydrogen atom must first migrate between the secondary amine group and the terminal ammonia group to form either CH3NH2NH or CH3NNH3; these two MMH isomers have not been reported in previous studies. In each case, the products may then be formed by lengthening of the N–N bond until an intersystem crossing (ISC) with the triplet state is reached,
 | (5) |
 | (6) |
The minimum point on the crossing seam (MSX) between the singlet and triplet PESs for (5) and (6) plays a role analogous to the transition state in determining the contribution to the dissociation kinetics from these channels.19,20 Several methods have been proposed for determining the MSX.21–24 The MOLPRO quantum chemistry program package,25 which was the primary resource for the electronic structure aspects of the present work, allows for the determination of the MSX with the complete active space self-consistent field (CASSCF) method.
Although the CASSCF method provides a good description of the multi-reference character of wave functions, it does not provide highly accurate energies due to its inadequate treatment of dynamical electron correlation. Thus, we have also evaluated the singlet and triplet energies at the CASSCF MSX geometries with the QCISD(T) method, which is expected to provide more accurate energies. Furthermore, on the singlet surface, lengthening of the N–N bond correlates with the formation of a singlet diradical, which often is not well described with single reference based methods such as the QCISD(T) method. Consequently, we have also used the complete active space with second-order perturbation theory (CASPT2) and multi-reference configuration interaction (MRCI) methods to explore the crossings. In these multi-reference calculations we have minimized the square of the singlet triplet energy difference, starting from geometries near the CASSCF MSX. Although these minimizations do not provide minimum points on the seam of crossing, they do provide geometries that are on the seam of crossing for the higher level method, and which are near to the CASSCF MSX geometry. As such, they likely provide a reasonable estimate of the true MSX geometry and energy. A two-electron, two-orbital, (2e, 2o) complete active space, which consists of the pair of radical electrons on the triplet NH or NCH3 radicals, was used in these calculations as the reference wave function.
For the N–N bond fission reaction there is also a roaming radical mediated pathway that leads to the production of CH2NH + NH3. The full roaming mediated reaction involves the sequence CH3NHNH2 → CH3NH⋯NH2 → CH2NH + NH3, where CH3NH⋯NH2 denotes a long-range hydrogen bonded complex separating MMH from CH2NH + NH3. The rovibrational and energetic properties of this roaming pathway were studied with the CASPT2 approach employing a (2e, 2o) active space. The active space for these calculations consists of the radical orbitals of NH2 and of CH3NH. The cc-pVQZ basis set was used in exploring the roaming saddle point, the aug-cc-pVTZ basis set was used in predicting the properties for the other stationary points, the cc-pVTZ basis set was employed in calculations along an NH distinguished reaction coordinate for the abstraction portion of the pathway, and the aug-cc-pVDZ basis set was used in the illustrative global mapping of the long-range interactions between CH2NH and NH3.
In the present study, all the density functional theory calculations were performed with the Gaussian program package,26 while the MOLPRO program package27 was used to perform all of the CASSCF, CASPT2, MRCI and QCISD(T) calculations.
2.2 High pressure kinetics
For the reaction channels with a large energy barrier, and thus a well-defined transition state, the high-pressure rate coefficients were obtained from transition state theory (TST) employing rigid-rotor harmonic-oscillator (RRHO) assumptions for all degrees of freedom except the torsional ones. Tunneling corrections based on asymmetric Eckart potentials were included. Hindered rotor corrections for the torsional modes were obtained from one-dimensional fits to the torsional potentials employing Pitzer–Gwinn like approximations and the I(2,3) moments of inertia.28 The fits to the torsional potentials were designed to reproduce the B3LYP/6-311++G(d,p) torsional frequency at the minimum as well as the QCISD(T)/CBS//B3LYP/6-311++G(d,p) torsional barrier heights and secondary minima, if they exist. For (1) and (2), the VRC-TST analysis from Zhang et al.8 was employed.Theoretical frameworks for predicting the intersystem crossing (ISC) rate have been presented.29,30 However, these methods are somewhat involved and so it is worthwhile to consider alternatives. For example, experimental observations can sometimes be used to derive ISC rates from empirical fits to the data,31,32 or alternatively they can demonstrate that the ISC is insignificant.33 It will be demonstrated in the next section that the MSXs for (5) and (6) have higher energy thresholds than the transition state for (1) and hence are expected to have a negligible influence on the decomposition rate of MMH. They are also expected to have a limited role in product formation. As a result, (5) and (6) were not considered in the present rate calculations.
2.3 Pressure dependent kinetics
As will be seen shortly, the PES for MMH decomposition consists of multiple, interconnected potential wells and multiple product channels. However, the particular form of this PES (i.e., the dominance of the decomposition from the CH3NHNH2 well to bimolecular products, even in the high pressure limit) allows for simplification to a single-well, multiple-channel one. The pressure dependent rate coefficients for this single well model were determined by solving the master equation. The relevant theory will not be discussed here since it has been described in detail in the literature34,35 and implemented in the VARIFLEX code.36Following our previous study,8 we employ a Lennard-Jones collision model and the energy transfer probability was approximated with a single-exponential-down model and an average downward energy transferred parameter, 〈ΔEdown〉, that is proportional to T0.85. The approximation that 〈ΔEdown〉 increases roughly linearly with temperature has been validated in related studies such as that for the dissociation of C2H3 and C2H5 (ref. 37) with the light bath gas molecules He, Ar, and N2. The Lennard-Jones parameters for the MMH molecule are σ = 4.4 Å and ε = 340 cm−1, which are based on the empirical method proposed by Wang and Frenklach.38 For N2, which was employed as the primary bath gas, we used σ = 3.62 Å and ε = 68 cm−1 (ref. 39) and the value of 〈ΔEdown〉 at room temperature was taken to be 120 cm−1. In order to compare with the recent experimental data of Li et al.,7 Ar was also used as the bath gas with σ = 3.47 Å, ε = 79 cm−1 and a slightly larger room temperature 〈ΔEdown〉 of 130 cm−1.39 We also performed calculations for toluene as a bath gas since it is in high concentration in the toluene-carrier flow system of Kerr et al.12 For toluene we employed Lennard-Jones parameters of σ = 4.7 Å and ε = 150 cm−1. Due to the larger size of toluene, with many vibrational modes, one expects greater 〈ΔEdown〉 values for its collisions with MMH and for it we explore the effect of varying the room temperature 〈ΔEdown〉 value from 300 to 600 cm−1.
2.4 Roaming radical kinetics
Kinetic predictions for the roaming radical pathway were obtained by treating the long-range CH3NH⋯NH2 complex as a second well with exit channels to (i) the abstraction products, (ii) CH3NH + NH2 and (iii) back to CH3NHNH2. A master equation based treatment of the decomposition then yields a predicted branching between formation of either CH3NH + NH2 or CH2NH + NH3. The requisite microcanonical rates for the abstraction channel are obtained from an RRHO based variational TST treatment employing a distinguished NH coordinate. The roaming saddle point correlates with the starting point of this variational pathway. The dissociation part of the analysis is performed with VRC-TST employing CASPT2(2e, 2o)/aug-cc-pVDZ sampling and a one-dimensional correction to the CASPT2(2e, 2o)/aug-cc-pVTZ level.3. Results and discussion
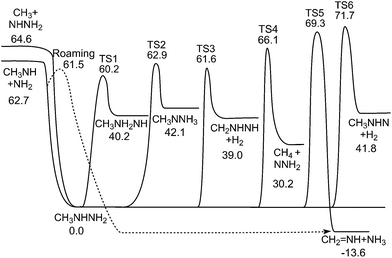
3.1 Potential energy surface for CH3NHNH2 decomposition
The PES for MMH dissociation calculated at the QCISD(T)/CBS//B3LYP/6-311++G(d,p) level is shown in Fig. 1. The optimized geometries for the stationary points on the PES and the corresponding frequencies and rotational constants are listed in the ESI.† Note that only the reaction channels that are directly connected to MMH and that also have relative energies less than 80 kcal mol−1 are included. Compared to the CBS-QB3 energies used in the previous study,3 the QCISD(T)/CBS energies are about 0.5–2.0 kcal mol−1 lower. Recognizing that the present QCISD(T)/CBS method employs a much larger basis set for the QCISD(T) calculation, and thereby removes various additivity assumptions, this method is generally expected to be more accurate than the CBS-QB3 method. Sample comparisons with experiment for related systems indicate that the QCISD(T)/CBS predictions have uncertainties of about 1 kcal mol−1 even for the transition states. In the present work, the QCISD(T)/CBS energies were used in the rate coefficient calculations.The PES employed in the VRC-TST calculations of the barrierless radical–radical association reactions (3) and (4) was described in detail in Zhang et al.8 and hence will be only briefly summarized here. In the VRC-TST calculations, the intermolecular degrees of freedom of the fragments are treated as fully coupled anharmonic modes via classical phase space integrals. The interaction potential for these degrees of freedom was determined with on-the-fly CASPT2 calculations40 employing the aug-cc-pVDZ basis set. These calculations employed the minimum active space for properly describing the separated fragments, namely two electrons in two orbitals, (2e, 2o). Two orientation-independent correction terms were also included in the final PES to account for effects of increasing the basis set from aug-cc-pVDZ to aug-cc-pVTZ, and of relaxing the internal structure of the reacting fragments along the minimum energy path.
Besides the simple bond scission reactions (1) and (2), decomposition of MMH can also proceed via three-center, four-center, five-center, and roaming + abstraction transition states to different isomers and bimolecular products, as shown in Fig. 1. Intramolecular transfer of a primary amine H atom to the central NH group via the three-center transition state TS1 yields CH3NH2NH,
| CH3NHNH2 → CH3NH2NH | (7) |
Similarly, intramolecular transfer of a secondary amine H atom to the terminal NH2 group via TS2 yields CH3NNH3,
| CH3NHNH2 → CH3NNH3 | (8) |
These isomers can undergo further decomposition reactions, which will be discussed shortly.
In the previous study,3 two H2 elimination reaction paths via four-center transition states were found to have energy barriers of 106.9 kcal mol−1 and 108.7 kcal mol−1, respectively. Here, a new H2 elimination reaction path was explored
| CH3NHNH2 → CH2NHNH + H2 | (9) |
It involves a five-center transition state, TS3, with an energy barrier of 61.6 kcal mol−1, which is actually 1.1 kcal mol−1 lower than that of (1). However, TS3 contains a five-member-ring and thus has a relatively low entropy due to the loss of all the internal rotors. Nevertheless, (9) holds the potential to be a kinetically important channel for direct H2 formation. Another new H2 elimination pathway involving a three-center transition state, TS6, was also explored. Although the energy barrier for TS6 is 10 kcal mol−1 higher than that for TS3, it has a larger entropy than that of TS3, and hence (10) may compete with (9) at higher temperatures.
| CH3NHNH2 → CH3NHN + H2 | (10) |
As discussed in the previous study,3 the intramolecular transfer of the H atom from the secondary amine group in MMH to the methyl group via a three-center transition state (TS4) yields CH4 + NNH2, with an energy barrier of 66.1 kcal mol−1. Furthermore, the migration of the H atom from the methyl group to the terminal amino group in MMH via a four-center transition state TS5 yields the products CH2![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH + NH3 with an energy barrier of 69.3 kcal mol−1. The corresponding reaction paths are denoted by
NH + NH3 with an energy barrier of 69.3 kcal mol−1. The corresponding reaction paths are denoted by
| CH3NHNH2 → CH4 + NNH2 | (11) |
CH3NHNH2 → CH2![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH + NH3 NH + NH3
| (12) |
Since TS4 and TS5 have energies higher than that of (1), and are tighter, these reaction channels are not likely to make a significant contribution to the rate of MMH decomposition. However, they may provide the dominant pathways for CH4 and NH3 formation.
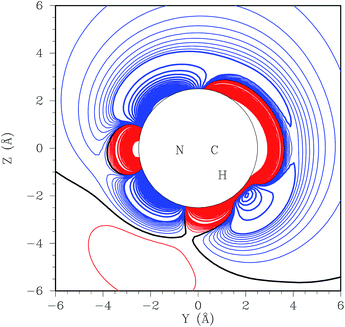
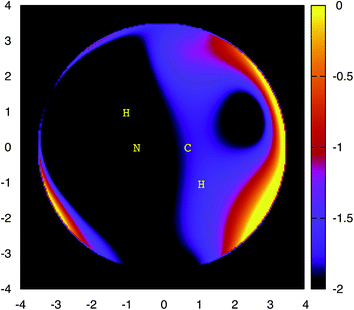
The N–N bond fission in CH3NHNH2 proceeds through a region of configurations corresponding to weakly interacting CH3NH and NH2 radicals. These radicals may reorient to place the NH2 closer to the C side of the CH3NH radical. From this side, there is a barrierless abstraction path to form NH3 + CH2NH. The plots in Fig. 2 and 3 provide two separate illustrations of these long-range interactions. The contour plot (Fig. 2) illustrates the interaction between the CH3NH and NH2 fragments for the N of NH2 in the NCH plane and the HNCH torsion of CH3NH roughly perpendicular to that plane. This plot indicates the presence of front and backside addition paths to form CH3NHNH2 (on the top and bottom left), an abstraction path to form CH2NH + NH3 (on the bottom right), and a roaming saddle point (at about y = 4, z = 0). The plot in Fig. 3 projects the interaction energy onto the plane for an NH2 group moving about the CH3NH at a fixed separation of 3.5 Å, but with the CH3NH now rotated so that the HNCH is in the plane of the plot. The addition paths now correlate with the black regions surrounding the N. The H that gets abstracted now lies out of the plane of the plot. The abstraction path then appears as the black region to the upper right of the C. The roaming dividing surface now shows up as the more or less vertical purple ridge passing through the C atom, with the roaming saddle point just above the C atom.
The fully optimized roaming saddle point for the reorientational motion, which is shown in Fig. 4, lies 1.2 kcal mol−1 below the threshold for forming NH2 + CH3NH. The roaming radical pathway provides the lowest energy route for decomposition and, at low enough temperatures, it provides the dominant decomposition path. At higher temperatures, entropic factors become more important and its role is reduced.
 | ||
| Fig. 4 CASPT2(2e, 2o)/cc-pVQZ geometry of the saddle point for NH2 roaming from the N side to the C side of CH3NH. | ||
3.2 Potential energy surface for CH3NH2NH decomposition
The CH3NH2NH isomer can also undergo further H2 and CH4 elimination to form products of diazenes and methyl diazenes, as shown in Fig. 5. For clarity, only the channels leading to the lower energy isomers of diazene and methyl diazene are shown in the figure. It is seen that the energies of their corresponding transition states, TS1H and TS1M, are too high for them to be the dominant pathways for MMH decomposition, although they may contribute to the formation of H2 and CH4, respectively. CH3NH2NH can also undergo N–N bond fission to form CH3NH2 + NH. The dissociation limit for formation of CH3NH2 + NH(a1Δ) radical is 29.8 kcal mol−1 above the products of (1). However, the singlet–triplet (1Δ–3∑) splitting of 35.9 kcal mol−1 (ref. 41) implies that CH3NH2 + NH(X3∑−) is at 56.6 kcal mol−1, well below the CH3NH + NH2 threshold. The contribution of this triplet channel (5) depends on the rate of intersystem crossing.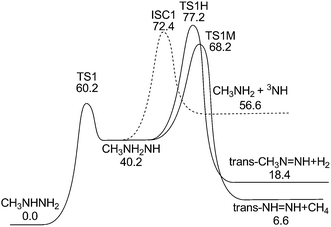 | ||
| Fig. 5 Potential energy surface for CH3NH2NH decomposition at the QCISD(T)/CBS//B3LYP/6-311++G(d,p) level (including ZPE correction) (unit: kcal mol−1). | ||
To locate the minimum singlet–triplet crossing point for (5), we first examined the minimum energy paths for CH3NH2NH → CH3NH2 + NH on both the singlet and triplet PESs at the CASPT2(2e, 2o)/aug-cc-pVDZ level, as shown in Fig. 6. The zero of energy in this plot corresponds to separated CH3NH2 + 3NH. The singlet and triplet potential curves cross each other at an N–N bond length of 1.98 Å, where the energy is about 72.0 kcal mol−1 relative to CH3NHNH2. The approximate crossing geometry for the singlet state was used as an initial guess in searching for the MSX.
 | ||
| Fig. 6 Potential curves for 1CH3NH2NH → CH3NH2 + 1NH (dashed) and 3CH3NH2NH → CH3NH2 + 3NH (solid) at the CASPT2(2e, 2o)/aug-cc-pVDZ level. | ||
Fig. 7(a) shows the geometry of the MSX at the CASSCF/6-311++G(d,p) level. The QCISD(T)/CBS energy for the singlet and triplet states at this geometry are 69.3 kcal mol−1 and 77.7 kcal mol−1, respectively. Notably, the T1 diagnostics for both states are quite small, being only 0.022 and 0.017 for the singlet and triplet states, respectively, implying only weak multireference effects for this geometry. Unfortunately, the 8 kcal mol−1 difference between the two QCISD(T) energies implies some dependence of the crossing geometry on dynamical correlation effects.
 | ||
| Fig. 7 Geometry of (a) the MSX for CH3NH2NH → CH3NH2 + NH at the CASSCF/6-311++G(d,p) level and (b) the corresponding approximate MSX at the CAS+1+2+QC(2e, 2o)/aug-cc-pVDZ level. | ||
To explore this effect, geometries on the CAS+1+2+QC(2e, 2o)/aug-cc-pVDZ crossing surface were found for a range of NN separations, as described in Section 2. The minimum of these crossing points was located at RN–N = 2.07 Å and the geometry (cf. Fig. 7(b)) is very similar to the CASSCF geometry. Notably, at this geometry the QCISD(T)/CBS energies for the singlet and triplet states are much closer, being 75.3 and 72.4 kcal mol−1, respectively. These observations suggest that the proper crossing point is likely at an energy of about 74 kcal mol−1, with an uncertainty of perhaps 5 kcal mol−1. Since this estimate for the MSX energy is more than 10 kcal mol−1 higher than the threshold for (1), the spin-forbidden channel (5) is unlikely to be kinetically significant. Thus, it will not be considered in the present rate calculations. This result is consistent with the observation of Golden et al.,13 that there is no indication of CH3NH2 in their very low pressure pyrolysis (VLPP) experiments, in which the radical–radical routes for CH3NH2 formation, such as CH3 + NH2 → CH3NH2, were suppressed.
3.3 Potential energy surface for CH3NNH3 decomposition
The CH3NNH3 isomer can undergo an H2 elimination reaction to form CH2NNH2, as shown in Fig. 8. The energy of the corresponding transition state, TS2H-a, is higher than the dissociation limits of (1) and (2) and consequently unlikely to be kinetically important. Another H2 elimination reaction of CH3NNH3 forms methyl diazene. The energy of the corresponding transition state, TS2H-b, is too high to be kinetically important.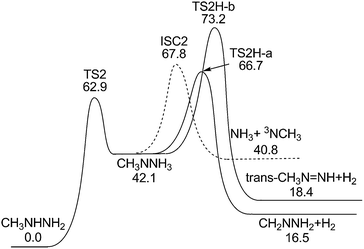 | ||
| Fig. 8 Potential energy surface for CH3NNH3 decomposition at the QCISD(T)/CBS//B3LYP/6-311++G(d,p) level (including ZPE correction) (unit: kcal mol−1). | ||
For CH3NNH3, the N–N bond fission yields NH3 and an NCH3 radical, where the ground state of NCH3 is again a triplet. The dissociation limit for the formation of the NCH3(X3A2) radical is only 40.8 kcal mol−1. This value indicates that the reaction (6) may also be an important channel for MMH decomposition and NH3 formation. The singlet–triplet adiabatic energy separation of 31.2 kcal mol−1 (ref. 42) again implies that the corresponding singlet products are kinetically inaccessible.
Following the same approach used to study (5), we first examined the minimum energy paths for CH3NNH3 → NH3 + NCH3 on both the singlet and triplet PESs at the CASPT2(2e, 2o)/aug-cc-pVDZ level, as shown in Fig. 9. In this plot the zero of energy now corresponds to separated NH3 + 3NCH3. The singlet and triplet potential curves cross each other at an N–N bond length of 1.92 Å, where the energy is about 65.8 kcal mol−1 relative to CH3NHNH2. The approximate crossing geometry for the singlet state was used as an initial guess in searching for the MSX.
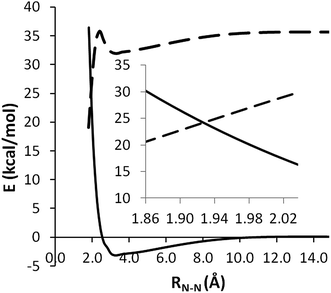 | ||
| Fig. 9 Potential curves for 1CH3NNH3 → NH3 + 1NCH3 (dashed) and 3CH3NNH3 → NH3 + 3NCH3 (solid) at CASPT2(2e, 2o)/aug-cc-pVDZ level. | ||
Fig. 10(a) shows the geometry of the MSX at the CASSCF/6-311++G(d,p) level. The QCISD(T)/CBS energies for the singlet and triplet states are 65.5 kcal mol−1 (T1 diagnostic = 0.018) and 72.1 kcal mol−1 (T1 diagnostic = 0.016), respectively. The location of CAS+1+2+QC(2e, 2o)/aug-cc-pVDZ crossing points as a function of RN–N again yields geometries (c.f. Fig. 10(b)) that are very similar to the CASSCF geometry. The corresponding splitting between the QCISD(T)/CBS singlet and triplet energies, which are now 67.8 and 72.0 kcal mol−1, is again reduced. These observations suggest that the proper crossing point is likely at an energy of 70 ± 5 kcal mol−1 and, once again, this spin-forbidden channel is unlikely to be kinetically significant for MMH decomposition. Thus, it will not be considered in the following rate calculations.
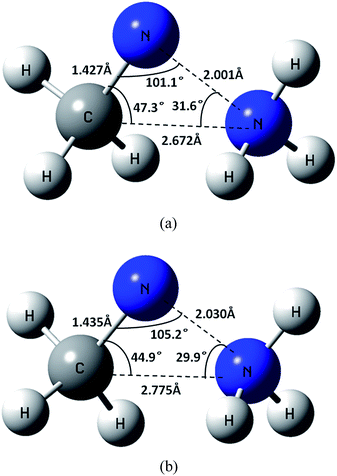 | ||
| Fig. 10 Geometry of (a) the MSX for CH3NNH3 → NH3 + NCH3 at the CASSCF/6-311++G(d,p) level and (b) the corresponding approximate MSX at the CAS+1+2+QC(2e, 2o)/aug-cc-pVDZ level. | ||
3.4 High pressure kinetics of MMH decomposition
For the barrierless (3) and (4) reactions, high pressure rate coefficients were calculated previously8 with the VRC-TST method,10,11,43 and then were converted to high pressure dissociation rate coefficients via computation of the equilibrium constant. The temperature dependence of the high-pressure decomposition and isomerization rate coefficients for MMH is shown in Fig. 11. The fits of the high-pressure rate constants over the temperature range of 400–2500 K by the modified Arrhenius function are listed in Table 1.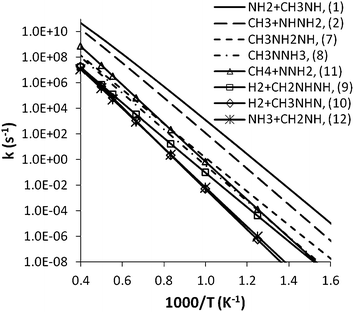 | ||
| Fig. 11 Temperature dependence of the high pressure rate coefficients for MMH dissociation and isomerization. | ||
| Reaction | P(N2)/atm | A/s−1 | B | C/cal mol−1 | D/s−1 | E | F/cal mol−1 |
|---|---|---|---|---|---|---|---|
| CH3NHNH2 → CH3NH + NH2 | 0.0013 | 1.02 × 1067 | −16.73 | 76![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 800 800 |
1.70 × 1059 | −14.10 | 79![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
| 0.013 | 6.64 × 1058 | −13.84 | 74![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
2.72 × 1050 | −11.57 | 72![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| 0.13 | 1.00 × 1029 | −9.00 | 45![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
2.65 × 1056 | −12.82 | 74![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 400 400 |
|
| 1 | 2.00 × 1038 | −11.70 | 48![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.91 × 1054 | −11.93 | 74![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
|
| 10 | 3.10 × 1075 | −19.99 | 75![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.32 × 1054 | −11.57 | 76![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 700 700 |
|
| 100 | 1.55 × 1058 | −14.00 | 71![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
2.26 × 1051 | −10.50 | 77![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
|
| ∞ | 4.55 × 1023 | −2.15 | 64![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 670 670 |
||||
| CH3NHNH2 → CH3 + NHNH2 | 0.0013 | 4.47 × 1055 | −13.76 | 75![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
3.25 × 1021 | −3.71 | 73![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 200 |
| 0.013 | 1.00 × 1057 | −13.84 | 74![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 990 990 |
3.55 × 1049 | −11.57 | 75![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| 0.13 | 1.00 × 1040 | −8.00 | 96![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
1.30 × 1056 | −13.08 | 76![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 080 080 |
|
| 1 | 1.51 × 1053 | −16.68 | 54![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.10 × 1055 | −12.44 | 76![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 900 900 |
|
| 10 | 2.08 × 1035 | −15.94 | 24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100 100 |
2.69 × 1053 | −11.62 | 78![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 298 298 |
|
| 100 | 2.39 × 1008 | −0.94 | 46![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
7.94 × 1048 | −10.02 | 77![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 750 750 |
|
| ∞ | 5.65 × 1019 | −1.12 | 65![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 640 640 |
||||
| CH3NHNH2 → CH2NHNH + H2 | 0.0013 | 1.26 × 1052 | −13.34 | 70![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 400 400 |
|||
| 0.013 | 7.21 × 1052 | −13.35 | 71![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 640 640 |
2.82 × 1047 | −11.67 | 79![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 900 900 |
|
| 0.13 | 1.26 × 1089 | −27.00 | 73![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
4.90 × 1054 | −13.55 | 74![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 600 600 |
|
| 1 | 2.04 × 1041 | −10.32 | 65![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
5.25 × 1053 | −12.99 | 75![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 900 900 |
|
| 10 | 6.74 × 1046 | −11.96 | 67![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.60 × 1051 | −11.92 | 77![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100 100 |
|
| 100 | 1.00 × 1050 | −12.50 | 70![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
3.95 × 1048 | −10.83 | 79![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 047 047 |
|
| ∞ | 1.46 × 1006 | 1.82 | 57![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 930 930 |
||||
| CH3NHNH2 → CH4 + NNH2 | 0.0013 | 1.35 × 1049 | −12.79 | 71![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300 300 |
2.68 × 1021 | −4.65 | 68![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
| 0.013 | 1.08 × 1047 | −11.73 | 71![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 750 750 |
3.16 × 1046 | −11.57 | 78![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
|
| 0.13 | 3.09 × 1001 | 1.55 | 57![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 600 600 |
1.05 × 1052 | −12.68 | 76![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100 100 |
|
| 1 | 1.00 × 1052 | −16.73 | 56![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 600 600 |
1.48 × 1054 | −12.88 | 79![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| 10 | 3.16 × 1030 | −6.84 | 64![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.76 × 1050 | −11.32 | 79![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300 300 |
|
| 100 | 1.14 × 1048 | −11.36 | 74![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
8.87 × 1052 | −11.62 | 85![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100 100 |
|
| ∞ | 1.51 × 1007 | 2.15 | 63![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 330 330 |
||||
CH3NHNH2 → CH2![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH + NH3 (12) NH + NH3 (12) |
0.0013 | 3.84 × 1038 | −10.51 | 71![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300 300 |
1.17 × 1011 | −2.54 | 62![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
| 0.013 | 2.66 × 1041 | −10.78 | 73![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 445 445 |
3.98 × 1039 | −10.00 | 90![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 400 400 |
|
| 0.13 | 2.45 × 1060 | −16.00 | 82![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
7.24 × 1040 | −10.08 | 75![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| 1 | 9.25 × 1027 | −10.43 | 48![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
8.31 × 1049 | −12.28 | 79![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 200 |
|
| 10 | 6.67 × 1030 | 9.06 | 36![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.70 × 1050 | −11.89 | 81![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 550 550 |
|
| 100 | 1.58 × 1045 | −11.30 | 74![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 200 |
3.16 × 1050 | −11.47 | 85![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 400 400 |
|
| ∞ | 5.12 × 1008 | 1.27 | 67![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 350 350 |
||||
| CH3NHNH2 → CH3NHN + H2 | 0.0013 | 1.27 × 1035 | −9.82 | 68![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
2.40 × 1014 | −3.51 | 68![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
| 0.013 | 8.37 × 1033 | −8.83 | 70![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 255 255 |
5.01 × 1040 | −10.00 | 107![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| 0.13 | 9.70 × 1080 | −24.95 | 78![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
4.17 × 1042 | −10.73 | 76![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 140 140 |
|
| 1 | 6.31 × 1088 | −31.00 | 63![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
2.34 × 1046 | −11.33 | 78![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 748 748 |
|
| 10 | 6.03 × 1039 | −10.04 | 72![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.05 × 1050 | −11.80 | 83![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 600 600 |
|
| 100 | 1.86 × 1044 | −11.28 | 74![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 200 |
6.92 × 1048 | −11.01 | 85![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300 300 |
|
| ∞ | 2.52 × 1006 | 2.04 | 68![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 030 030 |
||||
| CH3NHNH2 → CH3NH2NH | 0.0013 | 3.47 × 1050 | −12.56 | 69![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 200 |
1.41 × 1055 | −13.99 | 70![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 200 |
| 0.013 | 6.17 × 1053 | −13.22 | 71![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
6.31 × 1051 | −20.00 | 35![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 200 200 |
|
| 0.13 | 4.37 × 1033 | −8.88 | 55![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100 100 |
4.78 × 1054 | −13.19 | 73![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
|
| 1 | 3.55 × 1035 | −8.70 | 59![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
1.21 × 1054 | −12.75 | 75![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| 10 | 1.95 × 1036 | −8.03 | 64![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
5.81 × 1049 | −11.20 | 75![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| 100 | 3.31 × 1030 | −5.94 | 62![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 950 950 |
1.58 × 1049 | −10.69 | 78![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
|
| ∞ | 1.60 × 1006 | 2.05 | 56![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 040 040 |
||||
| CH3NHNH2 → CH3NNH3 | 0.0013 | 1.56 × 1034 | −8.13 | 70![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300 300 |
3.37 × 1059 | −15.57 | 74![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 750 750 |
| 0.013 | 5.01 × 1052 | −13.19 | 73![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 600 600 |
6.31 × 1044 | −11.82 | 65![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| 0.13 | 3.09 × 1001 | 1.55 | 57![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 600 600 |
1.00 × 1052 | −12.68 | 73![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 920 920 |
|
| 1 | 5.37 × 1048 | −12.35 | 69![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
7.92 × 1053 | −12.88 | 77![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| 10 | 4.03 × 1039 | −9.50 | 66![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
9.94 × 1051 | −11.93 | 78![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 600 600 |
|
| 100 | 4.39 × 1034 | −7.26 | 67![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
1.46 × 1051 | −11.31 | 82![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 000 |
|
| ∞ | 1.42 × 1007 | 1.83 | 60![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 060 060 |
Several useful observations about these rate calculations can be made. First, the two simple bond fission channels, especially (1), dominate the decomposition and isomerization kinetics of MMH over the entire temperature range of interest. This result implies that other channels have only secondary contributions to the total decomposition rate. Second, although the isomerization reaction (7) and the hydrogen elimination reaction (9) have slightly lower thresholds than (1), their decomposition rates are much smaller than those of (1) and (2) due to their tighter transition states. Third, the increasing importance of the CH4 elimination reaction (11) with temperature implies that it may be an important channel for CH4 formation at high temperatures. Finally, the hydrogen elimination rate of (9) is generally much larger than that of (10), which however becomes comparable to the former at higher temperatures due to its looser transition state structure, as discussed in Section 3.1.
3.5 High pressure kinetics of NH3 formation
In the VLPP experiments of Golden et al.,13 the product peaks at 17 amu (NH3) and 2 amu (H2) were used to compute total rate constants and the branching fraction for the formation of ammonia. The observation of only a small effect upon adding NO2 to the mixture, was taken to indicate the absence of any radical products. It was then proposed that ammonia is formed via the four-center deamination reaction (12).In VLPP experiments, collisions with a bath gas are replaced with wall collisions. This replacement makes it difficult to directly compare master equation predictions with VLPP data. Golden et al. obtained a high-pressure rate coefficient of 1013.2−54000/2.3RT s−1 for the reaction by fitting RRKM and RRK calculations to the predictions. There are two aspects of these indirect results that are strongly discordant with the present calculation, as seen in Fig. 12. First, the predicted rate constant for NH3 formation exceeds our high pressure rate constant for (12) by at least two orders of magnitude. Such a large disagreement cannot be explained by considering the contribution from the other NH3 forming channel, (7), because its NH3 formation rate is still much smaller than the experimental data even if we assume all of the CH3NNH3 decomposes to 3CH3N + NH3. Second, their NH3 formation rate coefficient roughly corresponds to their estimated total rate coefficient, which is about two orders of magnitude lower than our predicted rate for channel (1).
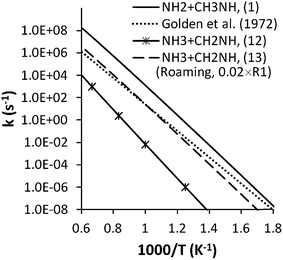 | ||
| Fig. 12 Rate coefficients for ammonia formation from MMH decomposition at different temperatures. The rate coefficient for the roaming channel ((13)) was estimated as 2% (the approximate branching ratio near 1000 K) of that for (1). | ||
Interestingly, a roaming radical pathway44,45 for forming NH3 + CH2![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH via
NH via
CH3NHNH2 → NH2⋯CH3NH → NH3 + CH2![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH NH
| (13) |
![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH. The present calculations suggest that the roaming radical channel contributes between 1.5 and 2.5% of the N–N bond fission rate for temperatures ranging from 500 to 2000 K and pressures ranging from 0.0013 atm to 100 atm. This fraction increases dramatically with decrease in temperature below 500 K reaching about 50% at 100 K, but little dissociation occurs at such low temperatures. Notably, in the 1000 K range this contribution to the NH3 formation rate is reasonably close to the value estimated by Golden et al.13 We expect considerable uncertainty (e.g., a factor of 5) in this prediction of the roaming contribution due to our use of harmonic oscillator assumptions for the roaming transition state.
NH. The present calculations suggest that the roaming radical channel contributes between 1.5 and 2.5% of the N–N bond fission rate for temperatures ranging from 500 to 2000 K and pressures ranging from 0.0013 atm to 100 atm. This fraction increases dramatically with decrease in temperature below 500 K reaching about 50% at 100 K, but little dissociation occurs at such low temperatures. Notably, in the 1000 K range this contribution to the NH3 formation rate is reasonably close to the value estimated by Golden et al.13 We expect considerable uncertainty (e.g., a factor of 5) in this prediction of the roaming contribution due to our use of harmonic oscillator assumptions for the roaming transition state.
The NH3 in the VLPP experiment could alternatively be attributed to a hydrogen abstraction reaction of NH2 from CH3NHNH2. Such radical–molecule reactions, which are generally considered to be suppressed in VLPP, will be discussed in the next section.
3.6 High pressure kinetics of H2 formation
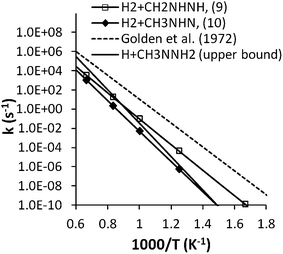
A large amount of hydrogen was found in the experiment of Golden et al.13 and proposed to be produced from the reaction MMH → CH3N![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH + H2 via a four-center transition state. Their high-pressure rate coefficient, 1013.5−57000/2.3RT s−1, for this reaction was again obtained by fitting their experimental results to predictions from RRKM and RRK theories. As shown in Fig. 13, neither the individual reactions (9) and (10) nor their combinations are sufficient to explain the estimates of Golden et al. For the channel proposed by Golden et al., MMH → CH3N
NH + H2 via a four-center transition state. Their high-pressure rate coefficient, 1013.5−57000/2.3RT s−1, for this reaction was again obtained by fitting their experimental results to predictions from RRKM and RRK theories. As shown in Fig. 13, neither the individual reactions (9) and (10) nor their combinations are sufficient to explain the estimates of Golden et al. For the channel proposed by Golden et al., MMH → CH3N![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH + H2, the presently calculated energy for its transition state is about 35–45 kcal mol−1 higher than that of (9) and (10), as discussed in Section 3.1. As a result, the rate coefficient of MMH → CH3N
NH + H2, the presently calculated energy for its transition state is about 35–45 kcal mol−1 higher than that of (9) and (10), as discussed in Section 3.1. As a result, the rate coefficient of MMH → CH3N![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH + H2 is simply too small to be kinetically important for hydrogen formation. By the same token, the reactions MMH → CH3NH2NH → CH3N
NH + H2 is simply too small to be kinetically important for hydrogen formation. By the same token, the reactions MMH → CH3NH2NH → CH3N![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH + H2, MMH → CH3NNH3 → CH2NNH2 + H2, and MMH → CH3NNH3 → CH3N
NH + H2, MMH → CH3NNH3 → CH2NNH2 + H2, and MMH → CH3NNH3 → CH3N![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH + H2 have negligible contributions to hydrogen formation due to their high energy transition state barriers and therefore small rate coefficients, as already seen in Fig. 5 and 8.
NH + H2 have negligible contributions to hydrogen formation due to their high energy transition state barriers and therefore small rate coefficients, as already seen in Fig. 5 and 8.
Sun and Law3 reported three barrierless reaction channels for H radical elimination, namely
| CH3NHNH2 → CH3NNH2 + H | (14) |
| CH3NHNH2 → CH3NHNH + H | (15) |
| CH3NHNH2 → CH2NNH2 + H | (16) |
In the present study, we repeated the geometry optimization and the higher level energy calculation for (14)–(16), among which (14) was found to have the smallest dissociation energy, which is 78.3 kcal mol−1 at the QCISD(T)/CBS//6-311++G(d,p) level, as compared to the 79 kcal mol−1 reported in Sun and Law.3 Furthermore, the dissociation energies of (15) and (16) were found to be 2.4 kcal mol−1 and 13.6 kcal mol−1 higher than that of (14), respectively.
To examine the possible significance of the roaming channel MMH → CH3NNH2 + H → CH2NNH2 + H2 for hydrogen formation, we made the following rough estimation of the upper bound for the dissociation rate of (14). First, we assumed the reverse reaction of (14) has an association rate constant of 4 × 10−10 cm3 per molecule per s, which should be an upper bound for all relevant temperatures and pressures. The dissociation rate can be estimated by using the association rate and an accurately calculated equilibrium constant. As seen in Fig. 13, the estimated dissociation rate for (14) is substantially smaller than the experimental data by a factor of 10–100 for temperatures between 900–1200 K. The branching ratio of a roaming induced abstraction channel to the radical–radical dissociation channel is generally much less than unity (typically 10% or less) in this temperature range. Thus, the roaming channel cannot explain the large amount of H2 formation in the experiment of Golden et al.13
Since we have now ruled out all the possible hydrogen formation channels on the PES of MMH decomposition, we reconsider the possibility of hydrogen formation via radical-involved secondary reactions, which are generally believed to be insignificant in the VLPP experiment. The most likely radical–molecule reactions for hydrogen formation are the hydrogen abstraction reactions
| H + CH3NHNH2 → CH3NNH2 + H2 | (17) |
| H + CH3NHNH2 → CH3NHNH + H2 | (18) |
| H + CH3NHNH2 → CH2NHNH2 + H2 | (19) |
An analysis of the relative importance of radical–molecule reactions in the VLPP experiment of Golden et al.13 is given in the Appendix. A critical rate constant, kM,cr = 3.2 × 10−12 cm3 per molecule per s, was derived based on the experimental condition of about 4 × 10−5 torr pressure and typical temperature of 1000 K. Based on the analysis, a radical–molecule reaction cannot be neglected in their experiment if its bimolecular reaction rate is close to or larger than the critical value.
The CCSD(T)/6-31+G(d,p)//MP2(full)/6-31+G(d,p) based ab initio transition state theory predictions of Sun and Law3 for (17)–(19) indicate that only the rate coefficient for (17), which is about 7.94 × 10−13 cm3 per molecule per s at 1000 K, is close to the critical rate constant and therefore holds potential importance to hydrogen formation. However, a reexamination of these rate coefficients at the QCISD(T)/CBS//B3LPY/6-311++G(d,p) level by Sun et al.46 yields much larger rate coefficients of 5.5 × 10−12, 4.7 × 10−12, and 2.5 × 10−12 cm3 per molecule per s, for (17), (18), and (19) respectively, which are all comparable to kM,cr. Consequently, (17)–(19) each hold the potential to be important in interpreting the hydrogen formation in the VLPP experiment and merit further study in modeling the experiment.
Sun and Law also predicted the rate coefficients for hydrogen abstraction reactions of MMH by the NH2 radical.3 Their predictions indicate that the highest rate coefficient for these reactions, corresponding to the attack of NH2 to the central amine hydrogen, is about 2.0 × 10−13 cm3 per molecule per s at 1000 K, which is well below the critical value. Cook et al. increased the rate by a factor of 10 to fit their measured NH2 and NH3 time history.6 However, such a modification was not supported by a reexamination of the rate at the QCISD(T)/CBS//B3LPY/6-311++G(d,p) level by Sun et al.46 Consequently, the contribution to ammonia formation from these reactions is presumed negligible here. This expectation provides support for our suggestion in Section 3.5 that the roaming channel (13) correlates well with the observed ammonia formation in the VLPP experimental conditions.
Another possible explanation for the large amount of hydrogen observed in the experiment of Golden et al. involves heterogeneous MMH decomposition. In fact, a substantial amount of hydrogen formed from the heterogeneous decomposition of MMH was observed in the experiment of Kerr et al.12 and the assumed reaction path MMH → CH4 + H2 + N2 agrees well with their experimental measurement. Moreover, their experiment confirmed that ammonia is not formed heterogeneously, at least under their experimental conditions.
3.7 High pressure kinetics of CH4 formation
Although CH4 was not observed in Golden et al.'s VLPP experiment, the present study shows that it can be directly formed (via (11)) from the MMH decomposition, especially at high temperatures. The pathway CH3NH2NH → CH4 + NH![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH is unlikely to be as important as (11) because of its higher energy barrier. Similar to (13), a roaming radical pathway via
NH is unlikely to be as important as (11) because of its higher energy barrier. Similar to (13), a roaming radical pathway via
CH3NHNH2 → CH3⋯NHNH2 → CH4 + NH![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH NH
| (20) |
![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) NH. This pathway would be expected to produce CH4 with a rate coefficient that is generally about 1–10% of that for (2).
NH. This pathway would be expected to produce CH4 with a rate coefficient that is generally about 1–10% of that for (2).
CH4 was observed in the experiment of Kerr et al.,12 who attributed it to the heterogeneous decomposition of MMH, as discussed in Section 3.6. A comparison between the present theory and their experiment is difficult because secondary radical reactions may play an important role under their experimental conditions where the pressures are 0.01–0.04 atm.
3.8 Pressure dependent kinetics
Based on the above discussion of the PES for the decomposition of MMH, we can simplify the multi-well, multi-channel PES of MMH decomposition to a single-well, multi-channel one, thereby avoiding the complication of dealing with the multi-well master equation.34 The contributions of the newly identified reaction channels for MMH decomposition are illustrated in Fig. 14, where their pressure dependent reaction rates are shown for pressures ranging from 0.0013 to 100 atm for a temperature of 1000 K. The pressure-dependent rates for the roaming channel, (13), were estimated with a constant branching ratio of 2%. It is seen that (1), (2), and (13) dominate the MMH decomposition over the entire pressure range and contributions from other channels are negligible.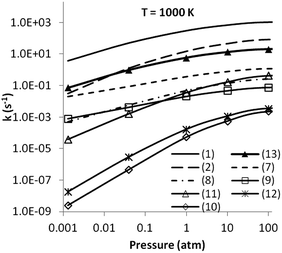 | ||
| Fig. 14 Pressure dependent rate coefficients for the dissociation and isomerization of MMH at a temperature of 1000 K. The rate coefficient for the roaming channel (13) was estimated as 2% (the approximate branching ratio near 1000 K from 0.001 atm to 100 atm) of that for (1). | ||
In our previous study,8 the experimental data of Kerr et al.,12 who measured the first-order rate coefficient for (1) at 0.01–0.04 atm and 750–860 K, were well reproduced by the theoretical rate coefficients calculated with 〈ΔEdown〉 = 200 (T/300)0.85 cm−1 for the bath gas N2. Considering that toluene was in high concentration in the toluene-carrier flow system of Kerr et al., we now also performed calculations for toluene as the bath gas using Lennard-Jones parameters, σ = 4.7 Å and ε = 150 cm−1. Given the larger molecular size and the presence of a torsional mode in toluene one expects the 〈ΔEdown〉 to be somewhat larger for it. Here we consider two separate values of 〈ΔEdown〉 for toluene of 300(T/300)0.85 and 600(T/300)0.85 cm−1. Several observations can be made from the comparison, as shown in Fig. 15. Overall, the agreement with the experimental data of Kerr et al. is quite reasonable, especially for the calculation at 0.04 atm, with the results for 〈ΔEdown〉 = 600(T/300)0.85 cm−1 providing the best fit to the data. However, the theoretical rate coefficients show a pressure fall-off of about a factor or 3 from 0.04 atm to 0.01 atm, while this pressure effect is absent in the experimental data of Kerr et al. It is not clear why this should be the case. Further comparisons between these parameters are not possible in the present study.
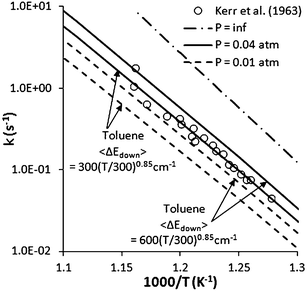 | ||
| Fig. 15 Pressure dependent rate coefficients for (1). | ||
In our previous study,8 the reactions (1) and (2) were found to be insufficient to explain the experimental data of Eberstein and Glassman5 for the total thermal decomposition rates of MMH at 750–1000 K and atmospheric pressure. Since other channels considered in the present study do not make a major contribution to the MMH dissociation, such a disagreement still holds and suggests that one cannot expect to properly explain the experimental results without considering the effects of secondary radical–molecule and/or radical–radical reactions arising from the MMH decomposition products.
Recently, Li et al.7 reported rate coefficients for (1) at 0.3–5.2 atm based on NH2 time-history measurements in shock-tube experiments with argon as the bath gas. The measured rate coefficients follow the same pressure fall-off trends as our previous theoretical predictions.8 However, a reduction of 40% in the theoretical rate coefficients was needed to reproduce their experimental data. To understand this discrepancy, we note that the average downward energy transfer used in our previous theoretical rates was based on a fit to the experimental data of Kerr et al., where toluene was used as bath gas. Apparently, a larger 〈ΔEdown〉 was needed to model the collisional energy transfer between MMH and toluene, as noted above. For the present study, we have recalculated the rates for Ar as the bath gas and using an average energy down 〈ΔEdown〉 = 130(T/300)0.85cm−1, where the room temperature 〈ΔEdown〉 = 130 cm−1 was suggested by Gilbert and Smith.39 Notably, this value is close to those employed in our studies of C3H8 [100 (T/300)0.85 cm−1],47 CH3CH2OH [125 (T/300)0.85 cm−1],48 and CH3CHO [150 (T/300)0.85 cm−1],49 which are of similar size and bond energies. As shown in Fig. 16, these revised theoretical predictions agree very well with Li et al.'s experimental data, with discrepancies within the experimental accuracy.
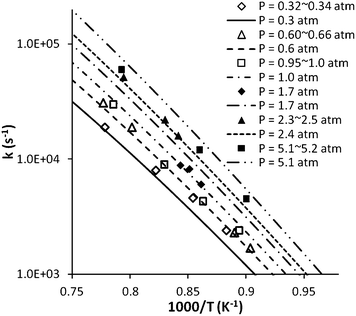 | ||
| Fig. 16 Pressure dependent rate coefficients for (1). Scatter points represent experimental data of Li et al. (2014) and lines the present calculation data. | ||
For all the reactions on the PES of MMH decomposition, the calculated fall-off data in the ranges of 400–2500 K and 0.0013–100 atm were fitted with a form that can be readily used in the modeling of MMH pyrolysis and oxidation. These calculations were for N2 as a bath gas with 〈ΔEdown〉 = 120(T/300)0.85 cm−1. The fitting coefficients are listed in Table 1.
4. Conclusions
The decomposition kinetics of monomethylhydrazine was studied with ab initio transition state theory based master equation calculations. In addition to the simple N–N and C–N bond fissions, new reaction pathways were identified with energies for all of the stationary points evaluated at the QCISD(T)/CBS//B3LYP/6-311++G(d,p) or QCISD(T)/CBS//CAS+1+2+QC/aug-cc-pVDZ level.The high pressure rate coefficients were calculated from transition state theory and compared with available experimental data. The roaming channel, MMH → CH3NH⋯NH2 → CH2NH + NH3, appears to be the dominant channel for ammonia formation and estimates of its rate constant agree well with the VLPP experiment of Golden et al.13 The possible reaction channels on the PES of MMH decomposition for hydrogen formation were identified and found to be insufficient to explain the large amount of hydrogen formation in the experiments. The possible contribution of radical–molecule reactions to hydrogen and ammonia formation was analyzed. The results show that the hydrogen abstraction channels of MMH by the hydrogen radical cannot be neglected in the VLPP experiments due to their high rate coefficients, while the hydrogen abstraction channels of MMH by the NH2 radical can be neglected due to their relatively low rate coefficients. Another possible explanation involves the heterogeneous decomposition of MMH, with hydrogen as a major product, while ammonia is absent.
The pressure dependence and product branching in MMH decomposition were obtained from solutions to the master equation. The new theoretical results agree well with the experimental data of Kerr et al.12 and of Li et al.7 It was also found that the calculated MMH decomposition rate coefficients are not sufficient to explain the measured total MMH loss rate of Eberstein and Glassman,5 and that secondary reactions involving MMH and its radicals must be considered. This shortcoming emphasizes the need for a full, detailed reaction mechanism.
Appendix
In the VLPP experiments of Golden et al.,13,50–53 bimolecular reactions are suppressed by operating the VLPP reactor at sufficiently low pressures and for sufficiently short residence times. The condition that bimolecular reactions can be neglected is given by54| fr = trkM[M] ≪ 1, | (21) |
In their VLPP study of MMH,13 Golden et al. did not explicitly specify the residence time or the concentration (pressure) for the experiment, and instead referred readers to ref. 50–53 for the apparatus and theory of VLPP. Nevertheless, they provided the frequency of gas-wall collisions in the reactor, ω = 7.7 × 103(T/W)1/2 s−1, where T is the temperature and W is the molar mass of M, and the flow rates F = 5 × 1015 to 5 × 1016 molecules per s. From this information we can still estimate the relevant experimental parameters as follows:50–54
First, we can obtain the residence time from the relation54
| ω = Zw/tr | (22) |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 400. Consequently, we can estimate the residence time from the expression
400. Consequently, we can estimate the residence time from the expression| tr = Zw/ω = 2.79 × 10−5Zw | (23) |
We can obtain the concentration via the relation
| F = [M]cAh/4, | (24) |
| [M] = 4F/cAh, | (25) |
From eqn (21) we can derive a criterion for the critical rate coefficient, kM,cr:
| kM,cr = 0.01/tr[M], | (26) |
Acknowledgements
The work at the Hong Kong Polytechnic University was supported by RGC/ECS (PolyU 5380/13E) and by SRFDP & RGC ERG Joint Research Scheme (M-PolyU509/13). The work at Princeton University was partially supported by the U.S. Army Research Office. The collaboration between Princeton University and the Argonne National Laboratory was facilitated through the Combustion Energy Frontier Research Center sponsored by the Department of Energy. The work at Argonne was supported by the Division of Chemical Sciences, Geosciences, and Biosciences, the Office of Basic Energy Sciences, the U.S. Department of Energy, under contract number DE-AC02-06CH11357. Support at ANL for the roaming radical calculations was provided by the Army Research Office under Grant #W911NF1310251 as part of their Molecular Structure and Dynamics program.References
- L. Catoire, N. Chaumeix and C. Paillard, J. Propul. Power, 2004, 20, 87–92 CrossRef CAS.
- E. W. Schmidt, Hydrazine and its derivatives: preparation, properties, applications, Wiley, New York, 2001 Search PubMed.
- H. Y. Sun and C. K. Law, J. Phys. Chem. A, 2007, 111, 3748–3760 CrossRef CAS PubMed.
- H. Y. Sun, L. Catoire and C. K. Law, Int. J. Chem. Kinet., 2009, 41, 176–186 CrossRef CAS.
- I. J. Eberstein and I. Glassman, Proc. Combust. Inst., 1965, 10, 365–374 CrossRef.
- R. D. Cook, S. H. Pyun, J. W. Cho, D. F. Davidson and R. K. Hanson, Combust. Flame, 2011, 158, 790–795 CrossRef CAS PubMed.
- S. J. Li, D. F. Davidson and R. K. Hanson, Combust. Flame, 2014, 161, 16–22 CrossRef CAS PubMed.
- P. Zhang, S. J. Klippenstein, H. Y. Sun and C. K. Law, Proc. Combust. Inst., 2011, 33, 425–432 CrossRef CAS PubMed.
- Y. Georgievskii and S. J. Klippenstein, J. Chem. Phys., 2003, 118, 5442–5455 CrossRef CAS PubMed.
- S. J. Klippenstein, J. Phys. Chem., 1994, 98, 11459–11464 CrossRef CAS.
- S. J. Klippenstein, J. Chem. Phys., 1992, 96, 367–371 CrossRef PubMed.
- J. A. Kerr, R. C. Sekhar and A. F. Trotman-Dickenson, J. Chem. Soc., 1963, 3217–3225 RSC.
- D. M. Golden, R. K. Solly, N. A. Gac and S. W. Benson, Int. J. Chem. Kinet., 1972, 4, 433–448 CrossRef CAS.
- A. D. Becke, J. Chem. Phys., 1993, 98, 5648–5652 CrossRef CAS PubMed.
- R. Krishnan, J. S. Binkley, R. Seeger and J. A. Pople, J. Chem. Phys., 1980, 72, 650–654 CrossRef CAS PubMed.
- T. H. Dunning, J. Chem. Phys., 1989, 90, 1007–1023 CrossRef CAS PubMed.
- R. A. Kendall, T. H. Dunning and R. J. Harrison, J. Chem. Phys., 1992, 96, 6796–6806 CrossRef CAS PubMed.
- J. M. L. Martin and O. Uzan, Chem. Phys. Lett., 1998, 282, 16–24 CrossRef CAS.
- B. G. Levine, J. D. Coe and T. J. Martinez, J. Phys. Chem. B, 2008, 112, 405–413 CrossRef CAS PubMed.
- D. G. Truhlar and C. A. Mead, Phys. Rev. A, 2003, 68, 032501 CrossRef.
- A. Farazdel and M. Dupuis, J. Comput. Chem., 1991, 12, 276–282 CrossRef CAS.
- M. J. Bearpark, M. A. Robb and H. B. Schlegel, Chem. Phys. Lett., 1994, 223, 269–274 CrossRef CAS.
- Q. Cui and K. Morokuma, Chem. Phys. Lett., 1997, 272, 319–327 CrossRef CAS.
- J. N. Harvey, M. Aschi, H. Schwarz and W. Koch, Theor. Chem. Acc., 1998, 99, 95–99 CrossRef CAS.
- P. J. K. H.-J. Werner, R. Lindh, F. R. Manby, M. Schütz, P. Celani, T. Korona, A. Mitrushenkov, G. Rauhut, T. B. Adler, R. D. Amos, A. Bernhardsson, A. Berning, D. L. Cooper, M. J. O. Deegan, A. J. Dobbyn, F. Eckert, E. Goll, C. Hampel, G. Hetzer, T. Hrenar, G. Knizia, C. Köppl, Y. Liu, A. W. Lloyd, R. A. Mata, A. J. May, S. J. McNicholas, W. Meyer, M. E. Mura, A. Nicklass, P. Palmieri, K. Pflüger, R. Pitzer, M. Reiher, U. Schumann, H. Stoll, A. J. Stone, R. Tarroni, T. Thorsteinsson, M. Wang and A. Wolf, MOLPRO, version 2008.1, a package of ab initio programs Search PubMed.
- M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, V. G. Zakrzewski, J. A. Montgomery, R. E. Stratmann, J. C. Burant, S. Dapprich, J. M. Millam, A. D. Daniels, K. N. Kudin, M. C. Strain, O. Farkas, J. Tomasi, V. Barone, M. Cossi, R. Cammi, B. Mennucci, C. Pomelli, C. Adamo, S. Clifford, J. Ochterski, G. A. Petersson, P. Y. Ayala, Q. Cui, K. Morokuma, D. K. Malick, A. D. Rabuck, K. Raghavachari, J. B. Foresman, J. Cioslowski, J. V. Ortiz, B. B. Stefanov, G. Liu, A. Liashenko, P. Piskorz, I. Komaromi, R. Gomperts, R. L. Martin, D. J. Fox, T. Keith, M. A. Al-Laham, C. Y. Peng, A. Nanyakkara, C. Gonzalez, M. Challacombe, P. M. W. Gill, B. Johnson, W. Chen, M. W. Wong, J. L. Andres, C. Gonzalez, M. Head-Gordon, E. S. Replogle and J. A. Pople, Gaussian 98, Gaussian, Inc., Pittsburgh, PA, 1998 Search PubMed.
- H.-J. Werner, P. J. Knowles, R. Lindh, M. Schutz, T. K. P. Celani, F. R. Manby, G. Rauhut, R. D. Amos, A. Bernhardsson, A. Berning, D. L. Cooper, M. J. O. Deegan, A. J. Dobbyn, F. Eckert, C. Hampel, G. Hetzer, A. W. Lloyd, S. J. McNicholas, W. Meyer, M. E. Mura, A. Nicklass, P. Palmieri, R. Pitzer, U. Schumann, H. Stoll, A. J. Stone and R. T. Tarroni, Molpro, Version 2006.1, a package of ab initio programs, 2006 Search PubMed.
- A. L. L. East and L. Radom, J. Chem. Phys., 1997, 106, 6655–6674 CrossRef CAS PubMed.
- Q. Cui, K. Morokuma, J. M. Bowman and S. J. Klippenstein, J. Chem. Phys., 1999, 110, 9469–9482 CrossRef CAS PubMed.
- J. N. Harvey, WIREs Comput. Mol. Sci., 2014, 4, 1–14 CrossRef CAS.
- S. J. Klippenstein and L. B. Harding, Proc. Combust. Inst., 2009, 32, 149–155 CrossRef CAS PubMed.
- C. A. Taatjes, D. L. Osborn, T. M. Selby, G. Meloni, A. J. Trevitt, E. Epifanovsky, A. I. Krylov, B. Sirjean, E. Dames and H. Wang, J. Phys. Chem. A, 2010, 114, 3355–3370 CrossRef CAS PubMed.
- S. J. Klippenstein, L. B. Harding, B. Ruscic, R. Sivaramakrishnan, N. K. Srinivasan, M. C. Su and J. V. Michael, J. Phys. Chem. A, 2009, 113, 10241–10259 CrossRef CAS PubMed.
- J. A. Miller and S. J. Klippenstein, J. Phys. Chem. A, 2006, 110, 10528–10544 CrossRef CAS PubMed.
- J. A. Miller and S. J. Klippenstein, J. Phys. Chem. A, 2003, 107, 2680–2692 CrossRef CAS.
- S. J. Klippenstein, A. F. Wagner, R. C. Dunbar, D. M. Wardlaw, S. H. Robertson and J. A. Miller, VARIFLEX: Version 2.0m, 2007 Search PubMed.
- J. A. Miller and S. J. Klippenstein, Phys. Chem. Chem. Phys., 2004, 6, 1192–1202 RSC.
- H. Wang and M. Frenklach, Combust. Flame, 1994, 96, 163–170 CrossRef CAS.
- R. G. Gilbert and S. C. Smith, Theory of Unimolecular and Recombination Reactions, Blackwell Scientific Publications, 1990 Search PubMed.
- K. Andersson, P. A. Malmqvist and B. O. Roos, J. Chem. Phys., 1992, 96, 1218–1226 CrossRef CAS PubMed.
- K. P. Huber and G. Herzberg, Constants of Diatomic Molecules, Van Nostrand Reinhold, New York, 1979 Search PubMed.
- M. J. Travers, D. C. Cowles, E. P. Clifford, G. B. Ellison and P. C. Engelking, J. Chem. Phys., 1999, 111, 5349–5360 CrossRef CAS PubMed.
- Y. Georgievskii and S. J. Klippenstein, J. Phys. Chem. A, 2003, 107, 9776–9781 CrossRef CAS.
- L. B. Harding, Y. Georgievskii and S. J. Klippenstein, J. Phys. Chem. A, 2009, 114, 765–777 CrossRef PubMed.
- L. B. Harding and S. J. Klippenstein, J. Phys. Chem. Lett., 2010, 1, 3016–3020 CrossRef CAS.
- H. Y. Sun, P. Zhang and C. K. Law, Thermal decomposition of monomethylhydrazine: reaction mechanism and kinetic modeling, 7th US National Technical Meeting of the Combustion Institute, Atlanta, GA, 2011 Search PubMed.
- R. Sivaramakrishnan, M. C. Su, J. V. Michael, S. J. Klippenstein, L. B. Harding and B. Ruscic, J. Phys. Chem. A, 2011, 115, 3366–3379 CrossRef CAS PubMed.
- R. Sivaramakrishnan, M. C. Su, J. V. Michael, S. J. Klippenstein, L. B. Harding and B. Ruscic, J. Phys. Chem. A, 2010, 114, 9425–9439 CrossRef CAS PubMed.
- L. B. Harding, Y. Georgievskii and S. J. Klippenstein, J. Phys. Chem. A, 2010, 114, 765–777 CrossRef CAS PubMed.
- P. C. Beadle, S. W. Benson, K. D. King and D. M. Golden, J. Am. Chem. Soc., 1972, 94, 2943–2947 CrossRef CAS.
- P. C. Beadle, D. M. Golden and S. W. Benson, Int. J. Chem. Kinet., 1972, 4, 265–271 CrossRef CAS.
- K. D. King, D. M. Golden, G. N. Spokes and S. W. Benson, Int. J. Chem. Kinet., 1971, 3, 411–426 CrossRef CAS.
- D. M. Golden, N. A. Gac, R. K. Solly and S. W. Benson, J. Am. Chem. Soc., 1972, 94, 363–369 CrossRef CAS.
- D. M. Golden, G. N. Spokes and S. W. Benson, Angew. Chem., Int. Ed. Engl., 1973, 12, 534–546 CrossRef.
- S. W. Benson and G. N. Spokes, J. Am. Chem. Soc., 1967, 89, 2525–2532 CrossRef CAS.
- M. J. Perona and D. M. Golden, Int. J. Chem. Kinet., 1973, 5, 55–65 CrossRef CAS.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/c4ra13131b |
| This journal is © The Royal Society of Chemistry 2014 |