KNa₃Mn₇(PO₄)₆: 2D spin-frustrated magnetic material with a diamond-like chain structure

Abstract

The search for spin frustrated magnets has attracted great scientific interest. Here we report on the synthesis of a novel two dimensional spin-frustrated compound KNa₃Mn₇(PO₄)₆ with a diamond-like chain structure. Our results confirm spin freezing at T_c = 3.9(1) K with the Weiss constant θ = −64.1(2) K, showing a remarkable spin frustration effect in the system. The origin of spin frustration is also suggested.

---

Geometrically frustrated magnets are the most known spin-frustrated materials in which antiferromagnetic interactions of localized spins can't be simultaneously satisfied, giving rise to a large degeneracy of the ground state.¹ Such a spin-frustration effect can be realized in many special spin lattices containing equilateral triangles or regular tetrahedra. Geometrically frustrated materials are usually found to exhibit a triangle, Kagomé, pyrochlore and spinel lattice structure, showing their exotic magnetic ground states such as spin glass, spin ices, and spin liquids.² In this respect, two-dimensional (2D) spin systems with a triangle or Kagomé lattice have attracted special attention in the past decades, since they may be considered as an ideal candidate for the investigation of quantum ground state responsible for the resonating valence bond model proposed by Anderson.³ For 2D triangle lattice, the anhydrous alums family A^IM^III(SO₄)₂ (A = K, Rb, Cs, M = transition metal ion) are well-known examples, in which magnetic M³⁺ ions form equilateral triangles in 2D planes.⁴ For 2D Kagomé lattice, the jarosite family with the formula AM₃X₆(SO₄)₂ (A = monovalent, divalent or trivalent cation, M = transition metal ion, X = OH or F) are typical examples, in which magnetic M³⁺ ions form equilateral triangles and further equilateral triangles connect to each other to form a 2D Kagomé lattice by corner-sharing.⁵ With intensive studies on 2D Kagomé lattice compounds, many hydroxide minerals such as Cu₃V₂O₇(OH)₂·2H₂O,⁶ ZnCu₃(OH)₆Cl₂,⁷ and BaM₃V₂O₈(OH)₂ (M = Cu, Ni)⁸ have been found that the triangular units of M₃(μ₃-X) construct Kagomé lattices through the secondary building units, showing an exotic magnetic behaviour with potential resonating valence state. Hence, it is a great challenge to design and synthesize geometrically frustrated materials with a new and promising spin-lattice.

On the other hand, one of the simplest one-dimensional (1D) frustrated geometry is diamond-chain where the triangles built by magnetic ions locate inside. Except for possible spin-frustration effect, transition-metal based compounds with a diamond-chain structure have also been found to exhibit various interesting magnetic properties.⁹ For example, 1/3 magnetization plateau can be observed in A₃Cu₃(PO₄)₄,¹⁰ Cu₂Cl₄·H₈C₄SO₂,¹¹ and Cu₃(CO₃)₂(OH)₂ (ref. 12) which are considered as S = 1/2 quantum frustrated magnets with a diamond-chain structure. However, Cu₃(TeO₃)₂Br₂ with a diamond-chain-like structure built by square planar [CuO₄] and trigonal bi-pyramidal [CuO₄Br], showing an antiferromagnetic ordering at 70 K with coexistence of ferromagnetic and antiferromagnetic interactions,¹³ while Bi₄Cu₃V₂O₁₄ with a distorted diamond-chain structure exhibits an antiferromagnetic ordering at 6 K and a metamagnetic transition at a critical field of H_c = 7.6 T.¹⁴ Many efforts have made for exploring new transition-metal compounds with a diamond chain structure instead of Cu ions. However there are a few compounds with diamond chain structure built by Co or Ni. Up to now, only [Co₄(phcina)₆(OH)₂(H₂O)₄]·2H₂O (phcina = α-phenylcinnamate)¹⁵ and [M^II₅(OH)₂(chtc)₂(H₂O)₁₀]·2H₂O (M = Co, Ni and chtc = cis,cis,cis-1,2,4,5-cyclohexanetetracarboxylate)¹⁶ have been reported to exhibit a diamond-chain structure.

In present study, we try to design and construct new geometrically frustrated magnetic materials using diamond-chain model as secondary building units. Here we report on successful synthesis of a new 2D phosphate KNa₃Mn₇(PO₄)₆ with diamond-like chain structure, which is one of the family formulated as ANa₃M₇(PO₄)₆ (A = K, Rb, Cs, and M = Mn, Fe, Co).¹⁷ Our experimental results show that the titled compound exhibits a long-range antiferromagnetic ordering at ∼4 K with a with the Weiss constant θ = −64.1(2) K, suggesting the appearance of spin frustration in the system.

Single crystals of KNa₃Mn₇(PO₄)₆ were synthesized by a conventional high-temperature solid-state reaction. A mixture of Na₂CO₃ (3 N, 0.2446 g), Mn(CH₃COO)₂ (3 N, 1.1446 g), NH₄H₂PO₄ (3 N, 0.5750 g) and KF (3 N, 0.1000 g) was ground carefully and homogenized thoroughly in an agate mortar. The mixture was packed into an alumina crucible and heated to 1153 K for 40 h and then cooled down to room temperature. Polycrystalline samples were prepared by mixture of Na₂CO₃, NH₄H₂PO₄, MnC₂O₄·2H₂O, and K₂CO₃ in molar ratio of 3 : 12 : 14 : 1 at 903 K for 60 h with several intermediate grindings. The quality of the powdered samples was confirmed by powder X-ray diffraction (see the Fig. S1 of ESI†). Powdered samples of KNa₃Mn₇(PO₄)₆ were placed in a gel capsules ample holder which was suspended in a plastic drinking straw. D_c magnetic susceptibility was measured at 0.1 T from 300 to 2 K and low-temperature susceptibility was measured at 0.05 and 0.1 T from 2 to 10 K (temperature scan of 5 K min⁻¹) with zero-field-cooling (ZFC) and field-cooling (FC) regimes using commercial Quantum Design MPMS-XL SQUID magnetometer. Magnetization was measured at 2 K in applied field from −8 to 8 T (field scan of 0.1 T/step) and ac magnetic susceptibilities were measured at an amplitude of 3 Oe with different frequencies from 50 to 10 000 Hz using Quantum Design Physical Property Measurement System (PPMS).

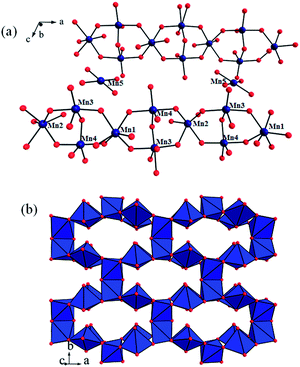
KNa₃Mn₇(PO₄)₆ crystallizes in the monoclinic space group C2/c with a = 13.165(8) Å, b = 10.907(6) Å, c = 15.960(1) Å, β = 113.243(9)° and Z = 4. The asymmetric unit contains one K, two Na, five Mn, and three P atoms. As seen in Fig. S2 of ESI,† we note that Mn²⁺ ions have five different sites, which exhibit multiple oxygen-coordinated modes with different polyhedral groups. Mn(1) and Mn(2) sites are coordinated by six oxygen atoms, forming distorted MnO₆ octahedra, while Mn(3) and Mn(4) sites are coordinated by five oxygen atoms, forming a square pyramidal MnO₅ and a trigonal bipyramidal MnO₅ geometry, respectively. Mn(5) sites are coordinated by four oxygen atoms, forming a quite unique planar square geometry.

To check the linkage of different polyhedra, we note that Mn(3)O₅ square pyramids connect to Mn(4)O₅ trigonal bipyramids via corner-sharing, forming a Mn(3)Mn(4)O₉ dimer. Further, such dimers connect to Mn(1) and Mn(2) via corner-sharing, forming an unique diamond-like chain running along the a-axis, in which Mn(5)O₄ planar squares are located between chains (Fig. 1a). Also, the diamond-like chains link to each other with the connection of Mn(1)O₆ and Mn(2)O₆ via edge-sharing along the b-axis, forming a 2D channel sheet on the a–b plane (Fig. 1b). As shown in Fig. S4 of ESI,† the 3D frameworks are built by the linkage of channel sheets and tetrahedral PO₄, in which the sodium atoms are located inside the channels. We note that the potassium atoms are embedded in the cavities arising from the linkage of 2D sheets and tetrahedral PO₄.

Fig. 1 (a) The linkage of [MnO_n] polyhedra in the title compound, in which the diamond-like chains are seen and the isolated Mn(5)O₄ planar squares are located between chains. (b) 2D channel sheets are seen on the ab-plane.

As shown in Fig. 2a, the susceptibility increases with increasing temperature, while a peak is observed at 4 K, indicating the onset of magnetic ordering. We note that the susceptibility above 70 K can be fitted well by the Curie–Weiss law, giving the Curie constant C = 4.11(2) emu K mol⁻¹ Mn²⁺ and Weiss temperature θ = −64.1(2) K. The effective magnetic moment (μ_eff) of Mn²⁺ ions is calculated to be 5.73(6) μ_B, which is smaller than the value of 5.916 μ_B for S = 5/2 with g = 2. This reduction of spin moments may be due to the Mn(5) sites (S = 3/2) with an outer electron configuration of (3d_xyd_xzd_yz)⁴(3dx² − y²)¹ in a square-oxygen-coordinated crystal field or slight spin-frustration in the system. Also, the negative Weiss temperature indicates that the dominative interactions between magnetic ions are antiferromagnetic in nature. The plot of χT versus temperature (T) is shown in Fig. 2b. The value of χT is ∼3.4 emu K mol⁻¹ Mn²⁺ at 300 K and keeps on falling with the decreasing temperature, confirming the dominative antiferromagnetic interaction exchanges in the system. Magnetic susceptibilities obtained with zero-field cooling (ZFC) and field cooling (FC) regimes are shown in Fig. 2c. A clear history is seen below 5 K between ZFC and FC regimes, confirming the onset of magnetic ordering. To further identify the nature of magnetic ordering at 4 K, the ac magnetic susceptibilities are measured with different frequencies from 50 to 10 000 Hz. As shown in Fig. 2d, the peak temperature is independent of frequency, ruling out the possibility of spin-glass ground state. This supports in turn that the onset of magnetic transition at 4 K is not a spin-glass freezing but a long-range antiferromagnetic ordering.

Fig. 2 (a) Temperature dependences of D_c magnetic reciprocal susceptibility obtained at 0.1 T and (b) the value of χT. (c) Magnetic susceptibilities measured at 0.1 T with zero-field cooling and field-cooling regimes. (d) Ac magnetic susceptibility obtained at different frequencies from 50 to 10 000 Hz.

Fig. 3 shows the isothermal magnetization as a function of applied field (M–H) at 2 K. The magnetization increases nonlinearly with increasing field, agreeing with the appearance of a weak ferromagnetic component in the system. However, no any hysteresis and remnant magnetization are observed near H = 0, while the magnetization do not saturate at the field up to 8 T. To judge spin frustration whether occurs at a magnetic system, an empirical measure has been suggested by defining the value of f = |θ_CW|/T_c, where θ_CW is the Weiss temperature and T_c is an ordering temperature. The value of f > 10 indicates a strong frustration effect in magnetic systems.¹⁸ We note the ordering temperature (T_N) of ∼4 K and the Weiss constant (θ) of ∼64 K in KNa₃Mn₇(PO₄)₆, giving the f value of 16 in the system. This indicates that KNa₃Mn₇(PO₄)₆ has a remarkable spin frustration effect.

Fig. 3 Magnetization as a function of applied field at 2 K.

Here we first investigate whether spin frustration occurs at a magnetic system with a triangle lattice. It is well-known that the Hamiltonian for exchange interaction between any two spins (S₁ and S₂) can be expressed as H = −2JS₁S₂, where the magnitude and the sign of the exchange constant J are determined by the bonding geometry according to the Goodenough rules.¹⁹ As shown in Fig. 4a, while the same magnetic ions occupy in all the sites of an equilateral triangle, the antiferromagnetic (AF) interaction energies between the spins are same. As a result, spin frustration absolutely occurs. Some typical examples for this description can be seen in Kagomé compounds such as Cu₃V₂O₇(OH)₂·2H₂O⁶ and ZnCu₃(OH)₆Cl₂,⁷ showing large spin frustration in the systems. However, if magnetic ions occupy in a non-regular triangle, the situation should be completely different from that of an equilateral triangle. Because the magnitudes of the AF interaction energy between two spins are quite different due to their different exchange constants J arising from different coupling distances. In this case, spin frustration does not completely occur. While magnetic ions occupy in an isosceles triangle, the situation may have two possibilities due to the appearance of two different exchange constants J₁ and J₂. As shown in Fig. 4b, if J₁ is larger than J₂ (J₁ > J₂), spin frustration does not likely occur. The spin arrangements in triangles can be suggested according to the order of lowest energy. As shown in Fig. 4c, if J₁ is smaller than J₂ (J₁ < J₂), spin frustration seems to occur, since the competition of antiferromagnetic and ferromagnetic exchange couplings with same magnitudes should appear in the triangles. As a result, the spin arrangements in one site of triangles cannot be determined.

Fig. 4 The spin arrangements in possible frustrated lattices: (a) equilateral triangle, (b) isosceles triangle with J₁ > J₂, and (c) isosceles triangle with J₁ < J₂. (d) Diamond-like chains built by Mn²⁺ ions of KNa₃Mn₇(PO₄)₆.

Now we discuss the origin of spin frustration in the titled material. As seen in Fig. 4d, spin lattice built by Mn²⁺ ions in the diamond-like chains of KNa₃Mn₇(PO₄)₆ can be described as two types of triangles built by Mn(1)–Mn(3)–Mn(4) and Mn(2)–Mn(3)–Mn(4) running along the a-axis. As shown in Table S3 of ESI,† we note that the distance (3.473 Å) of Mn(3)–Mn(4) is the shortest in the diamond chains, indicating the strongest antiferromagnetic interaction, while the distances of Mn(2)–Mn(3), Mn(1)–Mn(4), Mn(2)–Mn(3), and Mn(2)–Mn(4) are 3.958(4), 3.807(2), 3.866(2), and 3.906(5) Å, respectively. Considering the distances of spin coupling, a possible spin-arrangement is suggested in the diamond-like chains (Fig. 4d). We also note that the distances of Mn(2)–Mn(3) (∼3.906 Å) and Mn(2)–Mn(4) (∼3.866 Å) are quite close, indicating a similar magnitude of the AF interaction energy between them. Thus the triangles built by Mn(2), Mn(3), and Mn(4) sites may be considered as the case of an isosceles triangle in Fig. 4c, in which the antiferromagnetic interaction (J₂) between Mn(3) and Mn(4) ions is likely stronger than those (J₁) of Mn(2)–Mn(3) and Mn(2)–Mn(4). Also, Mn(2) sites are found to connect with Mn(1) sites of the nearest neighboring chains via edge-sharing, forming Mn(1)Mn(2)O₁₀ dimers in the sheets with the distance of 3.232 Å. Thus spin arrangements cannot be determined in the Mn(2) sites due to the spin-frustration arising from the competition of antiferromagnetic and ferromagnetic interactions between Mn(2)–Mn(3) and Mn(2)–Mn(4) routes, which may also affect strongly the spin-arrangements in Mn(1) sites. With decreasing temperature down to 2 K, the spin fluctuation becomes strong compared with thermal fluctuation in the system. As a result, spin freezing of Mn²⁺ ions occurs likely at 4 K and a long-range antimagnetic ordering below 4 K may correspond to the spin-arrangement as suggested in Fig. 4d.

In summary, a new compound KNa₃Mn₇(PO₄)₆ with a diamond chain structure was successfully synthesized by a conventional high-temperature solid-state reaction. Our results confirmed a long-range antiferromagnetic ordering at 4 K with the Weiss constant of ∼64 K, showing the spin-frustration in the system. We noted that there are many nearly isosceles triangles in the diamond-like chains. The spin-frustration in the titled compound was suggested to arise mainly from unsteady spin-arrangements in Mn(2) sites due to the competition of antiferromagnetic and ferromagnetic interactions in such nearly isosceles triangles seen in the diamond-like chains. We suggest that KNa₃Mn₇(PO₄)₆ with a diamond-like chain structure may be considered as a potential geometrically frustrated material.

Acknowledgements

This work was financially supported by the National Basic Research Program of China (no. 2012CB921701) and the NSFC (Grant no. 11074250).

Notes and references

1. J. E. Greedan, J. Mater. Chem., 2001, 11, 37.
2. L. Balents, Nature, 2010, 464, 199.
3. P. W. Anderson, Science, 1987, 235, 1196.
4. S. T. Bramwell, S. G. Carling, C. J. Harding, K. D. M. Harris, B. M. Kariuki, L. Nixon and I. P. Parkin, J. Phys.: Condens. Matter, 1996, 8, L123.
5. A. S. Wills and A. Harrison, J. Chem. Soc., Faraday Trans., 1996, 92, 2161.
6. H. Yoshida, J. Yamaura, M. Isobe, Y. Okamoto, G. J. Nilsen and Z. Hiroi, Nat. Commun., 2012, 3, 860.
7. (a) M. Rigol and R. R. Singh, Phys. Rev. Lett., 2007, 98, 207204; (b) S. H. Lee, H. Kikuchi, Y. Qiu, B. Lake, Q. Huang, K. Habicht and K. Kiefer, Nat. Mater., 2007, 6, 853–857.
8. (a) Y. Okamoto, H. Yoshida and Z. Hiroi, J. Phys. Soc. Jpn., 2009, 78, 033701; (b) D. E. Freedman, R. Chisnell, T. M. McQueen, Y. S. Lee, C. Payen and D. G. Nocera, Chem. Commun., 2012, 48, 64.
9. K. Okamoto1, T. Tonegawa and M. Kaburagi, J. Phys.: Condens. Matter, 2003, 15, 5979.
10. M. Matsuda, K. Kakurai, A. A. Belik, M. Azuma, M. Takano and M. Fujita, Phys. Rev. B: Condens. Matter Mater. Phys., 2005, 71, 144411.
11. M. Fujisawa, J. Yamaura, H. Tanaka, H. Kageyama, Y. Narumi and K. Kindo, J. Phys. Soc. Jpn., 2003, 72, 694.
12. H. Kikuchi, Y. Fujii, M. Chiba, S. Mitsudo, T. Idehara, T. Tonegawa and H. Ohta, Phys. Rev. Lett., 2005, 94, 227201.
13. D. Uematsu and M. Sato, J. Phys. Soc. Jpn., 2007, 76, 084712.
14. H. D. Zhou, E. S. Choi, Y. J. Jo, L. Balicas, J. Lu, L. L. Lumata, R. R. Urbano, P. L. Kuhns, A. P. Reyes, J. S. Brooks, R. Stillwell, S. W. Tozer, C. R. Wiebe, J. Whalen and T. Siegrist, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 82, 054435.
15. Y. Oka, K. Inoue, H. Kumagai and M. Kurmoo, Inorg. Chem., 2013, 52, 2142.
16. M. Kurmoo, K. Otsubo, H. Kitagawa, M. Henry, M. Ohba and S. Takagi, Inorg. Chem., 2010, 49, 9700.
17. W. L. Queen, S. J. Hwu and L. Wang, Angew. Chem., Int. Ed., 2007, 46, 5344.
18. P. Schiffer and A. P. Ramirez, Condens. Matter Phys., 1996, 10, 21.
19. J. Kanamori, J. Phys. Chem. Solids, 1959, 10, 87.

---

Footnote

† Electronic supplementary information (ESI) available: Crystallographic data for KNa₃Mn₇(PO₄)₆: M = 1062.47, orthorhombic, space group C2/c, a = 13.165(8) Å, b = 10.907(6) Å, c = 15.960(1) Å, V = 2106(2) ų, Z = 4, D_c = 3.351 g cm⁻³, Mo Kα, λ = 0.71073 Å, T = 293(2) K, total data 9282, unique data 2034, R₁ = 0.0517, wR₂ = 0.0961 on |F²| and Goof = 0.932. SCXmini diffractometer. The structure was solved by direct methods and all atoms were performed anisotropic refinement by full-matrix least-squares on F² using SHELXTL. CCDC 974986. For ESI and crystallographic data in CIF or other electronic format see DOI: 10.1039/c4ra01122h

---