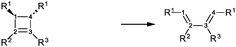On the ring-opening of substituted cyclobutene to benzocyclobutene: analysis of π delocalization, hyperconjugation, and ring strain

Abstract

The influence of several substituents on the ring-opening elementary step of cyclobutene-like systems is analyzed computationally in detail. We focus on trans-1,2-disiloxycyclobutene-like molecules. Electronic effects (hyperconjugation and π delocalization) and geometrical constraints are decoupled and allow for an instructive analysis. It is found that the energy difference between closed and open forms is dictated mainly by the electronic structure of the open form, in which the rotation along the resulting simple C–C bond drives the electronic delocalization. Our calculations led us to quantify effects that determine the energy difference in the special case of disubstituted benzocyclobutene with respect to the disubstituted o-xylylene (aromaticity, π delocalization, ring strain). The relevant role of the siloxy-substituents is rationalized by an analysis of the molecular orbital interaction in an original manner. Finally, calculations are presented and show that the PBE0 functional must be preferred to the popular B3LYP functional for computations on substituted cyclobutene-like rings.

---

1 Introduction

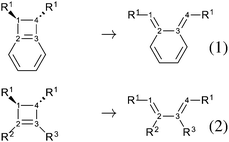
Highly strained molecules are fascinating systems because of their enhanced reactivity.^1–3 Among them, cyclobutene occupies historically a privileged position, since its thermal ring-opening led to the formulation of the famous Woodward and Hoffmann's rules, based on orbital symmetry conservation.⁴ Since then, the concerted conrotatory mechanism has been confirmed as the usual pathway for the thermal ring-opening of cyclobutene and benzocyclobutene, ref. 5 and 6 and references therein, even if it has been shown that it can be modified by mechanical forces.^7–10

Closed forms, i.e. the forms that contain the cyclobutene moiety, are in general less stable than open forms: the 1,3-butene is 11 kcal mol⁻¹ (experimental value)⁷ and 9.9 kcal mol⁻¹ (computed value for the s-cis conformation)⁵ more stable than the cyclobutene. This can be explained by considering the release in ring strain and the possible π electron delocalization in open forms. Quite interestingly, only in the case of the aromatic benzocyclobutenes, closed forms become more stable: the benzocyclobutene is about 13 kcal mol⁻¹ more stable than the o-xylylene (experimental observations).^11,12 Several theoretical studies have been conducted on the ring-opening of cyclobutene-like systems. Nevertheless, the comparison between open and closed forms was rarely the main issue,^13–15 since many studies have been focusing on substitution effects on barrier heights or torquoselectivity.^7,16,17 On the basis of Woodward and Hoffmann's work and of Longuet-Higgins and Abrahamson's study, Houk and coworkers have suggested a diagram that correlates the cyclobutene frontier molecular orbitals with those of the transition state for the ring-opening: the principal ingredients are the occupied π and σ_C–C orbitals, the latter accounts for the C₁–C₄ bond, and the corresponding virtual π* and σ_C–C* orbitals, Fig. 1. This picture helps understanding, for instance, the preference for the outward rotating structure of the 3-aminocyclobutene, which has been attributed to a stabilization due to the interaction between the lone pair and the σ_C–C* orbital in the transition state.¹⁶ This kind of stabilization takes place in general for allylic substituents that carry lone pairs, notably oxygen-based and siloxy.

Fig. 1 Ring opening reactions. If not specified otherwise in the text, R¹ = OTMS.

The present study is a computational work that aims at analyzing the effect of electronic delocalization and aromaticity on the relative stability between open and closed forms in a set of OTMS disubstituted molecules (OTMS = trimethylsilyloxy), as in reactions (1) and (2), Fig. 1. These reactions are elementary steps, the closed form is in the conformation that derives directly from the ring-opening of the cyclobutene moiety (s-cis conformation). Recently, it has been proven that the trans-disiloxybenzocyclobutene combines easily with dioxygen in its triplet state.¹⁸ This opens up the perspective of new, stable molecules capable of catching radical systems. In our previous work, it has been shown that the open form combines easily with dioxygen in its triplet state. The resulting triplet intermediate is crucial, since it undergoes spin orbit coupling, which allows the system to reach the singlet potential energy surface and to evolve in a barrierless process towards the product. In the overall reaction, the relevant parameter is the population of the triplet intermediate, which is directly driven by the amount of open form of the reactant. Thus, we focus on the thermodynamics of the opening reaction, since the reaction barrier of the opening process was crossed under experimental conditions.¹⁸ A spin-catalysis like mechanism could be at work here and lower the opening barrier.¹⁹

Our discussion is based on ΔH_R values, defined as follows:

ΔH_R = H_O − H_C, (3)

where H_C and H_O are the zero point corrected electronic energies of the closed and open forms, respectively. So defined, a positive ΔH_R indicates that the closed isomer is more stable than the open one. This paper is organized as follows: firstly, we report results for various alkylic substitution on OTMS disubstituted cyclobutenes at positions 2 and 3, Fig. 1. By varying R² and R³, several effects are discussed that play a role in the relative stability between closed and open forms (hyperconjugation, π delocalization, aromaticity and ring strain). This will lead us to suggest an energetic decomposition of the ΔH_R obtained in the case of the disubstituted benzocyclobutene. Next, we shall briefly comment on the effect of the allylic substituents on the cyclobutene moiety (R¹) on the ΔH_R values and on the ΔH^#, as well:

ΔH^# = H_TS − H_C, (4)

where H_TS is the zero point corrected electronic energy of the transition state that allows for the conrotatory ring opening.

Last, but not least, we shall present calculations that led us to choose the computational level. Those calculations concern ΔE_R and ΔE^# values of benzocyclobutene (C₈H₆(R¹)₂, R² = R³ = H) and cyclobutene (C₄H₄(R¹)₂, R² = R³ = H), disubstituted on the allylic positions of the cyclobutene, R¹ = H, NH₂, OTMS, CH₃, F, NO₂. Throughout this work, calculations are performed using the PBE0 functional²⁰ (within the frame of the Density Functional Theory methods) and the def2-TZVP basis set.²¹ Experimentally the substituents are OTBS, TBS = tert-butyldimethylsilyl; calculations were performed on OTMS analogous structures to reduce the computational cost, TMS = trimethylsilyl.

2 Results and discussion

Computed values of ΔH_R and ΔG⁰_R = ΔG^298.15 K_R are reported in Table 1. As expected, those values follow the same trend, but ΔG⁰_R values are lower than corresponding ΔH_R values: entropy always favors open forms, because of the release of the cyclobutene ring constraint. This stabilization shifts down all reaction energies by 3 to 5 kcal mol⁻¹. In the following, we shall analyze ΔH_R, whose behavior is driven by the electronic structures of reactants and products.

Table 1 List of the molecules referred to in this work (R¹ = OTMS). ΔH_R (kcal mol⁻¹) is the enthalpy of the ring-opening, ΔG⁰_R (kcal mol⁻¹) is the reaction Gibbs free energy at 298.15 K and τ(°) is the dihedral C₁–C₂–C₃–C₄ angle, Fig. 1

	ΔHR	ΔG^0R	τ(°)
	8.0	4.9	32.3
	−1.8	−6.6	57.2
	−2.4	−4.6	57.1
	−3.3	−7.2	49.8
	−4.1	−6.0	57.6
	−4.1	−7.2	45.9
	−6.4	−7.9	49.2
	−6.3	−10.8	49.1
	−10.2	−12.0	44.7
	−12.5	−15.3	40.2
	−7.7	−12.1	45.6
	−17.2	−21.6	38.6

---

2.1 Influence of electronic delocalization

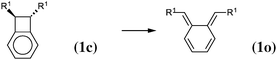
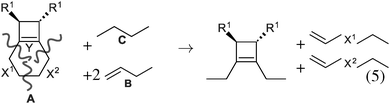
As our goal is to understand deeply the opening reaction of benzocyclobutene, from (1c) to (1o), we first study simpler molecules built in a systematic manner, Table 1. In order to analyze and quantify the effects that play a role in the relative stability between closed and open forms, we consider OTMS cyclobutene derivatives from (2) to (10), where R² and R³ are non-cyclic alkylic substituents. Molecules (11) and (12) are then discussed. Finally, we shall focus on benzocyclobutene. For the cyclic systems, the rationalization of ΔH_R values is supported by computations on hypothetical homodesmotic reactions, as defined by Houk and coworkers.²² Those reactions give access to energy differences that are not bond breaking and creation related, such as strain or aromaticity.

2.1.1 Non-cyclic R² and R³. From (10) to (1), closed forms become more and more stable compared to the open structures. We shall see that relevant effects are variations in hyperconjugation ((10) → (6)) and/or π delocalization ((5) → (1)). Molecule (10), for which R² = R³ = H, exhibits a ΔH_R of −12.5 kcal mol⁻¹. The replacement of one H by a methyl changes this value to −10.2 kcal mol⁻¹ for (9). Similarly, a drop of 3.9 kcal mol⁻¹ is observed, when a second methyl is introduced in (8). Replacing a methyl by an ethyl does not significantly change the energy difference (−6.8 kcal mol⁻¹ in (7)), but the replacement of the second methyl leads to a drop of further 2.6 kcal mol⁻¹ of the energy difference for (6). These energetic values are related to a larger stabilization of the closed structures with respect to the open forms by hyperconjugation: the appropriate combination of C–H σ orbitals interacts with the π system and lowers the total energy.²³ This stabilization does not occur as efficiently in the open form due to the large value of the τ = τ_C1C2C3C4 torsion angle: the interaction between the C–H σ and the π orbitals decreases when τ increases, thus the stabilization is less important. Evidence of hyperconjugation is also detectable in the C₂–R² and C₃–R³ distances, which are consistently 2 pm shorter in closed forms (about 148 pm) than in open forms (about 150 pm). This shortening is of the order of what is found for simpler similar systems.²⁴

In the closed form, because of the constraint due to the 4-membered ring, π delocalization can be efficient. In the open form, mesomery of π electrons along the C₁–C₂–C₃–C₄ backbone is favored when the torsion angle τ is low. The change from ethyl to vinyl (7) to (5) modifies again significantly the ΔH_R values by 2.7 kcal mol⁻¹. Now the effect is pure resonance due to the delocalization of the π electrons. It is worth keeping in mind that the open form has always two π electrons more than the closed form. The closed form (5c) is efficiently stabilized by mesomery: the p orbitals of the carbon atoms are orthogonal to the 4-membered ring, which leads to nice π delocalization of the four π electrons. In the open form (5o), there are six π electrons but τ is large (57.6°). This leads to two distinct π systems of 4 and 2 electrons, which repel each other because of electron pair repulsion. It is this repulsion that destabilizes the open form with respect to the closed one. Analysis of geometrical parameters corroborates the fact that π delocalization occurs better in the closed form than in the open one: the C₂–C₃ distance is equal to 148.0 pm in (5o), i.e. 4 pm longer than C₂–R². Thus, the π delocalization along the C₂–C₃ bond is weak. The same holds true from (5) to (2). The larger number of π electrons to delocalize in (2) stabilizes further the closed form with respect to the open form and ΔH_R approaches zero.¶

In summary, hyperconjugation and π electronic delocalization are the key phenomena which explain the relative stability of the closed and open forms of the non-cyclic molecules (2) to (10). If R² and R³ carry n₂ and n₃ π electrons, respectively, then the total number of π electrons is n₂ + n₃ + 2 in the closed form. As τ is constraint to values close to zero, the π system involves all these electrons through the C₂=C₃ bond and the stabilization is due to hyperconjugation and delocalization. In the open form, the breaking of C₁–C₄ releases the geometrical constraint on τ. The substituents R² and R³ lead to two distinct π systems with n₂ + 2 and n₃ + 2 electrons, respectively, which possibly repel each other. The stability of the open form is due to a balance between π delocalization together with ring strain release and repulsion between π systems together with the breaking of the C₁–C₄ single bond.

2.1.2 Molecules with fused rings. What happens when R² and R³ are linked, forming a cycle? In those cases, rotations around the C₂ and C₃ bonds in the open forms are limited, steric repulsion cannot be avoided, leading to strain in the cycle. Notably, the ΔH_R value of (12) is quite large, when compared to non-cyclic analogues (4) or (5). In order to identify the key parameters of those reactions, we computed the two reaction energies of the 6-membered ring opening before and after the opening of the cyclobutene moiety by a procedure detailed in Fig. 2. The so-obtained homodesmotic reactions give access to the 6-membered ring strains and are then connected by the two cyclobutene ring-opening reactions. The resulting Hess cycles for (11) and (12) are presented in Fig. 3 and 4. Reaction (6) reveals that the 6-membered ring in (11c) is not too strained, as expected for a cyclohexene-like structure;²⁵ nevertheless this ring strain is present and accounts for 2.8 kcal mol⁻¹. For reaction (7), the 6-membered ring is now similar to a cyclohexane and the calculation leads to a strain energy of zero. Thus, the opening of the cyclobutene moiety from (11c) to (11o) is slightly more exothermic than the one from (6c) to (6o) because of the release of the 6-membered ring strain in (11o).

Fig. 2 A homodesmotic reaction can be built as follows. The reactant A is cut into three parts (X¹, X² and Y). Fragment X¹ is obtained by cleavage of one C_sp³–C_sp² and one C_sp³–C_sp³ bond. It is thus inserted between the single and the double bond of B. The same procedure applies to X² and Y and determines the nature of the other reactants. In the current case, one B is needed to insert X² and one C to insert Y. This fully defines the left and right hand side of the arrow. This procedure ensures that the same number of each kind of bond is on each side of the reaction arrow.

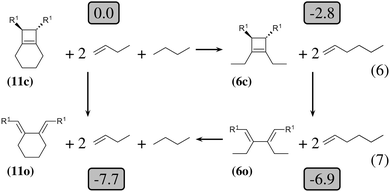Fig. 3 Thermodynamic cycle which decomposes the opening of (11c) into three steps. Relative energies in kcal mol⁻¹ are indicated in gray boxes for R¹ = OTMS. Reactions (6) and (7) are homodesmotic.

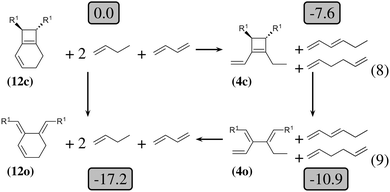Fig. 4 Thermodynamic cycle which decomposes the opening of (12c) into three steps. Relative energies in kcal mol⁻¹ are indicated in gray boxes for R¹ = OTMS. Reactions (8) and (9) are homodesmotic.

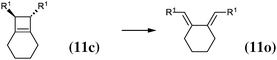
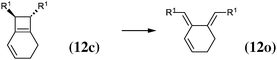
The 4-membered ring-opening of (12c) is 9.5 kcal mol⁻¹ more favorable than the same opening for (11c). To understand the reason for this difference, we decompose the transformation into three steps. The first step, reaction (8), releases 7.6 kcal mol⁻¹, which is the ring strain energy of the 6-membered ring of (12c). It is larger than the corresponding strain energy of (11c) because of the lack of flexibility due to the sp² carbon atoms. The opening of the cyclobutene moiety from (4c) to (4o) is 3.3 kcal mol⁻¹ exothermic, which is similar to the opening of the cyclobutene moiety from (6c) to (6o) (4.1 kcal mol⁻¹). Quite surprisingly, the closing of the 6-membered ring, reaction (9), is 6.3 kcal mol⁻¹exothermic: the ring constraint in (12o) leads to a more stable molecule than (4o). Indeed, the cyclic constraint imposes a τ value of 38.6°: this is the lowest value in the set of molecules in Table 1, with the exception of the aromatic (1c). In (12o), the π electronic delocalization is favored because of this low τ value, thus the 17.2 kcal mol⁻¹ exothermicity of the opening of the cyclobutene moiety in (12) comes from the stabilization energy due to π delocalization in (12o), induced by the 6-membered ring constraint.

2.1.3 The benzocyclobutene case. Finally, let us discuss the case of the benzocyclobutene, for which the closed form is the most stable. Following the aromaticity criterion of Julg and François based on the alternation of the long/short bonds,²⁶ the (1c) molecule is aromatic whereas (1o) is not (geometrical parameters are provided in the ESI‡). We shall now quantify energetic effects due to the loss of aromaticity and, in general, variation in the π system. To do that, we suggest a decomposition of the ΔH_R value according to the following Hess cycle that has been computed for R¹ = OTMS and R¹ = H, Fig. 5. Furthermore, a homodesmotic reaction for benzene alone can also be constructed, Fig. 6.

Fig. 5 Thermodynamic cycle which decomposes the opening of (1c) into three steps. Relative energies in kcal mol⁻¹ are indicated in gray boxes for R¹ = OTMS and R¹ = H. Reactions (10) and (11) are homodesmotic.

Fig. 6 Homodesmotic opening reaction of benzene. Relative energies in kcal mol⁻¹ are indicated in gray boxes.

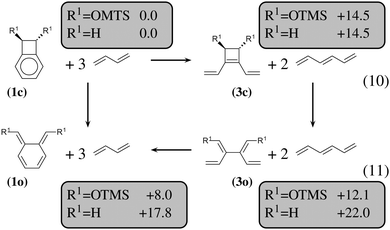
The most obvious result is that OTMS substituents do not have any influence on the energetics of the 6-membered ring-opening: the reaction energies of (10), which lead from (1c) to (3c), are of 14.5 kcal mol⁻¹, and the reaction energies of (11), which lead from (3o) to (1o), are of about −4 kcal mol⁻¹, both for R¹ = H and R¹ = OTMS. Nevertheless, the OTMS substituents have a large influence on the ring-opening of the cyclobutene moiety, of about 10 kcal mol⁻¹. The effect is so strong that the relative stability of (3c) vs. (3o) is inverted for R¹ = H (the closed form is the most stable) with respect to R¹ = OTMS (the open form is the most stable).

We shall now analyze the energetic contributions of the reactions in the Hess cycle. According to the IUPAC definition,²³ the reaction energy of (10) and (12) equals the aromaticity loss. For benzocyclobutene (substituted or not), this loss accounts for 14.5 kcal mol⁻¹, while the opening of the benzene ring leads to an endothermicity of 20.2 kcal mol⁻¹, reaction (12). The 5.7 kcal mol⁻¹ difference corresponds to a partial loss of aromaticity in (1c), due to the geometrical constraint brought by the cyclobutene moiety (ring strain energy of the 6-membered ring in benzocyclobutene).

For reaction (11), from (3o) to (1o), an energy gain of about 4 kcal mol⁻¹ is computed. There are eight π electrons in (3o) and in (1o). For (3o), the delocalization involves two π systems of four electrons each, with a large τ (57°, OTMS-disubstituted case) as described in the previous subsection. For (1o), the delocalization involves all the eight electrons, since it occurs mainly via the cycle rather than through the C₂–C₃ bond. It is, therefore, much more efficient.

In conclusion, the energetic decomposition of the ΔH_R values is the following: in the OTMS-disubstituted case, ΔH_R is of 8 kcal mol⁻¹. The breaking of the aromaticity implies an energy loss of 14.5 kcal mol⁻¹. The 6.5 kcal mol⁻¹ that needs to be recovered are due to two contributions: the opening of the cyclobutene moiety releases 2.4 kcal mol⁻¹; secondly, the efficient electron delocalization in (1o) accounts for the remaining 4.1 kcal mol⁻¹. A similar picture can be drawn for the non-substituted case. Here, ΔH_R is of 17.8 kcal mol⁻¹ and the only difference with respect to the previous picture lies in the energetic demanding opening (7.5 kcal mol⁻¹) of the cyclobutene moiety.

2.2 Substitution on cyclobutene allylic positions

In the previous section, we have pointed out that OTMS substitutes play an important role in the ring-opening of the cyclobutene moiety. As reminded in the Introduction, effects of substitution on allylic positions of the cyclobutene have been widely studied. Here we disclose a further aspect that affects ΔH_R and ΔH^# values and that has not been clearly reported so far, to the best of our knowledge.

We consider disubstituted cyclobutenes and benzocyclobutenes, where R¹ = H, OTMS, NH₂, the substituents carry lone pairs. The ΔH_R and ΔH^# values are collected in Table 2. The SCS-MP2²⁷ reference values are also indicated. The discussion is based on the PBE0 values, but the SCS-MP2 values have been reported, because effects are slightly too pronounced at the PBE0 level, even if trends are preserved.

Table 2 PBE0 and SCS-MP2 ΔH_R and ΔH^# (kcal mol⁻¹) values for C₄H₄(R¹)₂ and C₈H₆(R¹)₂ systems. SCS-MP2 values are single points on the PBE0 structures plus the PBE0 zero point energies. The basis set is of def2-TZVP quality

	PBE0		SCS-MP2
R^1	ΔHR	ΔH^#	ΔHR	ΔH^#
a Experimental values: ΔHR = 13.3; ΔH^# = 40.0.^11,12
C4H4(R^1)2
H	−5.8	35.3	−8.6	34.6
NH2	−17.7	14.6	−15.2	17.3
OTMS	−12.5	19.1	−10.2	21.1
C8H6(R^1)2^a
H	17.8	43.1	14.2	40.7
NH2	5.4	20.3	7.8	21.9
OTMS	8.0	24.6	7.2	23.1

---

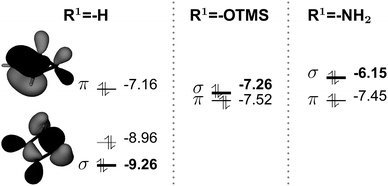
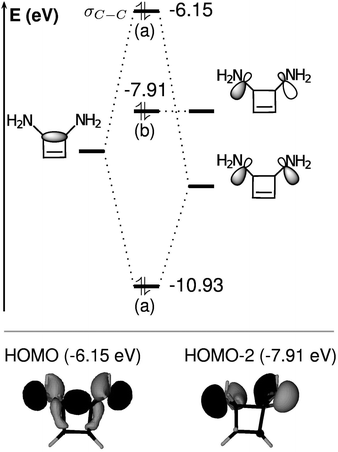
The destabilization of the closed forms with respect to the transition states and the open forms for substituted cases correlates well with the highest occupied molecular orbital pictures in Fig. 7. We recall that crucial orbitals in the closed form are those that correspond to bonds that need to be broken: the π and π* orbitals between C₂ and C₃, and the σ_C–C and σ_C–C* orbitals that can be ascribed to the C₁–C₄ bond.

Fig. 7 Highest occupied molecular orbitals for cyclobutene cases (energies in eV at the PBE0/def2-TZVP level, R¹ = H, OTMS, NH₂). σ stands for the molecular orbital that corresponds to the C₁–C₄ bond (in bold). Represented molecular orbital densities (contour ±0.05 a.u.) are for the cyclobutene π and the σ orbital. For cyclobutene, the σ orbital is the HOMO − 2, while for disubstituted cases the σ orbital is the HOMO.

For R¹ = H, the HOMO is the π orbital, but the σ_C–C orbital is the HOMO − 2 and relatively low in energy. For R¹ = OTMS, NH₂, the presence of the substituents does not significantly perturb the π system. Nevertheless their action is relevant to the σ_C–C orbital: its energy raises substantially and it becomes the new HOMO, with an energy close to that of the π orbital. This results in a weakening of the C₁–C₄ bond. As a consequence, the barrier height decreases and the closed form is destabilized with respect to the open form. The open form is further stabilized by the contribution of the substituents to the delocalized π system.

The raise in energy of the σ_C–C orbital finds its origin in the interaction with the two lone pairs on the substituents: this interaction leads to three molecular orbitals, as represented in Fig. 8 for cyclobutene and R¹ = NH₂. The most interesting outcome is that the most energetic orbital becomes the σ_C–C for the substituted cases.

Fig. 8 Schematic representation of the interaction between lone pairs and the σ_C–C orbital, in the disubstituted cyclobutene R¹ = NH₂. Symmetry labels are given in parentheses in the C₂ point group of symmetry. The HOMO and HOMO − 2 orbitals are represented below the orbital interaction diagram. The HOMO (−6.15 eV) is the σ_C–C bond, the HOMO − 2 (−7.91 eV) is localized on the N atoms and the lowest energetic orbital is delocalized on the cycle. Energies are in eV (PBE0/def2-TZVP level). The energy of the not represented π orbital (the HOMO − 1) is of −7.45 eV. Energies before interaction are arbitrary.

To summarize, several factors that intervene in the ring-opening of cyclobutene derivatives have been already discussed elsewhere,^7,15–17 here we emphasize a further aspect, the weakening of the C₁–C₄ bond upon disubstitution on cyclobutene allylic positions by R¹ = OTMS, NH₂. This contributes to lower barrier heights and, in general, to destabilize closed forms.

2.3 Computational details

From a theoretical point of view, the functional B3LYP^28–33 has been widely employed to study electrocyclic reactions of cyclobutene-like systems and its validity has been carefully tested with respect to hydrocarbon pericyclic reactions.³⁴ Nevertheless, this type of benchmarking does not guarantee that a DFT functional maintains the same performances when substituents are introduced that perturb deeply the electronic structure of a molecule, such as OTMS. Thus, calculations on allylic substituted cyclobutene C₈H₆(R¹)₂ and benzocyclobutene C₄H₄(R¹)₂ have been employed to compare results from DFT methods to highly accurate CCSD(T) and SCS-MP2 values.

Calculations at the CCSD(T) level were performed with the MOLPRO program package³⁵ and give access to SCS-MP2 energies, as well. Otherwise, DFT (B3LYP, PBE,^28,29,36,37 and PBE0) and additional SCS-MP2 calculations were performed with the program package TURBOMOLE.³⁸ The def2-TZVP basis set was employed, unless specified. Structures were fully optimized at each DFT level. The ab initio calculations (SCS-MP2 and CCSD(T)) are obtained as single point energy calculations on PBE0 geometries. The Resolution of Identity approximation was employed.^39–43 Comparison among methods is based on ΔE_R and ΔE^# values, which do not contain zero-point energies. We do not have here the ambition to provide an extensive benchmark, but our aim is to investigate some specific cyclobutene derivatives, thus we have restricted our preliminary methodological analysis to a small set of similar molecules, R¹ = H, NH₂, OTMS, CH₃, F, NO₂. For the conciseness sake, we shall discuss results in terms of average errors (ae), mean absolute errors (mae), and maximum errors (max), details are reported in the ESI.‡ We employed the following scheme:

(i) the reliability of the CCSD(T) and SCS-MP2 methods has been checked with respect to the basis set choice. Thus, reference ΔE_R and ΔE^# values of the C₄H₆ and C₄H₄F₂ systems have been computed at the complete basis set (cbs) CCSD(T) level, the cbs extrapolation scheme uses cc-pVTZ, cc-pVQZ and cc-pV5Z basis sets.^44–46 Results are reported in Table 3 and show that deviations for SCS-MP2 values are negligible (less than 1.5 kcal mol⁻¹) and that ΔE_R and ΔE^# have already converged with a def2-TZVP basis set (both for SCS-MP2 and CCSD(T) calculations).

Table 3 Computed ΔE_R and ΔE^# (kcal mol⁻¹, in bold) for C₄H₆ and C₄H₄F₂ at the CCSD(T)/cbs (cbs = complete basis set, as explained in Section 2.3). Deviations from those values follow for SCS-MP2 and CCSD(T) employing the def2-QZVP and def2-TZVP basis sets (kcal mol⁻¹). SCS-MP2 and CCSD(T) values are from single point calculations on PBE0 structures

Method	Basis set	C4H6		C4H4F2
		ΔER	ΔE^#	ΔER	ΔE^#
a Extrapolation from cc-pVTZ, cc-pVQZ, cc-pV5Z basis sets. b Experimental ΔH^# = 32.5 ± 0.5 kcal mol^−1.^7
CCSD(T)	cbs^a	−8.12	35.17	−3.98	27.74
	def2-QZVP	−0.3	−0.2	−0.3	−0.2
	def2-TZVP	−0.4	−0.4	−0.6	−0.5
SCS-MP2	def2-QZVP	0.5	1.2	0.4	0.7
	def2-TZVP	0.4	1.1	0.2	0.6

---

(ii) SCS-MP2 and DFT values of substituted cyclobutene C₄H₄(R¹)₂ have been compared to those at the CCSD(T) level, Table 4. Here, again, SCS-MP2 deviations from CCSD(T) results are within 1 kcal mol⁻¹, which confirms that SCS-MP2 provides reliable reference data for those systems.

Table 4 Deviations from reference ΔE_R and ΔE^# values for several methods. Treated systems are C₄H₄(R¹)₂ and C₈H₆(R¹)₂, where R¹ = H, NH₂, OTMS, CH₃, F, NO₂. Average errors (ae), mean absolute errors (mae), and maximum deviations (max) are reported (kcal mol⁻¹). The reference calculations are at the CCSD(T) level for C₄H₄(R¹)₂ systems and at the SCS-MP2 level for C₈H₆(R¹)₂ systems. Structures are optimized at each DFT method. SCS-MP2 and CCSD(T) values are from single point calculations on PBE0 structures. The basis set is of def2-TZVP quality

R^1	PBE0	PBE	B3LYP	SCS-MP2
a R^1 = H. b R^1 = NH2. c R^1 = OTMS.
C4H4(R^1)2
ΔER
ae	0.1	−2.6	−6.2	0.5
mae	1.7	3.2	6.2	0.5
max	3.7^a	−5.6^b	−8.3^c	0.9
ΔE^#
ae	−0.5	−6.0	−3.8	0.6
mae	1.3	6.0	3.8	0.6
max	−2.8	−8.3	−5.6	1.5
C8H6(R^1)2
ΔER
ae	0.4	−4.5	−6.0
mae	1.5	4.6	6.0
max	3.6^a	−8.0^b	−8.0^b
ΔE^#
ae	0.6	−6.7	−3.7
mae	1.3	6.7	3.7
max	2.4	−8.8	−5.4

---

(iii) DFT calculations for substituted benzocyclobutene C₈H₆(R¹)₂ have been consequently compared to SCS-MP2 only, Table 4.

Let us consider first DFT performances for ΔE^# values: as expected, the PBE functional underestimates reaction barriers by about 6–7 kcal mol⁻¹. Results for B3LYP and PBE0 are significantly better, the mean average errors are of 3.8 and 1.3 kcal mol⁻¹, respectively.

Let us now consider ΔE_R values. The PBE0 functional behaves quite well, mean average errors are less than 2 kcal mol⁻¹ and the largest error is for non-substituted cases (R = H: 3.7 kcal mol⁻¹ for cyclobutene and 3.6 kcal mol⁻¹ for benzocyclobutene, Table 2). PBE and B3LYP show larger deviations from our reference calculations. The B3LYP functional performs the worst and underestimates the ΔE_R values, thus suggesting open forms much too stable with respect to closed forms. Errors are not negligible, notably for disubstituted benzocyclobutene, R¹ = OTMS, NH₂. For those cases, PBE and B3LYP ΔE_R results are misleading, since they suggest that open and closed forms are almost degenerate, while the equilibrium is clearly displaced towards closed structures.

We conclude that, even if barrier heights are well reproduced, the validity of B3LYP is questionable for cyclobutene-like systems, when substitutions on allylic positions perturb their electronic structure. In the present work, since we largely focus on OTMS disubstituted systems, we have chosen to present PBE0 results that provide a better performance with respect to our reference calculations.

3 Conclusions

In this work we have suggested an analysis of key parameters that determine the relative stability between closed and open forms of OTMS disubstituted cyclobutene derivatives, where the open forms are in the conformations that derive directly from the cyclobutene ring-opening elementary step.

The analysis of the relative stability of closed and open forms for a set of molecules shows that the nature of R² and R³ plays a decisive role. The cyclobutene ring imposes a geometrical constraint that leads to a frustration (the ring strain), but allows for efficient hyperconjugation (systems (10c) → (6c)) and π delocalization around C₂–C₃ (systems (5c) → (2c)). The relative stability of the open form is determined by an equilibrium of several factors, notably the C₄ strain release, the repulsion between R² and R³, and the π delocalization along C₂–C₃ that is driven by the dihedral angle τ = τ_C1C2C3C4. Thus, electronic effects due to R² and R³ differ in the open and closed form, which explains the evolution of ΔH_R in the set of molecules studied.

When R² and R³ are bound, leading to cyclic structures, strain energy plays a significant role in the relative stability of the open and closed forms. The balance between energy strain and π electronic delocalization leads to large variations in ΔH_R. For benzocyclobutene, the closed form is more stable. This is related to the loss of the aromaticity, which accounts for 14 kcal mol⁻¹. Even if this energetic loss is tempered by the constraint on the benzene due to the cyclobutene, the release of the strain of the cyclobutene and the efficient π delocalization through the C₆ cycle in the o-xylylene are not sufficient to compensate it.

Finally, substitution on allylic positions of the cyclobutene by R = OTMS, NH₂ impacts and weakens the C₁–C₄ bond in the closed form, lowering significantly ΔH^# values with respect to R = H and destabilizing the closed form with respect to the open form. As mentioned in the Introduction, the trans-disiloxybenzocyclobutene is active towards radical species. Since in this kind of reaction, the rate limiting step is the ring-opening of the cyclobutene moiety, the amino-disubstituted benzocyclobutene is a potential candidate, which performs as good as or even better than the OTBS analogues.

Acknowledgements

We thank Pr. Maurice Santelli, Pr. Stéphane Humbel and Dr Laurent Commeiras for fruitful discussions. This work is dedicated to Guillaume Chailly, a dearest friend.

References

1. M. R. Wilson and R. E. Taylor, Angew. Chem., Int. Ed., 2013, 52, 4078–4087.
2. F. Schoenebeck, D. H. Ess, G. O. Jones and K. N. Houk, J. Am. Chem. Soc., 2009, 131, 8121–8133.
3. W. J. van Zeist and F. M. Bickelhaupt, Org. Biomol. Chem., 2010, 8, 3118–3127.
4. R. B. Woodward and R. Hoffmann, Angew. Chem., Int. Ed. Engl., 1969, 8, 781–853.
5. S. Sakai, J. Phys. Chem. A, 2000, 104, 11615–11621.
6. D. C. Spellmeyer and K. N. Houk, J. Am. Chem. Soc., 1988, 110, 3412–3416.
7. P. Lee, S. Sakai, P. Horstermann, W. Roth, E. Kallel and K. Houk, J. Am. Chem. Soc., 2003, 125, 5839–5848.
8. C. R. Hickenboth, J. S. Moore, S. R. White, N. R. Sottos, J. Baudry and S. R. Wilson, Nature, 2007, 446, 423–427.
9. J. Friedrichs, M. Luessmann and I. Frank, ChemPhysChem, 2010, 11, 3339–3342.
10. J. Ribas-Arino, M. Shiga and D. Marx, Chem. – Eur. J., 2009, 15, 13331–13335.
11. W. R. Roth, M. Biermann, H. Dekker, R. Jochems, C. Mosselmann and H. Hermann, Chem. Ber., 1978, 111, 3892–3903.
12. W. R. Roth and B. P. Scholz, Chem. Ber., 1981, 114, 3741–3750.
13. A. G. Csàszàr, J. Phys. Chem. A, 2004, 108, 2002–2007.
14. L. Lo Presti, A. Ellern, R. Destro, R. Soave and B. Lunelli, J. Phys. Chem. A, 2011, 115, 12695–12707.
15. N. Mariet, H. Pellissier, J.-L. Parrain and M. Santelli, Tetrahedron, 2004, 60, 2829–2835.
16. P. Lee, X. Zhang and K. Houk, J. Am. Chem. Soc., 2003, 125, 5072–5079.
17. M. Murakami, M. Hasegawa and H. Igawa, J. Org. Chem., 2004, 69, 587–590.
18. J. Drujon, R. Rahmani, V. Héran, R. Blanc, Y. Carissan, B. Tuccio, L. Commeiras and J.-L. Parrain, Phys. Chem. Chem. Phys., 2014, 16, 7513–7520.
19. O. Plachkevytch, B. Minaev and H. Ågren, J. Phys. Chem., 1996, 100, 8308–8315.
20. J. Perdew, M. Emzerhof and K. Burke, J. Chem. Phys., 1996, 105, 9982–9985.
21. F. Weigend and R. Ahlrichs, Phys. Chem. Chem. Phys., 2005, 7, 3297–3305.
22. S. E. Wheeler, K. N. Houk, P. V. R. Schleyer and W. D. Allen, J. Am. Chem. Soc., 2009, 131, 2547–2560.
23. A. D. McNaught and A. Wilkinson, IUPAC. Compendium of Chemical Terminology, 2nd edn, (the “Gold Book”), XML on-line corrected version: http://goldbook.iupac.org (2006) created by M. Nic, J. Jirat, B. Kosata; updates compiled by A. Jenkins., Blackwell Scientific Publications, Oxford, 1997.
24. I. Fernàndez and G. Frenking, Chem. – Eur. J., 2006, 12, 3617.
25. F. Liu, R. S. Paton, S. Kim, Y. Liang and K. N. Houk, J. Am. Chem. Soc., 2013, 135, 15642–15649.
26. A. Julg and P. François, Theor. Chim. Acta, 1967, 8, 249–259.
27. S. Grimme, J. Chem. Phys., 2003, 118, 9095–9102.
28. P. A. M. Dirac, Proc. R. Soc. London, Ser. A, 1929, 123, 714–733.
29. J. C. Slater, Phys. Rev., 1951, 81, 385–390.
30. S. Vosko, L. Wilk and M. Nussair, Can. J. Phys., 1980, 58, 1200.
31. A. D. Becke, Phys. Rev. A: At., Mol., Opt. Phys., 1988, 38, 3098–3100.
32. A. D. Becke, J. Chem. Phys., 1993, 98, 5648–5652.
33. C. Lee, W. Yang and R. G. Parr, Phys. Rev. B: Condens. Matter Mater. Phys., 1988, 37, 785.
34. V. Guner, K. Khuong, A. Leach, P. Lee, M. Bartberger and K. Houk, J. Phys. Chem. A, 2003, 107, 11445–11459.
35. H.-J. Werner, P. J. Knowles, G. Knizia, F. R. Manby, M. Schütz, P. Celani, T. Korona, R. Lindh, A. Mitrushenkov, G. Rauhut, K. R. Shamasundar, T. B. Adler, R. D. Amos, A. Bernhardsson, A. Berning, D. L. Cooper, M. J. O. Deegan, A. J. Dobbyn, F. Eckert, E. Goll, C. Hampel, A. Hesselmann, G. Hetzer, T. Hrenar, G. Jansen, C. Köppl, Y. Liu, A. W. Lloyd, R. A. Mata, A. J. May, S. J. McNicholas, W. Meyer, M. E. Mura, A. Nicklass, D. P. O'Neill, P. Palmieri, K. Pflüger, R. Pitzer, M. Reiher, T. Shiozaki, H. Stoll, A. J. Stone, R. Tarroni, T. Thorsteinsson, M. Wang and A. Wolf, MOLPRO, version 2006.1, a package of ab initio programs, 2006.
36. J. Perdew and Y. Wang, Phys. Rev. B: Condens. Matter Mater. Phys., 1992, 45, 13244–13249.
37. J. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865–3868.
38. TURBOMOLE V6.5 2013, a development of University of Karlsruhe and Forschungszentrum Karlsruhe GmbH, 1989–2007, TURBOMOLE GmbH, since 2007, available from http://www.turbomole.com.
39. K. Eichkorn, O. Treutler, H. Öhm, M. Häser and R. Ahlrichs, Chem. Phys. Lett., 1995, 242, 652–660.
40. K. Eichkorn, F. Weigend, O. Treutler and R. Ahlrichs, Theor. Chem. Acc., 1997, 97, 119–124.
41. F. Weigend, Phys. Chem. Chem. Phys., 2006, 8, 1057–1065.
42. C. Hättig and F. Weigend, J. Chem. Phys., 2000, 113, 5154–5161.
43. F. Weigend, A. Köhn and C. Hättig, J. Chem. Phys., 2001, 116, 3175–3183.
44. T. H. Dunning, J. Chem. Phys., 1989, 90, 1007–1023.
45. R. A. Kendall, T. H. Dunning and R. J. Harrison, J. Chem. Phys., 1992, 96, 6796–6806.
46. A. Halkier, T. Helgaker, P. Jørgensen, W. Klopper, H. Koch, J. Olsen and A. K. Wilson, Chem. Phys. Lett., 1998, 286, 243–252.

---

Footnotes

† This work is dedicated to Guillaume Chailly.

‡ Electronic supplementary information (ESI) available. See DOI: 10.1039/c4cp01695e

§ These authors contributed equally to this work.

¶ For (2), |ΔE_R| is smaller than the accuracy of the theoretical method used: the mean absolute error is found to be 1.7 kcal mol⁻¹ (see Section 2.3).

---