The structure of the melamine–cyanuric acid co-crystal

Abstract

The crystal structure of the melamine:cyanuric acid (CA.M) adduct has been redetermined and the previously reported cell is shown to be incorrect. The true unit cell has approximately twice the volume of the earlier cell. Crystal data for CA.M: monoclinic, space group I2/m, a = 14.8152(19) Å, b = 9.6353(18) Å, c = 7.0405(9) Å, β = 93.194(11)°, V = 1003.5(3) ų, Z = 2. In contrast to the previous report, this contains an ordered array of cyanuric acid and melamine. Hydrogen bonding between the two components is described in detail and precise information about intermolecular distances reported for the first time. The structure contains hydrogen-bonded sheets stacked perpendicular to the crystallographic (101) plane. The molecular geometry of the cyanuric acid and melamine components is described in detail. Possible explanations for the difference between this structure and the previous report are described in the light of quantum chemical calculations.

---

Introduction

Co-crystals of melamine–cyanuric acid (CA.M) (Scheme 1) have attracted enormous attention for a variety of reasons. This compound is commercially important, potentially hazardous to health, and yet a shining example of non-covalent synthesis. CA.M has been examined as a solid lubricant,¹ and as a fire retardant,^2,3 and for other patented uses (opacifier, flame suppressant).^4,5CA.M forms the basis of the ‘disappearing spot test’ for cyanuric acid widely used in swimming pools;⁶ in which a dark spot is covered by precipitation of white opaque CA.M.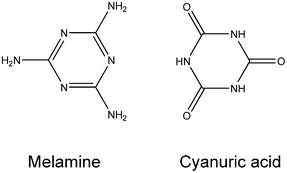

Scheme 1 Molecular components of the melamine–cyanuric acid co-crystal.

Within the body, melamine is metabolised to cyanuric acid, a process that leads to the precipitation of co-crystals of CA.M within the kidneys, causing renal failure. The toxicity has been the subject of considerable public interest following numerous incidences of unscrupulous suppliers including melamine in products such as wheat or baby milk to increase the apparent protein content.^7,8 Very recently there have been reports of further incidents of this type in the developing world.⁹ It is notable that the crystal morphology of CA.M has been reported to be different for samples produced in vitro from those produced in vivo.¹⁰ This is worthy of further study. There has been a very pronounced research effect to develop effective, sensitive, techniques for the detection of melamine in foodstuffs. Methods have included colorimetry employing gold nanoparticles,¹¹ mass spectrometry,^12,13 electrophoresis,¹⁴ and electrochemical methods.^15,16

Prior to the determination of the crystal structure of CA.M, the complementary hydrogen bonding proposed within CA.M, the so-called ‘cyanuric acid–melamine lattice’, was used as the basis of wide variety of supramolecular assemblies.^17,18 Since the first report of the crystal structure of melamine–cyanuric acid co-crystals in 1999,¹⁹ this compound has been a flagship for the rational design of solids through complementary hydrogen-bonding interactions. The original report has been very highly cited and the structure used to illustrate the concept of crystal engineering.^20–22 The complementarity of the melamine and cyanuric acid units has been used to rationalise the stability and relatively poor aqueous solubility of the co-crystal. This model has been used as the basis of elegant studies of CA.M crystal growth²³ and studies of CA.M under pressure.²⁴

However, the crystal structure of the melamine–cyanuric acid adduct (CA.M) in space group C2/m reported previously¹⁹ (CCDC²⁵ Refcode QACSUI) does not contain an ordered array of melamine and cyanuric acid. The asymmetric unit of QACSUI, shown in Fig. 1, contains one half of a hexagonal molecule. This model implies that the melamine and cyanuric acid molecules are disordered within the structure, a situation that is at odds with the proposed hydrogen bonding present which necessitates strict ordering of the hydrogen-bond donor and acceptor molecules. The situation also implies that the molecular geometry of the two six-membered rings is indistinguishable. We believed this model to be incorrect and sought to determine the true crystal structure of the CA.M adduct and thereby obtain precise bond lengths and angles for the molecules present, and perhaps more importantly, obtain precise intermolecular distances to shed light on the hydrogen bonding present.

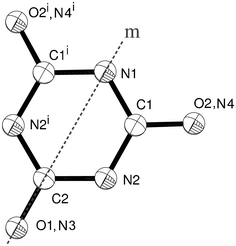Fig. 1 The disordered melamine/cyanuric acid molecule within QACSUI. Atoms are drawn as 50% probability spheres. Symmetry equivalent atoms are generated by the mirror plane labelled m, namely symmetry operator i = x, −y, z.

Experimental

Synthesis

In line with the previous report, crystals of CA.M were obtained by hydrothermal techniques, employing melamine as the starting material. Under the acidic conditions of the reaction, melamine is hydrolysed to cyanuric acid. 0.2522 g melamine (2.00 mmol) and 0.3459 g of phosphoric acid, (H₃PO₄ 85 wt% in H₂O) (3.00 mmol) were added to 10 mL of deionized water with catalytic iron(II) chloride tetrahydrate (0.1988 g, 1.00 mmol). The mixture was stirred for 15 minutes in a 23 mL Teflon vessel. The Teflon-liner was placed within a stainless steel autoclave, sealed and heated in an oven at 170 °C for 48 h. This was allowed to cool radiatively to room temperature. Once cool, the product was filtered and washed with distilled water and dried at 85 °C in air.

Crystal structure determination

A pale grey needle of dimensions 0.36 × 0.11 × 0.11 mm³ was selected and covered in a thin film of perfluoropolyether oil and mounted at the end of a glass fibre. (The ESI† contains a photograph of the crystal studied.) The crystal was mounted on a Stoe IPDS2 image plate diffractometer operating with Mo Kα radiation. The crystal was cooled to 100 K using an Oxford Instruments nitrogen gas cryostream. X-ray diffraction data were collected in two series of ω-scans, with 1° frames and a counting time of 600 s per frame. Data were integrated using standard procedures within the package Stoe X-AREA and corrected for the effects of absorption using a face-index technique. The unit cell was determined using 6024 unique reflections. X-ray powder diffraction confirmed that the crystal examined was representative of the bulk product (see ESI†).

Quantum chemical calculations

Geometries were optimised under planar constraints at the SCS-RI-MP2/def2-TZVP++²⁶ level of theory using the ORCA 2.9.1 suite of programs. In order to accelerate the calculations, we also used the RIJ-COSX approximation²⁷ and the RI-MP2 approximation along with automatically generated auxiliary basis sets.

The initial structure of the cyanuric acid molecule stacked on a single melamine molecule was taken from our crystal structure, while the other two models were constructed by replacing the relevant molecule by either a cyanuric acid molecule or a melamine molecule to obtain a realistic stacking arrangement.

In order to provide a reliable estimation of the stacking energy, we also performed complete basis set (CBS) SMP3 calculations.²⁸ These were performed using the ORCA suite and used an aug-pVnZ basis set,²⁹ combined with the automatic extrapolation procedure implemented in the suite.³⁰ We used a combination of aug-cc-pVTZ and aug-cc-pVQZ to obtain the extrapolated RI-MP2 value and an RI-MP3/aug-ano-pVDZ³⁰ calculation to obtain the third order correction, E(3). This E(3) correction was then scaled by 0.6 and added to the RI-MP2/CBS energy estimation as recommended by Pitonák et al.²⁸

Results and discussion

Unit cell symmetry

Crystals of CA.M were grown by hydrothermal synthesis by partial acid hydrolysis of melamine in aqueous solution. From the product a single crystal representative of the bulk was selected and studied by X-ray diffraction.‡ The diffraction data could be indexed using the previously reported cell (space group C2/m; a = 14.853(2) Å; b = 9.641(2) Å; c = 3.581(1) Å; β = 92.26(1)°; V = 512.4(2) ų (T = 298 K)), but careful inspection of the diffraction images showed the presence of additional weak reflections not fitted by this cell, consistent with a doubling of the c-axis. We therefore collected a full data set with the crystal held at 100 K with high data redundancy (redundancy 4.9, R_int = 0.0462). We did not observe significant diffuse scattering in the diffraction images. The diffraction data can be indexed with an I-centred monoclinic cell of approximately double the volume (a, b, 2c, α, β, γ) with space group I2/m; a = 14.8152(19) Å; b = 9.6353(18) Å; c = 7.0405(9) Å; β = 93.194(11)°; V = 1003.5(3) ų (T = 100 K). The I-centred cell was unambiguously identified from systematic absences in the data and contains two separate half molecules within the asymmetric unit: melamine and cyanuric acid. This cell is metrically doubled compared with the previous cell and is I-centred not C-centred. When the diffraction images were integrated using a primitive cell, this centring was apparent. For a truly C-centred cell, 7088 reflections with h + k = 2n + 1 would have zero intensity. However, of these 155 have I > 4sigma(I). For a truly I-centred cell, 7044 reflections with h + k + l = 2n + 1 would have zero intensity. We did not observe any reflections in this group with I > 4sigma(I). Refinements carried out in C2/m are stable, but lead to non-positive definite displacement parameters for C/N/O. There are no such problems with the refinement in I2/m. We note that it would be possible to convert the I-centred monoclinic cell into the standard setting (a′ = c − a; c′ = −a) which would generate a C-centred cell, but we have chosen the I-centred cell for easy comparison with the previous report.

Crystal structure

The unit cell has approximately double the volume of the previously reported cell, and contains a strictly ordered array of melamine and cyanuric acid. The asymmetric unit is shown in Fig. 2. Pleasingly, the assignment of atoms types was greatly assisted by unambiguous location of hydrogen atoms identified from Fourier difference maps, within which the amine group of melamine and C–H bonds of cyanuric acid in its keto form were clearly visible. (Fig. 3a and 3b) There is evidence of proton transfer from cyanuric acid to melamine in approximately 35% of CA.M. This was allowed for in the data analysis. Full details can be found in the ESI.†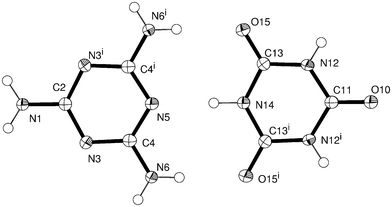

Fig. 2 Discrete melamine and cyanuric acid molecules within CA.M. Atoms are drawn as 50% probability ellipsoids. Symmetry equivalent atoms are generated by the operator i = x, −y, z.

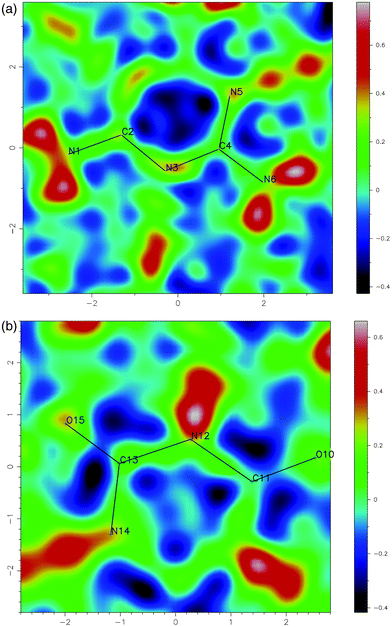Fig. 3 Two-dimensional difference Fourier maps calculated using all data showing the location of electron density corresponding to hydrogen atoms in (a) melamine and (b) cyanuric acid.

Selected bond lengths and angles within the melamine and cyanuric acid components are shown in Table 1. The bond lengths of two molecules are significantly different. For melamine, the mean length of the C–N bonds within the ring is 1.3579(16) Å, but for cyanuric acid the mean length is 1.3733(16) Å. More strikingly, the bond distances confirm that cyanuric acid is present in the keto form: for melamine the mean C–NH₂ bond length is 1.3272(18), but for cyanuric acid the equivalent mean C=O distance is 1.2337(17) Å. Each of the rings is distorted from a perfect hexagon, but they are distorted in different ways. At the C=O in cyanuric acid, the bond angles within the rings are 116.26(12)° and 115.92(16)°, but in melamine the equivalent bond angles at the amine group are 124.82(17)° and 124.35(12)°.

Table 1 Selected bond lengths and bond angles within CA.M^a

Melamine			Cyanuric acid
a Symmetry equivalent atoms are generated by the following symmetry operator: i = x, −y, z.
Bond lengths/Å
N(1)–C(2)		1.330(2)	O(10)–C(11)		1.232(2)
C(4)–N(6)		1.3244(16)	C(13)–O(15)		1.2354(14)
	Mean	1.3272(18)		Mean	1.2337(17)
C(2)–N(3)		1.3562(14)	C(11)–N(12)		1.3746(15)
N(3)–C(4)		1.3600(17)	N(12)–C(13)		1.3708(17)
C(4)–N(5)		1.3575(16)	C(13)–N(14)		1.3746(15)
	Mean	1.3579(16)		Mean	1.3733(16)
Angles/°
N(3)–C(2)–N(3)^i		124.82(17)	N(12)–C(13)–N(14)		116.26(12)
C(2)–N(3)–C(4)		115.35(11)	C(13)–N(12)–C(11)		123.97(12)
N(5)–C(4)–N(3)		124.35(12)	N(12)^i–C(11)–N(12)		115.92(16)
C(4)^i–N(5)–C(4)		115.69(17)	C(13)^i–N(14)–C(13)		123.58(16)

---

Here we are able to give precise atom-to-atom distances for each of the hydrogen bonds within the structure. (Table 2) The hydrogen bonding about the melamine molecule illustrated in Fig. 4 shows all the symmetry unique hydrogen bonds within the structure. The present structure determination reveals that the N⋯N distances in the two symmetry unique N–H⋯N hydrogen bonds are very similar and likewise the three symmetry unique N⋯O distances (in N–H⋯O) are very similar, but there is a notable difference between the mean N⋯N distance (2.8537(17) Å) and mean N⋯O distance (2.9409(14) Å). In line with the previous report, the CA.M assembly contains sheets held together by complementary hydrogen bonding between melamine and cyanuric acid. The triangular nature of each molecule gives rise to sheets that contain a very familiar rosette motif.¹⁸ (Fig. 5) The rms deviation of the nine non-hydrogen atoms of the melamine from their mean plane is 0.0155 Å and for cyanuric acid, the rms deviation is 0.0169 Å. These two molecules are effectively coplanar within 3σ; the two means planes subtend an angle of 0.15(6)° to each other. These sheets lie parallel to the plane (101) and are stacked perpendicular to it at a separation of 3.173(2) Å such that melamine and cyanuric acid overlie in adjacent layers. This separation and the orientation of molecules suggest the presence of significant π–π interactions between the layers.

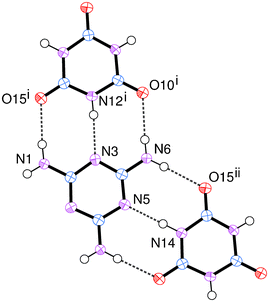Fig. 4 Hydrogen bonding within CA.M. Dashed lines illustrate hydrogen bonds.

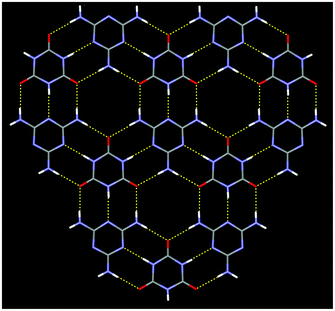Fig. 5 A portion of the hydrogen-bonded sheet within CA.M. Dashed lines illustrate hydrogen bonds.

Table 2 Hydrogen bonds within CA.M^a

Donor (D)	Acceptor (A)	D–H/Å	D⋯A/Å	H⋯A/Å	D–H⋯A/°
a Symmetry equivalent atoms are generated by the following symmetry operators: i = x − ½, y + ½, z + ½; ii = x, −y, z; iii = x + ½, y − ½, z − ½.
N1–H1	O15^i	0.88(2)	2.9379(14)	2.07(2)	172.3(18)
N6–H6A	O15^ii	0.87(2)	2.9469(13)	2.08(2)	177.7(18)
N6–H6B	O10^i	0.84(2)	2.9379(15)	2.11(2)	174.8(19)
N12–H12	N3^iii	0.84(3)	2.8468(14)	2.01(3)	176(2)
N14–H14	N5	0.93(4)	2.8605(19)	1.93(4)	180(3)

---

Relationship between current and previous structure determinations

The previous model presents a disordered structure; we will explore the two possible reasons for this. The first possibility is that the true cell is the one described here and the previous report is based upon a subset of strong reflections from this cell. This explanation has the benefit of being the simplest solution and is understandable given the molecules involved. From our diffraction data it is possible to compare the intensities of pertinent groups of reflections. For all the observed low angle data, hkl, (to 40° 2θ for Mo Kα radiation) we observe that <I_{l= 2n}>/<I_{l= 2n+1}> ≈ 90. To put this another way, the subset of strong data consistent with the previous model (l even) are on average 90 times stronger than the weak reflections that point to doubling of the c axis (l odd). It is important to stress that this comes about because the two molecules, melamine and cyanuric acid, are extremely similar: they each have 66 electrons; they each contain almost planar rings that are close to being hexagons; these rings have similar mean bond lengths. These molecules differ in the location of protons and in the way in which there is a small distortion away from a perfect hexagonal ring. Critically, ordering of these two molecules within the layers is manifested by very weak diffraction peaks. Without speculating about the data quality in the previous report, these weak reflections may have been very easy to miss.

The second possible origin of a disordered structure is a lack of registry between well-ordered hydrogen-bonded sheets that gives rise to stacking disorder. This occurs when multiple equivalent ways of stacking of sheets are possible, and the alternative possible stacking arrangements do not differ significantly in energy. Often this is associated with very weak interlayer interactions.³¹ The previous report does not describe any diffuse scattering that would be associated with such faults, but the structure may have been solved ignoring diffuse scattering. We have produced simulated precession images from our raw diffraction data (see ESI†) and examined them for “flocks of parallel diffuse streaks of scattering density”³² along c* (the stacking direction). We see no significant diffuse scattering in our precession images and rows of spots along c* are sharp and discrete and not accompanied by streaks.

At the time, the previous authors must have given considerable thought to the stacking of layers as they address the stacking sequence. Their calculations¹⁹ (ref. 8 within A. Ranganathan et al., J. Am. Chem. Soc., 1999, 121, 1752–1753) demonstrate that an ordered arrangement of melamine and cyanuric acid (CA) in adjacent layers is substantially more stable (37 kJ mol⁻¹) than the arrangement with CA over CA. This calculation is line with the intuitive expectation that the arrangement with electron rich and electron poor rings adjacent is more stable than other possibilities.

We sought to confirm using high level theory calculations that we did indeed have an ordered structure and a regular stacking sequence. The two extreme forms of stacking consistent with the earlier model are these: 1) CA.M above M.CA and 2) CA.M above CA.M (cyanuric–cyanuric acid aligned and melamine–melamine aligned). In order to estimate the stacking energy of these two forms, we performed quantum chemical calculations on three model systems consisting of: a single cyanuric acid molecule stacked on a single melamine molecule, two stacked cyanuric acid molecules, and two stacked melamine molecules. Hydrogen bonding, which is confined to the almost planar layers, was not included the model as the focus is on inter-layer interactions.

Detailed results are contained within the ESI† and these demonstrate that there is a favourable stacking energy when melamine and cyanuric acid are stacked as in the crystal structure described here. For two non-interacting stacks, the observed stacking arrangement (CA.M above M.CA) is predicted to be about 25 kJ mol⁻¹ more stable than an arrangement with CA.M above CA.M. Notably, the stacking interaction between cyanuric acid molecules is energetically unfavourable which de-stabilises the arrangement with CA.M above CA.M even if the melamine–melamine stacking is energetically favourable.

Our calculations improve over the semi-empirical estimates of the earlier work¹⁹ and are compatible with their study. However, it is known that weak stacking interactions are often ill-described by the semi-empirical techniques used in the earlier study and thus our results provides a more rigorous basis for comparison of the interactions. We believe we have correctly identified an ordered variant of CA.M and our calculations confirm there is a substantial energy penalty if different layer stacking arrangements are adopted.

Conclusion

The unequivocal elucidation of this structure sheds light upon the formation of CA.M, a jewel in the crown of crystal engineering. The present study demonstrates the importance of inspection of diffraction images and reinforces the need to obtain accurate measurements of weak reflections. In the original report of CA.M the analogous compound melamine–thiocyanuric acid was reported also to crystallise in the small C-centred unit cell. For the reasons described above for CA.M we believe this is incorrect and aim to resolve this. The greatly enhanced contrast in scattering between nitrogen and sulfur should aid this work.

Acknowledgements

The authors are grateful to the EPSRC for funding under grant EP/I028692/1.

References

1. Y. Tsuya, M. Watanabe, T. Hirae, M. Sato and A. Kawakita, ASLE Trans., 1981, 24, 49–60.
2. N. Feng, J. Liu, H. Uyama and W. Liu, Appl. Mech. Mater., 2012, 130–134, 1511–1515.
3. W. Yang, G. Tang, L. Song, Y. Hu and R. K. K. Yuen, Thermochim. Acta, 2011, 526, 185–191.
4. H. B. Hans, US Pat., US3532635A, 1970.
5. P. L. Posson and M. L. Clark, US Pat., US20050242319A1, 2005.
6. C. J. Downes, J. W. Mitchell, E. S. Viotto and N. J. Eggers, Water Res., 1984, 18, 277–280.
7. C. G. Skinner, J. D. Thomas and J. D. Osterloh, J. Med. Toxicol., 2010, 6, 50–55.
8. Y. Wei and D. Liu, Toxicol. Ind. Health, 2012, 28, 579–582.
9. S. Hassani, F. Tavakoli, M. Amini, F. Kobarfard, A. Nili-Ahmadabadi and O. Sabzevari, Food Addit. Contam., Part A, 2013, 30, 413–420.
10. S. Taksinoros and H. Murata, J. Vet. Med. Sci., 2012, 74, 1569–1573.
11. K. Ai, Y. Liu and L. Lu, J. Am. Chem. Soc., 2009, 131, 9496–9497.
12. S. Yang, J. Ding, J. Zheng, B. Hu, J. Li, H. Chen, Z. Zhou and X. Qiao, Anal. Chem., 2009, 81, 2426–2436.
13. L. Zhu, G. Gamez, H. Chen, K. Chingin and R. Zenobi, Chem. Commun., 2009, 559–561.
14. N. Yan, L. Zhou, Z. Zhu and X. Chen, J. Agric. Food Chem., 2009, 57, 807–811.
15. T. H. Tsai, S. Thiagarajan and S. M. Chen, J. Agric. Food Chem., 2010, 58, 4537–4544.
16. Y. T. Liu, J. Deng, X. L. Xiao, L. Ding, Y. L. Yuan, H. Li, X. T. Li, X. N. Yan and L. L. Wang, Electrochim. Acta, 2011, 56, 4595–4602.
17. C. T. Seto and G. M. Whitesides, J. Am. Chem. Soc., 1990, 112, 6409–6411.
18. J. A. Zerkowski, C. T. Seto and G. M. Whitesides, J. Am. Chem. Soc., 1992, 114, 5473–5475.
19. A. Ranganathan, V. R. Pedireddi and C. N. R. Rao, J. Am. Chem. Soc., 1999, 121, 1752–1753.
20. C. B. Aakeroy and P. D. Chopade, Cocrystals: synthesis, structure, and applications, 2012.
21. H.-F. Ji and X. Xu, Langmuir, 2010, 26, 4620–4622.
22. H.-M. Zhang, Z.-X. Xie, L.-S. Long, H.-P. Zhong, W. Zhao, B.-W. Mao, X. Xu and L.-S. Zheng, J. Phys. Chem. C, 2008, 112, 4209–4218.
23. D. Musumeci and M. D. Ward, CrystEngComm, 2011, 13, 1067–1069.
24. K. Wang, D. Duan, R. Wang, D. Liu, L. Tang, T. Cui, B. Liu, Q. Cui, J. Liu, B. Zou and G. Zou, J. Phys. Chem. B, 2009, 113, 14719–14724.
25. F. H. Allen, Acta Crystallogr., Sect. B: Struct. Sci., 2002, 58, 380–388 , see www.ccdc.cam.ac.uk.
26. F. Weigend and R. Ahlrichs, Phys. Chem. Chem. Phys., 2005, 7, 3297–3305.
27. F. Neese, F. Wennmohs, A. Hansen and U. Becker, Chem. Phys., 2009, 356, 98–109.
28. M. Pitonak, P. Neogrady, J. Cerny, S. Grimme and P. Hobza, ChemPhysChem, 2009, 10, 282–289.
29. T. H. Dunning, J. Chem. Phys., 1989, 90, 1007–1023.
30. F. Neese and E. F. Valeev, J. Chem. Theory Comput., 2011, 7, 33–43.
31. H. Birkedal, H. B. Burgi, K. Komatsu and D. Schwarzenbach, J. Mol. Struct., 2003, 647, 233–242.
32. H. B. Burgi, M. Hostettler, H. Birkedal and D. Schwarzenbach, Z. Kristallogr., 2005, 220, 1066–1075.

---

Footnotes

† Electronic supplementary information (ESI) available: Simulated precession photographs, X-ray powder diffraction data, details of quantum chemical calculations, details of hydrogen atom positions, and tables of crystal data are contained within the ESI. CCDC 935325. For ESI and crystallographic data in CIF or other electronic format see DOI: 10.1039/c3ce40709h

‡ C₃H₃N₃O₃·C₃H₆N₆; M_r = 510.40; Z = 2; monoclinic, a = 14.8152(19) Å, b = 9.6353(18) Å, c = 7.0405(9) Å, β = 93.194(11)°, V = 1003.5(3) ų; T = 100 K; space group I2/m; no. of reflections (measured/independent) 7067/1434; R_int = 0.046; final R₁ values (I > 2sigma(I), all data) 0.035, 0.0727; final wR(F²) values (I > 2sigma(I), all data) 0.0898, 0.1047; radiation type Mo Kα; GooF 0.957.

---