How hydrogen bonding affects ligand binding and fluxionality in transition metal complexes: a DFT study on interligand hydrogen bonds involving HF and H₂O

Abstract

DFT calculations (B3PW91) predicted structures for hydrogen-bonded complexes of type Ir(H)(L)(bq–G)(PH₃)₂^q+ (bq–H = benzo[h]quinoline-10-yl, L = empty site, FH or OH₂; G = H or NH₂, q  = 1; L = F⁻, G = NH₂, q  = 0), which are either too unstable for X-ray crystallography study, or for which the crystal structure does not allow H atom positions reliably to be located. The work shows how the two-point binding site provided by the bq–NH₂ complex is ideal for HF but not for H₂O binding, thus stabilizing the former to the extent that it can be observed by NMR at low temperature. Fluxionality in the aqua complex is fully interpreted by location of the appropriate TS. One such TS is strongly stabilized by hydrogen bonding leading to rapid exchange of NH₂ positions even at −80 °C. An improved ligand is suggested for stabilizing an HF complex.

---

Introduction

Interaction energies in chemistry go from the weak van der Waals bond (100 cm⁻¹ for D₀ in Ar·H₂O)¹ to the strong covalent bond (150 kcal mol⁻¹ for C–F bond in C₆F₆).² The directionality and intermediate interaction energy of the hydrogen bond allow creation of specific interactions in crystal engineering and supramolecular architecture;^3–5 theoretical studies on weak hydrogen bonds have been reviewed recently.⁶

Multifunctional ligands with reactive groups not involved in metal–ligand binding are of particular interest when they contain lone pairs unable to bind directly to the metal, but able to hydrogen bond to an adjacent ligand.^7,8 If properly placed, these groups could have effects similar to those of hydrogen-bonding residues in the active site cavity of metalloenzymes which are believed to contribute to the acceleration of enzyme reactions by transition-state stabilization.⁹

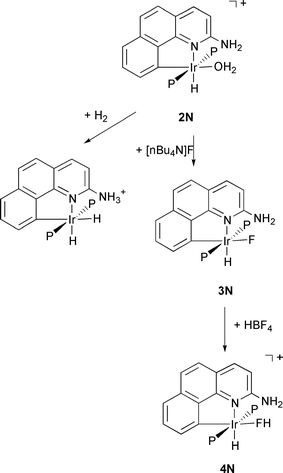
A simple example is shown as 1 below, where G can be either H (denoted 1H) or a pendant amino group NH₂ (denoted 1N) capable of hydrogen bonding to an incoming ligand L′ bearing acidic protons (HF, H₂O, for example). In prior work we have shown how water binds to 1H to yield the aqua complex Ir(H)(H₂O)(bq–H)(PPh₃)₂⁺2H (bq–H = benzo[h]quinolin-10-yl) that can reversibly bind H₂ with loss of H₂O.^10,11

In the case of G = NH₂, the aqua complex Ir(H)(H₂O)(bq–NH₂)(PPh₃)₂⁺2N reacts with H₂ to give reversible heterolytic cleavage of H₂ not seen for 2H (Scheme 1).¹² Moreover, the complex 2N reacts rapidly with [nBu₄N]F to give the fluoride complex 3N (Scheme 1).^13,14

Scheme 1

Ligand substitution in metal fluoro complexes is subject to acid catalysis and the intermediacy of transient HF complexes was proposed¹⁵ but experimental support was never obtained in prior studies. Fully characterized halogenocarbon complexes of type L_nM–X–R (X = halide, R = alkyl or aryl) have been known for some time,¹⁶ but the corresponding hydrogen halide complexes (R = H, X = F) have only been reported very recently.^13,14,17 Two bifluoride complexes, Mo(PMe₃)₄(H)₂(F)(FHF)¹⁸ and trans-Ru(dmpe)₂(H)(FHF),¹⁹ have also been characterized very recently by X-ray crystallography. In both cases the bifluoride ligand is η¹- bonded to the metal with an M–F···F angle of 134 and 130°, respectively.²⁰ The hydrogen-bond within the coordinate bifluoride is asymmetric and less strong than in free FHF⁻.

The HF complex 4N is obtained by protonation of 3N (HBF₄·Et₂O) and has so far only been detected spectroscopically and at low temperature (Scheme 1). The critical observation was the finding of a J_HF value of 440 Hz, characteristic of hydrogen bonded HF. The pendant group NH₂ is believed to interact with the HF ligand through hydrogen bonding to give overall two point binding [italic v]ia an Ir–F coordinate bond and an N···HF hydrogen bond. With the stabilization of an HF complex, we now have experimental support for the presence of such intermediates in acid catalysed substitution and also a new ligand type.

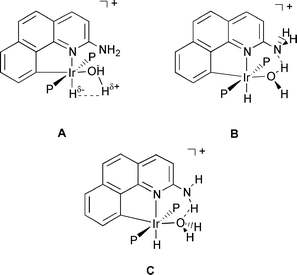
Since the HF complex decomposes with loss of HF above −30 °C, no crystal structure is available and so the metric parameters of this new ligand type were still unknown. Efforts to make stabler derivatives having failed, we turn here to DFT (B3PW91) studies to predict the static structure expected for the new ligand. Being difficult to locate with X-ray diffraction the presence of H is often inferred from IR and NMR spectroscopic observations. Theory is one of the most powerful tools available to characterize the geometry of hydrogen bonded species and an accurate determination of the hydrogen atom positions is provided by DFT calculations.²¹ The aqua complex 2N provides a stringent test of the DFT methodology with three types of hydrogen bonding situations in competition (Scheme 2).

Scheme 2

The aim of this paper is thus to explore computationally the structure and reactivity of the complexes between 1 and ligands like H₂O, F⁻ or HF. Emphasis will be put on the role of G and thus on how hydrogen bonding helps in stabilizing particular geometries and in determining reactivity. One of our goals is to use DFT calculations to analyse the trends in binding energies between the metal fragment 1 and ligands like H₂O or HF as a function of pendant group G.

Computational details

All calculations have been performed within the framework of Density Functional Theory (DFT) with the GAUSSIAN 98 set of programs.²² The B3 hybrid exchange potential of Becke²³ was used in conjunction with the PW91 correlation potential of Perdew and Wang.²⁴ The relativistic Effective Core Potential (RECP) of the Stuttgart group was used for Ir with the associated (8s7p5d)/[6s5p3d] basis set augmented with an f function (α  = 1.0).²⁵ The phosphorus atoms were also treated with Stuttgart's RECPs and the associated basis set,²⁶ augmented by a d function (α  = 0.387). A 6-31G(d,p) basis set was used for the hydride, the fluoride, both atoms (C and N) linked to Ir, and for the atoms of the G group (G = H or NH₂). For the remaining atoms a 6-31G basis set was used.

Geometry optimizations without any symmetry constraints were performed on model systems where the three conjugated rings of the bq–G (G = H or NH₂) ligand were explicitly considered. The only difference between the experimental and the calculated molecules lies in the use of PH₃ to represent the phosphine ligands. This turns out to be a reasonable simplification since the electronic influence of PPh₃ is rather well reproduced by PH₃ according to the respective Tolman electronic factors (2069 cm⁻¹ for PPh₃[italic v]s. estimated 2081 cm⁻¹ for PH₃).²⁷ We will therefore not introduce an additional labeling scheme to distinguish between experimental and theoretical systems. This study being mostly theoretical, we will explicitly indicate when we refer to experimental results.

The nature of all located extrema was checked through analytical computations of the vibrational frequencies. The binding energy Δ_bind^XE(L′) of the ligand L′ in the complex 1X–L′ (X = H for G = H and X = N for G = NH₂) is evaluated as in eqn. (1) where E_opt(Y) is the electronic energy of system Y in its optimized geometry (binding energies are thus defined to be positive). Note that no basis set superposition error (BSSE) correction has been introduced since we focus on trends only (role of G) and BSSE is known to be small for DFT calculations with a double zeta polarisation (DZP) quality basis set.²⁸

Results and discussion

G = H: Hydrogen bond?

Aqua complex: L′  = H₂O. Benzo[h]quinoline reacts smoothly with the complex [Ir(cod)(PPh₃)₂]BF₄ and hydrogen gas in moist CH₂Cl₂ to give the cyclometallated complex 2H,¹⁰ a rare example both of an organometallic aqua species and of an aqua hydride complex.^29,30 The cyclometallated nature of the benzoquinoline ligand bq–H was clearly established by X-ray crystallography.¹¹ Although the hydride and the water protons were not refined, the connectivity around the metal center was characterized from NMR and IR studies: H₂O trans to C and H trans to N.

The optimized geometry for complex 2H is shown in Fig. 1 along with some geometrical parameters. The agreement with the crystal structure is acceptable since the crystallographic determination was of only moderate quality (R  = 8.5%). The best agreement is obtained for Ir–C (2.018 [italic v]s. 1.99(2) Å), Ir–P (2.318 [italic v]s. 2.336(7) and 2.319(7) Å), and the bite angle of the bq–H ligand (80.2 [italic v]s. 80.2(9)°). The Ir–N (2.189 [italic v]s. 2.10(2) Å) and Ir–O (2.319 [italic v]s. 2.26(2) Å) bonds are computed too long. The Ir–H bond distance (1.593 Å) is typical of iridum (III) complexes.

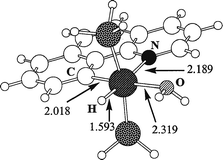Fig. 1 Optimized geometry for the aqua complex, 2H, with G = H. Bond distances are in Å.

The calculated binding energy Δ_bind^HE(H₂O) of the H₂O ligand is 23.2 kcal mol⁻¹. This value is rather high for an aqua complex especially with a long Ir–O bond distance (2.319 Å). This finding is in agreement with the experimental observation of tenacious binding of the aqua ligand in 2H. Even if the reagents and solvents used are dried, the aqua complex still tends to be formed from adventitious water.¹¹

One factor that leads to the special stability of the aqua complex can be seen from the geometry of 2H (Fig. 1) where one O–H bond is aligned with the Ir–H bond (H–Ir–O–H dihedral angle: 4°). This “cis interaction” (A in Scheme 2) between polarized M^δ+  − H^δ− and O^δ−  − H^δ+ bonds resembles that described by Milstein et al. for cis-[Ir(H)(OH)(PMe₃)₄][PF₆],³¹ where the neutron diffraction structure³² shows a short O–H···H–Ir distance of 2.40(1) Å and a small Ir–O–H angle of 104.4(7)°. This orientation, reproduced by DFT calculations,³³ was explained by a dipole–dipole interaction.³⁴

Although there is certainly no covalent bond between the basic hydride and the acidic proton on H₂O in complex 2H (H···H 2.642 Å), the interaction might have been viewed as a hydrogen bond, but, this “hydrogen bond”, if any, is certainly very weak both from experiment (the two Hs on H₂O are equivalent by NMR even at low temperature) and from theory (the two O–H bond distances are the same: 0.966 Å). Interestingly the Ir–O–H angles are different. That for the O–H aligned with Ir–H is significantly smaller (108.6 [italic v]s. 117.1°). The metal–hydride bond might thus be considered as a source of weak attracting interaction. This cis effect³⁵ is sufficiently strong to induce a preferential orientation for an H₂ ligand in cis position with respect to M–H: in a cis-M(H)(H₂) unit, M, H and H₂ are almost always coplanar.²¹

Hydrogen fluoride complex: L′  = HF. By reaction with [nBu₄N]F, the water ligand in complex 2H is substituted by fluoride to give 3H.¹⁴ Upon protonation at −80 °C, no HF complex is obtained and the aqua complex is recovered with loss of HF. The same procedure led to the observation of an HF complex when G = NH₂,^13,14 and is directly related to the hydrogen-bonding properties of the G group ([italic v]ide infra).

The instability of the HF complex for G = H could then be attributed to the absence of stabilizing interaction within the complex. However, we showed for 2H that the Ir–H bond is a source of stabilization (although weak) for the X^δ−  − H^δ+ bond. We therefore looked for possible complexation of HF to 1H and found the ground state structure 4H (Fig. 2). The essential feature of the optimized structure 4H is the geometry of the HF ligand. The Ir–F bond (2.416 Å) is much longer than the usual range and in particular much longer than in the bifluoride complexes (2.124(3) Å for Mo(PMe₃)₄(H)₂(F)(FHF)¹⁸ and 2.284(5) Å for trans-Ru(dmpe)₂(H)(FHF)¹⁹).³⁶ The Ir–F–H angle of 107.5° is smaller than the corresponding angle for Mo(PMe₃)₄(H)₂(F)(FHF) (123.4°) indicating some attraction between the basic hydride and the acidic proton. As expected for an attractive effect, the coordinated HF in 4H is slightly elongated in comparison with free HF (0.930 [italic v]s. 0.922 Å).

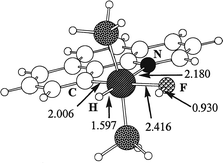Fig. 2 Optimized geometry for the hydrogen fluoride complex, 4H, with G = H. Bond distances are in Å.

The binding energy Δ_bind^HE(HF) of 18.0 kcal mol⁻¹ is rather high, a result that can not entirely be attributed to the cis effect. We have therefore tried to estimate the magnitude of the stabilizing cis attraction by Ir–H. Rotating the HF ligand by 180° around the Ir–F bond removes the cis interaction. A geometry, 4H-TS, was optimized as a transition state on the potential energy surface. The motion along the coordinate associated with the imaginary frequency corresponds to a departure of the HF ligand from the bq plane to yield back 4H. The geometry of the HF ligand in the TS confirms the absence of cis attraction (Ir–F 2.437, H–F 0.928 Å, and Ir–F–H 124.3°). The relative energy of +3.1 kcal mol⁻¹ for 4H-TS with respect to 4H gives an estimate of the stabilizing energy associated with the cis effect which gives only a small contribution to the total binding energy (18.0 kcal mol⁻¹).

The coordination of HF being thermodynamically favored, why have so few systems been characterized? In the particular case of 4H a key point is that the binding energy of H₂O is stronger and the equilibrium (2) is shifted to the right. The reaction energy of −5.2 kcal mol⁻¹ is large enough for the thermal population of 4H to be negligible (⪡1%), and moreover free HF is very reactive toward glassware. The equilibrium is then probably completely shifted to the right.

To isolate an HF complex it is necessary both to increase the binding energy and to protect the acidic proton from any adventitious base. The key is to design a system with a strong hydrogen-bonding capability. The basic group must not be too strong however, or the proton will transfer to the base and we will no longer have an HF complex.

G = NH₂: Hydrogen bond!

Fluoride complex: L′  = F⁻. The aqua complex 2N reacts with [nBu₄N]F to yield the fluoride complex Ir(H)(F)(bq–NH₂)(PPh₃)₂3N.^13,14 The crystal structure for 3N does not show the hydrogen atoms and, in particular, does not permit structural characterization of the hydrogen bonding pattern.¹⁴

The optimized geometry for complex 3N (Fig. 3) is in very good agreement with the crystal structure. The NH₂ group is almost planar and the nitrogen lone pair conjugates with the bq rings as illustrated by the short C2–N2 bond (1.348 Å). The G group and the vacant site in 1 define a “ binding cavity” where two-point binding of L′ with Ir and G may occur.³⁸ The NH₂ hydrogens are labeled H_endo (toward the vacant site) and H_exo (away from the vacant site). From the optimized structure for 3N (Fig. 3) there is clearly an F···H–N hydrogen bond, in agreement with the experimental results. The N–H_endo bond is noticeably elongated compared to N–H_exo (1.051 [italic v]s. 1.005 Å). The F···H distance of 1.560 Å is consistent with the observed J(F,H) coupling constant of 52 Hz at 193 K. In the optimized structure 3N the two NH₂ protons are inequivalent as observed by NMR at low temperature.

Fig. 3 Optimized geometry for the fluoride complex, 3N, with G = NH₂ and comparison with geometrical parameters for the corresponding experimental structure (PPh₃).¹⁴ Bond distances are in Å and angles are in degrees.

Having established the existence of an F···H–N hydrogen bond in complex 3N we now turn to the estimation of the strength of this bond. Experimentally, this is usually done by VT NMR experiments in which the two NH₂ protons are different at low temperature but become equivalent at room temperature. The Gibbs energy of activation ΔG^‡ for H exchange (12.4 kcal mol⁻¹) gives an estimate of the strength of the hydrogen-bond after correction for the intrinsic rotation barrier around the C–N bond.⁸

This correction procedure is easily carried out through calculations by locating the appropriate transition states for rotation around the C–N bond. Starting from 1N (vacant site trans to C) the NH₂ group can be rotated in two ways (Fig. 4). The structure 1N-TS_endo, optimized as a transition state (i209 cm⁻¹), contains a nitrogen lone pair pointing toward the vacant site (endo position, Fig. 4). A second transition state 1N-TS_exo (i355.9 cm⁻¹) has a nitrogen lone pair pointing away from the vacant site (exo position, Fig. 4). As expected, the interaction between the nitrogen lone pair and the vacant site in 1N-TS_endo favors this structure over the other one 1N-TS_exo: +4.3 [italic v]s. +8.8 kcal mol⁻¹. It is interesting that it costs only 4.3 kcal mol⁻¹ to reach a geometry (1N-TS_endo) ideally suited for hydrogen bonding interaction with an incoming ligand L′ ([italic v]ide infra).

Fig. 4 Transition states for NH₂ rotation in the complex with L = vacant site and G = NH₂, 1N. See text for definition of endo and exo; energies in kcal mol⁻¹.

Two similar transition states for H exchange, 3N-TS_endo (i489 cm⁻¹) and 3N-TS_exo (i501 cm⁻¹), were located (Fig. 5). Owing to the lone pairs of the fluoride ligand, lone pair–lone pair repulsion may be present in the transition state. It is therefore not surprising to obtain an inversion of the TS order with 3N-TS_endo lying at +29.9 kcal mol⁻¹ above 3N and 3N-TS_exo lying at +17.3 kcal mol⁻¹. The latter is in fair agreement with ΔG^‡  = 12.4 kcal mol⁻¹ for H exchange at 193 K in 3N (VT NMR). If the calculated 3N-TS_exo is stabilized by hydrogen bonds to CD₂Cl₂ solvent experiment and theory can be reconciled.

Fig. 5 Transition states for NH₂ rotation in the complex with fluoride ligand and G = NH₂, 3N. Details as in Fig. 4.

The exchange mechanism for the two Hs of NH₂ does not require a 360° rotation around the C–N bond. A 180° rotation through 3N-TS_exo is sufficient to achieve exchange (Scheme 3). With equivalent phosphine ligands, configurations (a) and (b) are enantiomeric and thus equivalent in NMR studies in an achiral solvent.

Scheme 3

The difference in relative energy for 3N-TS_endo and 3N-TS_exo illustrates the concept of transition-state stabilization.⁹ The lower activation energy for 3N-TS_exo arises from a conjunction of two factors: the absence of lone pair–lone pair repulsion and the presence of a hydrogen bonding interaction between F and both N–H bonds. From the relative energies of the four TS, the following semi-quantitative scheme can be derived: ΔE_rot  +  ΔE_lp,vs  = 4.3; ΔE_rot  = 8.8; ΔE_rot  +  ΔE_lp,lp  +  ΔE_H-bond  = 29.9; ΔE_rot  +  ΔE_H-bond  = 17.3. Here ΔE_rot is the intrinsic rotation barrier for NH₂ , ΔE_lp,vs the stabilizing (negative) energy associated with lone pair–vacant site interaction in 1N-TS_endo, ΔE_H-bond the energy of the hydrogen bond in 3N lost in both TS (3N-TS_endo and 3N-TS_exo), and ΔE_lp,lp the lone pair–lone pair repulsion introduced in 3N-TS_endo. The neglect of the weak hydrogen-bonding (2.292 Å) stabilizing term in 3N-TS_exo leads to a slight overestimation of the lp–lp repulsion. We thus obtain the following values: ΔE_rot  = 8.8, ΔE_lp,vs  =  −4.5, ΔE_lp,lp  = 12.6 and ΔE_H-bond  = 8.5 kcal mol⁻¹. The strength of the hydrogen bond in complex 3N can thus be estimated as 8.5 kcal mol⁻¹ in agreement with estimated values in related systems.^6,39

Hydrogen fluoride complex: L′  = HF. In their classic 1958 text, Basolo and Pearson⁴⁰ indicate that the acid catalysis of fluoride substitution in cobalt (III) complexes implies that the fluoride ligand can be protonated to give a transient hydrogen fluoride complex of the type M–F–H, which rapidly loses HF. Protonation of the pendant amine fluoro-complex, 3N, with HBF₄ ·Et₂O at 195 K in CD₂Cl₂ leads to formation of the protonated form, 4N, in solution (Scheme 1).^13,14 The complex decomposed on attempted isolation, but the ¹H NMR data at 183 K of 4N indicated it is a hydrogen fluoride complex. The key observation is the presence of a ¹J(H,F) coupling of 440 Hz consistent with 4N being a true N···H–F complex, rather than having a hydrogen-bonded N–H···F system as in 3N (¹J(H,F) = 52 Hz).

The existence of the HF complex being established, we turn to DFT calculations to predict its structure. The optimized geometry of the complex clearly exhibits a coordinated HF molecule interacting with the amino group (Fig. 6). The essential features are entirely consistent with those deduced from the spectral data but we can now also predict the key metric parameters. The H–F ligand lies in the “ binding cavity” with an F–H···N bond to G. The H–F distance of 1.042 Å is slightly longer than that of monomeric HF (0.922 Å),⁴¹ but much shorter than the F···H_endo–N distance (1.560 Å) in the neutral system 3N. The formation of an H–F bond has also resulted in a significant elongation of the Ir–F bond (from 2.123 Å in 3N to 2.262 Å in 4N). However the variation is much smaller for G = NH₂ than for G = H where the Ir–F bond elongates from 2.072 Å for 3H to 2.416 Å for 4H as a result of a stronger HF bond (0.930 Å).

Fig. 6 Optimized geometry for the hydrogen fluoride complex, 4N, with G = NH₂. Bond distances are in Å.

The distance between N and the H of HF (1.43 Å) is considerably longer than in the two normal N–H covalent bonds (1.021 Å). This is entirely consistent with the description of 4N as an HF complex. The F···N distance of 2.470 Å is much shorter than the F···N distance found experimentally (X-ray) for the fluoride complex 3N (2.674 Å). The N–H–F angle of 175° is in excellent agreement with the linear arrangement usually preferred for hydrogen bonding given the constraints of the bonding site. The marked pyramidalization of N certainly increases the Lewis basicity of the lone pair involved in the hydrogen bond.

The calculated binding energy Δ_bind^NE(HF) of 28.2 kcal mol⁻¹ in complex 4N is very much higher than for G = H (18.0 kcal mol⁻¹) consistent with the presence of additional stabilization as a result of hydrogen bonding. We can qualitatively estimate the hydrogen bonding contribution. The intrinsic binding energy of HF to Ir is 15 kcal mol⁻¹ as deduced from 4H-TS where the cis interaction with Ir–H is absent. The hydrogen bonding strength in 4N is best evaluated with 1N-TS_endo as a reference (having a similar orientation of the NH₂ group). The total binding energy is therefore 32.5 kcal mol⁻¹ from which the intrinsic binding energy of HF needs to be subtracted. This yields a qualitative estimate of the hydrogen bond in 4N of 17.5 kcal mol⁻¹. The value is doubled by comparison to 3N (8.5 kcal mol⁻¹) in agreement with N being a better base than F and HF being a better acid than RNH₂.

To elucidate the origin of such a stabilization we have carried out an Atoms in Molecules⁴³ analysis (AIM) of the electron density for the two HF complexes 4H and 4N. The important result (Table 1) from this analysis is the existence of bond critical points for 4N for both F–H and H···N bonds. However the two bonds are not of the same type. The electron density ρ_b at the critical point is much higher for F–H (Table 1). The Laplacian of the density also shows that more electron density has accumulated in the vicinity of the critical point for F–H (more negative value). Finally, the electron energy density is clearly more negative for F–H than for N···H. This is a sign of increased covalent character of the bond.⁴⁴ Therefore we can conclude that, in 4N, there is clearly a hydrogen bond as illustrated by the presence of two critical points. The hydrogen bond can best be described as F–H···N with a covalent bond between F and H and a donor/acceptor type of bond between H and N.

Table 1 Results of the AIM analysis (in atomic units) for complexes 4H and 4N. Only properties at selected bond critical points are shown. ρ_b is the value of the density, ∇ρ_b² the Laplacian of the density, ε the ellipticity, and H_b the electron energy density all calculated at the corresponding critical point

4H				4N
Bond	ρb	∇ρb^2	ε	Hb	ρb	∇ρb^2	ε	Hb
Ir–H	0.1549	0.0744	0.0590	−0.0867	0.1607	0.0484	0.0613	−0.0929
Ir–F	0.0355	0.1805	0.0316	−0.00268	0.0541	0.267	0.240	−0.0048
F–H	0.3574	−2.730	0.0028	−0.7660	0.2426	−0.8965	0.0036	−0.3344
N···H	—	—	—	—	0.1075	−0.0107	0.0049	−0.0618

---

Moreover, the AIM analysis for complex 4H does not show any critical point between the hydride and the acidic H of HF. The cis interaction is thus best described as a dipole–dipole interaction between polarized bonds. The presence of the amino group in 4N has completely reversed the geometrical preference by creating the F–H···N hydrogen bond. We have tried to optimize the complex Ir(H)(F)(bq–NH₃⁺)(PH₃) with an N–H···F hydrogen bond but the system always returned to 4N. This is consistent with N being a better hydrogen bond acceptor than F, and H–F being a better hydrogen bond donor than N–H. The case of L′  = H₂O is somewhat analogous in this respect and also shows the dichotomy of hydrogen bond acceptor/hydrogen bond donor power, to a lesser extent however.

Weaker hydrogen bonds in water compound: L′  = H₂O. Experimentally, the detailed structure of the aqua complex 2N was not well determined from ¹H NMR spectroscopy.¹⁴ At 25 °C the protons of the bq–NH₂ are equivalent, and free and bound H₂O undergo fast exchange. At −80 °C the free/bound H₂O exchange is frozen out and the bound H₂O appears in the form of two resonances in 1:1 ratio. The bq–NH₂ appears as a single resonance at all temperatures. Therefore structure B (Scheme 2) was weakly preferred from the experimental observations. A theoretical study of the dynamics of H exchange was thought necessary to put our understanding of the NMR results on a firmer footing.

We now find the predicted ground state structure for complex 2N is best described as structure B (Scheme 2 and Fig. 7). The essential feature of the structure is the presence of an O–H···N hydrogen bond. Each O–H bond is different (O–H_endo 1.004 and O–H_exo 0.963 Å). The O–H–N angle of 159° is not optimal for efficient hydrogen bonding but is imposed by the steric constraints introduced by G = NH₂. The Ir–O bond (2.246 Å) is typical for a water complex.¹¹ Structure 2N (Fig. 7) does not strictly agree with the experimental results because the four protons of NH₂ and H₂O are all different. As the oxygen atom is pyramidal (H_endo–O–H_exo 110°) the seemingly equivalent protons for NH₂ are in fact diastereotopic. We next searched for possible low energy pathways for proton exchange.

Fig. 7 Optimized geometry for the aqua complex, 2N, with G = NH₂. Bond distances are in Å.

The key point governing the dynamics of complex 2N is the maintenance of stabilizing interactions in the transition states. This is in fact the case for the transition state 2N-TS_N that exchanges the Hs of NH₂. The most striking difference between 2N and 2N-TS_N is the planarity of the H₂O ligand in the TS. The O–H bonds are still different (0.993 and 0.960 Å) showing some O–H···N hydrogen bonding is maintained in the transition state. As a consequence 2N-TS_N is only 2.0 kcal mol⁻¹ above 2N indicating very easy exchange.

The transition state coordinate (i416 cm⁻¹) corresponds to the out of plane motion of H_exo. The easy exchange associated with 2N-TS_N therefore keeps both protons on H₂O different while making both Hs on NH₂ equivalent. The very low activation energy of 2.0 kcal mol⁻¹ is consistent with the experimental observation of only one NMR peak for NH₂ even down to the lowest accessible temperature (−80 °C).

We could not locate a second transition state 2N-TS_O with N–H···O rather than O–H···N hydrogen bonding. However, a geometry with the OH₂ perpendicular to the bq plane and NH₂ in the plane is only 7 kcal mol⁻¹ above the ground state. So the barrier for H/H′ exchange in HOH′ must be about the same size. In conclusion the H/H′ exchange in the NH₂ group is fast and one NMR peak is always seen but the H/H′ exchange in the H₂O ligand is slow enough so that two peaks are seen at −80 °C. The exchange with free H₂O makes a quantitative estimate of the H/H′ exchange barrier in the complex difficult to make but lineshape analysis shows it is close to 9 kcal mol⁻¹.

How to isolate an HF complex?. For G = H the HF binding energy is calculated to be 18.0 kcal mol⁻¹, but for G = NH₂ it is 28.2 kcal mol⁻¹. The hydrogen bonding ability of NH₂ therefore stabilizes the HF complex. Surprisingly, the hydrogen bonding group has almost no influence on the binding of H₂O since the binding energy of H₂O is 24.9 kcal mol⁻¹ for G = NH₂ and 23.3 kcal mol⁻¹ for G = H. The result is a complete change in the equilibrium between free and coordinated HF (eqn. 2 vs. 3), ΔE  =  +3.3 kcal mol⁻¹.

Thermodynamically, the HF complex is more stable than the H₂O complex and is the favored species at low temperature (G = NH₂). This is in full agreement with experiment where the HF complex is observed at low temperature and dissociates upon warming. The enhanced stability of the HF complex is thus caused by the N···H–F hydrogen bond.

The special topology of the binding cavity has two major consequences. The size is perfectly suited for HF hydrogen bonding (F–H–N angle of 175°), but less favorable for H₂O (O–H–N angle of 159°). The thermodynamic preference for the HF complex is thus enhanced for G = NH₂ both by making the HF complex more stable and making the H₂O complex less stable.

By its design, the binding cavity protects the acidic proton from external reagent and enhances the kinetic stability of the complex. The key to isolating an HF complex would be to build a binding cavity with somewhat stronger hydrogen bonding capabilities that also sterically protects the HF molecule from any incoming reagent. One possibility is a binding cavity with a nitrogen lone pair pointing towards the binding site. A bq with a fused pyridine ring (see below) should be perfect in this respect. Preliminary studies of the HF complex of this system gave a binding energy of 35.2 kcal mol⁻¹. Thus the hydrogen bond in this system is stronger than in 4N and experimental work at Yale is underway to synthesize and try to isolate and structurally characterize this HF complex.⁴⁵

Conclusion

In this paper we predict the structure of a new type of complex that has proved too unstable for X-ray crystallography study and suggest a ligand system that might better stabilize it for future isolation. We have also shown by DFT calculations how hydrogen bonding can be a very powerful and sensitive tool to tune structure and reactivity in organometallic chemistry. With appropriate ligand design, it becomes possible to stabilize unusual species like HF selectively while not stabilizing the corresponding aqua complex even though it has the same structure. We find hydrogen bonding facilitates several dynamic processes. Calculations have shown how strong hydrogen bonding in one TS allows fast exchange of NH₂.

Acknowledgements

This work was supported by the U.S. NSF, the Université de Montpellier and the CNRS. E. C. would like to thank the RSC for a generous travel grant. R.H.C. thanks the University of Montpellier for a visiting professorship. We are grateful to Dong-Heon Lee for checking the NMR spectroscopic properties of the aqua complex 2N.

References and notes

1. R. C. Cohen and R. J. Saykally, J. Chem. Phys., 1993, 98, 6007.
2. B. E. Smart, Mol. Struct. Energy, 1986, 3, 141.
3. D. Braga, F. Grepioni and G. R. Desiraju, Chem. Re[italic v]., 1998, 98, 1375.
4. D. Braga, F. Grepioni and G. R. Desiraju, J. Organomet. Chem., 1997, 548, 33.
5. L. Brammer, D. Zhao, F. T. Ladipo and J. Braddockwilking, Acta Crystallogr., Sect. B: Struct. Sci., 1995, 51, 632.
6. M. J. Calhorda, Chem. Commun., 2000, 801.
7. E. Peris, J. C. Lee, Jr and R. H. Crabtree, J. Chem. Soc., Chem. Commun., 1994, 2573.
8. E. Peris, J. C. Lee, Jr., J. R. Rambo, O. Eisenstein and R. H. Crabtree, J. Am. Chem. Soc., 1995, 117, 3485.
9. C. Walsh, Enzymatic Reaction Mechanisms, Freeman, San Francisco, 1979..
10. R. H. Crabtree, M. Lavin and L. Bonneviot, J. Am. Chem. Soc., 1986, 108, 4032.
11. M. Lavin, E. M. Holt and R. H. Crabtree, Organometallics, 1989, 8, 99.
12. D.-H. Lee, B. P. Patel, E. Clot, O. Eisenstein and R. H. Crabtree, Chem. Commun., 1999, 297.
13. B. P. Patel and R. H. Crabtree, J. Am. Chem. Soc., 1996, 118, 13105.
14. D.-H. Lee, H.-J Kwon, B. P. Patel, L. M. Liable-Sands, A. L. Rheingold and R. H. Crabtree, Organometallics, 1999, 18, 1615.
15. R. Kuhlman, Coord. Chem. Re[italic v]., 1997, 167, 205.
16. R. J. Kulawiec and R. H. Crabtree, Coord. Chem. Re[italic v]., 1990, 99, 89.
17. Z. Mazej, K. Borrmann, K. Lutar and B. Žemva, Inorg. Chem., 1998, 37, 5912.
18. V. J. Murphy, T. Hacall, J. Y. Chen and G. Parkin, J. Am. Chem. Soc., 1996, 118, 7428.
19. M. K. Whittlesey, R. N. Perutz, B. Greener and M. H. Moore, Chem. Commun., 1997, 187.
20. During the completion of this work we became aware of the synthesis of a new bifluoride complex: D. C. Roe, W. J. Marshall, F. Davidson, P. D. Soper and V. V. Grushin, Organometallics, 2000, 19, 4575.
21. F. Maseras, A. Llédos, E. Clot and O. Eisenstein, Chem. Re[italic v]., 2000, 100, 601.
22. M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, V. G. Zakrzewski, J. A. Montgomery, R. E. Stratmann, J. C. Burant, S. Dapprich, J. M. Millam, A. D. Daniels, K. N. Kudin, M. C. Strain, O. Farkas, J. Tomasi, V. Barone, M. Cossi, R. Cammi, B. Mennucci, C. Pomelli, C. Adamo, S. Clifford, J. Ochterski, G. A. Petersson, P. Y. Ayala, Q. Cui, K. Morokuma, D. K. Malick, A. D. Rabuck, K. Raghavachari, J. B. Foresman, J. Cioslowski, J. V. Ortiz, B. B. Stefanov, G. Liu, A. Liashenko, P. Piskorz, I. Komaromi, G. Gomperts, R. L. Martin, D. J. Fox, T. Keith, M. A. Al-Laham, C. Y. Peng, A. Nanayakkara, C. Gonzalez, M. Challacombe, P. M. W. Gill, B. G. Johnson, W. Chen, M. W. Wong, J. L. Andres, M. Head-Gordon, E. S. Replogle and J. A. Pople, GAUSSIAN 98, Revision A.7, Gaussian, Inc., Pittsburgh, PA, 1998..
23. A. D. Becke, J. Chem. Phys., 1993, 98, 5648.
24. J. P. Perdew and Y. Wang, Phys. Re[italic v]. B, 1992, 82, 284.
25. D. Andrae, U. Häußermann, M. Dolg, H. Stoll and H. Preuß, Theor. Chim. Acta, 1990, 77, 123.
26. A. Bergner, M. Dolg, W. Küchle, H. Stoll and H. Preuß, Mol. Phys., 1999, 30, 1431.
27. C. A. Tolman, J. Am. Chem. Soc., 1970, 92, 2953.
28. A. Rosa, A. W. Ehlers, E. J. Baerends, J. G. Snijders and G. te Velde, J. Phys. Chem., 1996, 100, 5690.
29. H. Taube, Coord. Chem. Re[italic v]., 1978, 26, 33.
30. R. H. Crabtree, P. C. Demou, D. Eden, J. M. Mihelcic, C. Parnell, J. M. Quirk and G. E. Morris, J. Am. Chem. Soc., 1982, 104, 6994 and references cited in this paper.
31. D. Milstein, J. C. Calabrese and I. D. Williams, J. Am. Chem. Soc., 1986, 108, 6387.
32. R. C. Stevens, R. Bau, D. Milstein, O. Blum and T. F. Koetzle, J. Chem. Soc., Dalton Trans., 1990, 1429.
33. D. Braga, F. Grepioni, E. Tedesco, M. J. Calhorda and P. E. M. Lopes, New J. Chem., 1999, 23, 129.
34. J. C. Lee, Jr, A. L. Rheingold, B. Muller, P. S. Pregosin and R. H. Crabtree, J. Chem. Soc., Chem. Commun., 1994, 1021.
35. L. S. Van der Sluys, J. Eckert, O. Eisenstein, J. H. Hall, J. C. Huffman, S. A. Jackson, T. F. Koetzle, G. J. Kubas, P. J. Vergamini and K. G. Caulton, J. Am. Chem. Soc., 1990, 112, 4831.
36. The long Ir–F bond length is similar to the Ag–F bond distance in Ag···FH⁺ (2.403 Å) computed by Chattaraj and Schleyer at the MP2/6-311 + G** level.³⁷.
37. P. K. Chattaraj and P. von Rague Schleyer, J. Am. Chem. Soc., 1994, 116, 1067.
38. K. Gruet, R. H. Crabtree, D.-H. Lee, L. M. Liable-Sands and A. L. Rheingold, Organometallics, 2000, 19, 2228.
39. R. H. Crabtree, P. E. M. Siegbahn, O. Eisenstein, A. L. Rheingold and T. F. Koetzle, Acc. Chem. Res., 1996, 29, 348.
40. F. Basolo and R. G. Pearson, Mechanism of Inorganic Reactions, Wiley, New York, 1958, p. 153..
41. B3PWP1/6-31G** optimal value for free HF for comparison with the calculated value in the complex. This value compares well with the experimental value of 0.9168 Å as deduced from IR spectra of HF in rare-gas matrices.⁴².
42. M. G. Mason, W. G. von Holle and D. W. Robinson, J. Chem. Phys., 1971, 54, 3491.
43. R. F. W. Bader, Atoms in Molecules, Clarendon Press, Oxford, 1990. We have used the AIMPAC package from Bader's group available on the internet at http://www.chemistry.mcmaster.ca/aimpac..
44. D. Cremer and E. Kraka, Angew. Chem., Int. Ed. Engl., 1984, 23, 627.
45. R. H. Crabtree and T. Dubé, work in progress..

---