Wigner function approach to the quantum Brownian motion of a particle in a potential
Abstract
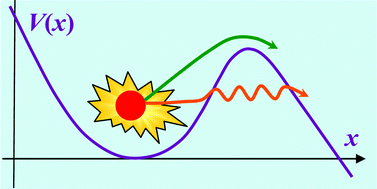
Recent progress in our understanding of quantum effects on the Brownian motion in an external potential is reviewed. This problem is ubiquitous in physics and chemistry, particularly in the context of decay of metastable states, for example, the reversal of the magnetization of a single domain ferromagnetic particle, kinetics of a superconducting tunnelling junction, etc. Emphasis is laid on the establishment of master equations describing the diffusion process in phase space analogous to the classical Fokker–Planck equation. In particular, it is shown how Wigner’s [E. P. Wigner, Phys. Rev., 1932, 40, 749] method of obtaining quantum corrections to the classical equilibrium Maxwell–Boltzmann distribution may be extended to the dissipative non-equilibrium dynamics governing the quantum Brownian motion in an external potential V(x), yielding a master equation for the Wigner distribution function W(x,p,t) in phase space (x,p). The explicit form of the master equation so obtained contains quantum correction terms up to o(ℏ4) and in the classical limit, ℏ → 0, reduces to the classical Klein–Kramers equation. For a quantum oscillator, the method yields an evolution equation coinciding in all respects with that of Agarwal [G. S. Agarwal, Phys. Rev. A, 1971, 4, 739]. In the high dissipation limit, the master equation reduces to a semi-classical Smoluchowski equation describing non-inertial quantum diffusion in configuration space. The Wigner function formulation of quantum Brownian motion is further illustrated by finding quantum corrections to the Kramers escape rate, which, in appropriate limits, reduce to those yielded via quantum generalizations of reaction rate theory.

 Please wait while we load your content...
Please wait while we load your content...