Consolidation of strong colloidal gels under arbitrary compressive loadings

Abstract

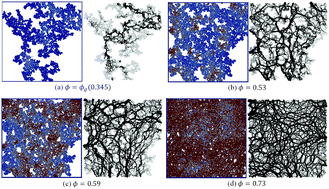
Although highly successful, classical constitutive theories for the consolidation of strong colloidal gels are limited to one-dimensional (1D) uniaxial consolidation. Many consolidation applications are inherently multidimensional and there currently exists little understanding and no constitutive theory for how strong colloidal gels consolidate under arbitrary compressive loadings. In this study, we address this shortcoming by considering the consolidation mechanics of strong colloidal gels under arbitrary compressive loadings via 2D DEM biaxial simulations of assemblies of cohesive frictional particles. We show that although particle-scale consolidation differs significantly between uniaxial and isotropic consolidation, the maximum normal stress during consolidation is a unique function of the volumetric strain and hence the concentration of the solids phase. We use these insights to develop a generalised constitutive model for the macroscopic compressive rheology under arbitrary compressive loadings that is consistent with the classical constitutive model for uniaxial consolidation. Surprisingly, we find that this generalized constitutive model can predict multidimensional consolidation under arbitrary compressive loadings without need for further characterisation beyond uniaxial consolidation. These results provide significant insights into the consolidation of strong colloidal gels and facilitate prediction of multi-dimensional consolidation over a wide range of applications, and so represents an initial foray toward the development of a tensorial rheology of strong colloidal gels.