Dynamics of droplets on cones: self-propulsion due to curvature gradients
Abstract
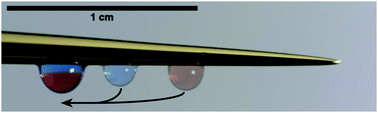
We study the dynamics of droplets driven by a gradient of curvature, as may be achieved by placing a drop on the surface of a cone. The curvature gradient induces a pressure gradient within the drop, which in turn leads to spontaneous propulsion of the droplet. To investigate the resulting driving force we perform a series of experiments in which we track a droplet's displacement, s, from the apex of a cone whose surface is treated to exhibit near-zero pinning effects. We find an s ∼ t1/4 scaling at sufficiently late times t. To shed light upon these dynamics, we perform an asymptotic calculation of the equilibrium shape of a droplet on a weakly curved cylinder, deriving the curvature-induced force responsible for its propulsion. By balancing this driving force with viscous dissipation, we recover a differential equation for the droplet displacement, whose predictions are found to be in good agreement with our experimental results.


 Please wait while we load your content...
Please wait while we load your content...