Approaching the Yablonovitch limit with free-floating arrays of subwavelength trumpet non-imaging light concentrators driven by extraordinary low transmission†
Abstract
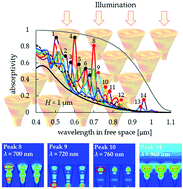
Metamaterials based on arrays of subwavelength dielectric structures have recently proved to be a viable research tool towards the realization of various photonic devices. In the current study we introduce a new approach towards efficient light trapping and broadband absorption of solar radiation based on silicon surface arrays composed of subwavelength trumpet non-imaging light concentrators (henceforth, trumpet arrays). In geometrical optics, a three-dimensional trumpet non-imaging light concentrator is a hyperboloid of revolution with an ideal light concentration ratio. We use finite-difference time-domain electromagnetic calculations to examine the optical response of an infinite cubic-tiled substrate-less silicon trumpet array under normal illumination. The absorptivity spectra of trumpet arrays are characterized by strong absorption peaks, some of which are just below the Yablonovitch limit. The enhanced light trapping is attributed solely to the efficient occupation of the array Mie modes, and we show absorption enhancement at near infrared that is an order of magnitude higher than that of the optimized nanopillar (NP) arrays. We show superior broadband absorption of solar radiation in trumpet arrays (with unoptimized geometry) compared with that of the optimized NP arrays (∼26% enhancement). The higher optical absorption in the trumpet array is governed by low transmissivity, in contrast to the NP array in which the absorption is governed by low reflectivity. Finally, we show that low reflectivity in trumpet arrays is governed by modal excitation at the upper part of the trumpets (which is also supported by the weak dependency of the reflectivity on the array height), whereas the transmissivity is governed by modal excitation at the lower part of the trumpets.


 Please wait while we load your content...
Please wait while we load your content...