Reporting measurement uncertainty and coverage intervals near natural limits
Abstract
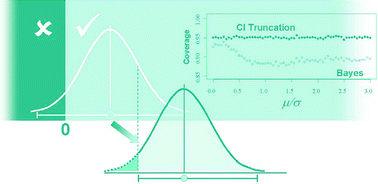
Different methods of treating data which lie close to a natural limit in a feasible range, such as zero or 100% mass or mole fraction, are discussed and recommendations made concerning the most appropriate. The methods considered include discarding observations beyond the limit, shifting observations to the limit, truncation of a classical confidence interval based on Student's t (coupled with shifting the result to the limit if outside the feasible range), truncation and renormalisation of an assumed normal distribution, and the maximum density interval of a Bayesian estimate based on a normal measurement distribution and a uniform prior within the feasible range. Based on consideration of bias and simulation to assess coverage, it is recommended that for most purposes, a confidence interval near a natural limit should be constructed by first calculating the usual confidence interval based on Student's t, then truncating the out-of-range portion to leave an asymmetric interval and adjusting the reported value to within the resulting interval if required. It is suggested that the original standard uncertainty is retained for uncertainty propagation purposes.

 Please wait while we load your content...
Please wait while we load your content...