Synthesis, crystal and electronic structure, and optical properties of two new chalcogenide-iodides: Ba₃Q₄I₂ (Q = S, Se)

Abstract

Two new ternary chalcogenide-iodides, Ba₃S₄I₂ and Ba₃Se₄I₂, were synthesized through high-temperature solid-state reactions, and their structures were determined via single-crystal X-ray diffraction. Both compounds are isostructural and crystallize in the monoclinic space group C2/c (no. 15) with unit cell parameters of a = 14.507(4)/15.080(7) Å, b = 10.104(3)/10.400(5) Å, c = 8.206(2)/8.383(4) Å, β = 101.847(4)/103.206(8)°, and Z = 4 for Ba₃S₄I₂/Ba₃Se₄I₂, respectively. The crystal structure of Ba₃Q₄I₂ (Q = S, Se) is constructed from Q₂²⁻ dumbbells and isolated I¹⁻ anions, which are surrounded by Ba²⁺ cations. According to UV/Vis spectroscopy, Ba₃S₄I₂ is a semiconductor with a bandgap of 2.45(5) eV. Quantum-chemical calculations predict that Ba₃S₄I₂ and Ba₃Se₄I₂ are wide bandgap semiconductors with bandgaps of 2.50 and 2.06 eV, respectively. Electron localization function analysis of chemical bonding indicates covalent interactions in the Q₂²⁻ dumbbells.

---

Kirill Kovnir

Kirill Kovnir is an Assistant Professor at the University of California, Davis. He was born in Kirovograd, Ukraine. He studied chemistry and received a Ph.D. at the Lomonosov Moscow State University with Prof. A. V. Shevelkov scrutinizing inverse clathrates. Afterwards he was tunneling between Max Planck Institute for Chemical Physics of Solids in Dresden, and Fritz Haber Institute of the Max Planck Society in Berlin, exploring the potential of intermetallic compounds in heterogeneous catalysis supervised by Prof. Yu. Grin and Prof. R. Schlögl. In 2008 he moved to Florida State University where he acquired a comprehensive knowledge of magnetism of complex solids under the guidance of Prof. M. Shatruk. Kirill's research interests are in the broad field of solid state and materials chemistry. Research in his group is focused on synthesis of novel thermoelectric, superconducting, magnetic, and low-dimensional materials and exploring their crystal structures, chemical bonding, and physical properties. Understanding the structure–property relationship is a key to the rational design of such materials.

---

Introduction

Metal chalcogenide-halides exhibit unique structural chemistry and properties that are different from both metal chalcogenides and metal halides. The recent discoveries of giant Rashba-type splitting in BiTeI,^1,2 spin density waves in La₂TeI₂,³ high ionic conductivity and excellent thermoelectric properties in Ag–Te–X compounds (X = Cl, Br) are just few examples of the diversity in metal chalcogenide-halides.^4–8 In contrast to the ternary rare-earth- or transition metal-chalcogenide-halides, no ternary alkaline-earth-containing sulfide- or selenide-halides have been reported.^9–11 Only a few quaternary or higher order compounds have been reported in literature, such as Ba₄Fe₂S₄I₅,¹² Ca₂LaGeS₄Cl₃,¹³ and ABa₃Ga₅Se₁₀Cl₂ (A = Cs, Rb, K).¹⁴ Systematic investigations of the alkaline-earth metal–chalcogen–halogen systems may reveal new compounds and further rationalize the relationship between their structures and properties. In the current work, we report the first examples of two ternary compounds, Ba₃S₄I₂ and Ba₃Se₄I₂, which were synthesized through high-temperature reactions of elements. Detailed characterizations of the crystal structure, optical properties, and the electronic structure and bonding are reported.

Experimental

Synthesis

All preparation processes were handled in an argon-filled glovebox with the O₂ level <1 ppm. All starting materials were commercial grade and used as received: Ba (Sigma Aldrich, 99.9%), I₂ (Alfa Aesar, resublimed crystals, 99.9985%), S (Alfa Aesar, pieces, 99.999%), and Se (Alfa Aesar, powder 99.999%).

Polycrystalline Ba₃Q₄I₂ (Q = S, Se) samples were synthesized via two-step high-temperature reactions. In the first step, the reactants were loaded in stoichiometric ratios Ba : Q : I = 3 : 4 : 2 into carbonized silica ampoules, evacuated, and flame-sealed. The ampoules were placed in muffle furnaces and heated from room temperature to 1073 K over a period of 20 hours, and then annealed at this temperature for 20 h, after which the furnace was turned off and the ampoules were cooled to room temperature. In the second step, the ampoules were opened in a glovebox, ground, resealed inside evacuated carbonized silica ampoules, and heated to 1073 K with the same heating rate, and annealed at 1073 K for an extended period of time, 144 h. The polycrystalline Ba₃S₄I₂ sample has a light yellow color, while Ba₃Se₄I₂ is dark red. The Ba₃S₄I₂ sample was almost phase pure with a tiny admixture of BaS according to powder X-ray diffraction (Fig. S1†), while the Ba₃Se₄I₂ samples always contained admixtures of mainly BaSe and BaSe₂. All our attempts to synthesize single-phase samples of Ba₃Se₄I₂ by varying the temperature profile were unsuccessful. Both Ba₃Se₄I₂ and Ba₃Se₄I₂ are highly air and moisture sensitive, and will decompose in a few minutes under exposure to ambient atmosphere. Long time (2–3 months) storage of Ba₃S₄I₂ sample in the glovebox also resulted in partial sample decomposition.

X-ray powder diffraction

Powder X-ray diffraction (XRD) was carried out on a Rigaku Miniflex 600 diffractometer employing Cu-K_α radiation. Air-sensitive holders with Be or Kapton windows were used to prevent the decomposition of the samples during data collection.

Single crystal X-ray diffraction

Due to the air-sensitive nature of Ba₃Q₄I₂ (Q = S, Se), crystal selection and cutting were performed inside the glove box. Suitable crystals were quickly transferred to the dry nitrogen flow in the single crystal X-ray diffractometer. The datasets were collected at 90 K under a N₂ stream using a Bruker AXS SMART diffractometer with Mo-K_α radiation and an APEX-II CCD detector. The datasets were recorded as ω-scans with a 0.4° step width and integrated with the Bruker SAINT software package.¹⁵ Multi-scan absorption corrections were applied.¹⁵ The solutions and refinements of the crystal structures were carried out using the SHELX suite of programs.¹⁶ The final refinements were performed using anisotropic atomic displacement parameters for all atoms. A summary of pertinent information related to unit cell parameters, data collection, and refinements is provided in Table 1 and the atomic parameters and interatomic distances are provided in Tables 2 and 3. Further details of the crystal structure determination may be obtained from Fachinformations-zentrum Karlsruhe, D-76344 Eggenstein-Leopoldshafen, Germany, by quoting the depository numbers CSD-430355 (Ba₃S₄I₂) and CSD-430356 (Ba₃Se₄I₂).

Table 1 Selected crystal data and structure refinement parameters for Ba₃Q₄I₂

Empirical formula	Ba3S4I2	Ba3Se4I2
a R 1 = ∑||Fo| − |Fc||/∑|Fo|; wR2 = [∑[w(Fo^2 − Fc^2)^2]/∑[w(Fo^2)^2]]^1/2, and w = 1/[σ^2Fo^2 + (A·P)^2 + B·P], P = (Fo^2 + 2Fc^2)/3; A and B are weight coefficients.
Formula weight	794.06 g mol^−1	947.00 g mol^−1
CSD number	430 355	430 356
Temperature	90(2) K
Radiation, wavelength	Mo-Kα, 0.71073 Å
Crystal system	Monoclinic
Space group	C2/c (no. 15)
Unit cell dimensions	a = 14.507(4) Å	a = 15.080(7) Å
	b = 10.104(3) Å	b = 10.400(5) Å
	c = 8.206(2) Å	c = 8.383(4) Å
	β = 101.847(4)°	β = 103.206(8)°
Unit cell volume	1177.3(5) Å^3	1280.0(11) Å^3
Z	4
Density (calc.)	4.48 g cm^−3	5.09 g cm^−3
Absorption coefficient	15.81 mm^−1	25.27 mm^−1
Goodness-of-fit	1.07	1.05
Final R indices^a [I > 2σ(I)]	R 1 = 0.029	R 1 = 0.040
	wR2 = 0.051	wR2 = 0.075
Final R indices^a [all data]	R 1 = 0.040	R 1 = 0.057
	wR2 = 0.056	wR2 = 0.083

---

Table 2 Refined atomic coordinates and equivalent displacement parameters for Ba₃Q₄I₂

Atom	Wyckoff	x/a	y/b	z/c	S.O.F.	U eq ^a (Å^2)
a U eq is defined as one third of the trace of the orthogonalized Uijtensor.
Ba 3 S 4 I 2
Ba1	8f	0.32778(2)	0.08927(3)	0.25914(3)	1	0.00547(7)
Ba2	4e	0	0.17704(4)	1/4	1	0.00585(9)
I1	8f	0.36677(2)	0.40252(3)	0.07541(4)	1	0.00734(8)
S1	8f	0.20128(8)	0.29103(12)	0.3998(2)	1	0.0073(2)
S2	8f	0.46625(8)	0.0928(1)	0.0035(2)	1	0.0067(2)
Ba 3 Se 4 I 2
Ba1	8f	0.32777(5)	0.09615(7)	0.25626(9)	1	0.0114(2)
Ba2	4e	0	0.1639(1)	1/4	1	0.0119(3)
I1	8f	0.36657(6)	0.40283(8)	0.0708(1)	1	0.0137(2)
Se1	8f	0.19701(8)	0.2952(1)	0.3862(2)	1	0.0115(3)
Se2	8f	0.46253(8)	0.1015(1)	−0.0002(2)	1	0.0113(3)

---

Table 3 Selected interatomic distances (Å) in Ba₃Q₄I₂

Atom pair	Distance (Å)	Atom pair	Distance (Å)
Ba 3 S 4 I 2
Ba1–S1	3.119(1)	Ba2–S1 × 2	3.145(1)
Ba1–S1	3.156(1)	S2 × 2	3.224(1)
Ba1–S1	3.276(1)	I1 × 2	3.5136(8)
Ba1–S2	3.128(1)	I1 × 2	3.6921(7)
Ba1–S2	3.188(1)	S1–S1	2.107(2)
Ba1–S2	3.215(1)	S2–S2	2.121(2)
Ba1–I1	3.5111(8)
Ba1–I1	3.6003(8)
Ba 3 Se 4 I 2
Ba1–Se1	3.216(2)	Ba2–Se1 × 2	3.232(2)
Ba1–Se1	3.303(2)	Se2 × 2	3.347(2)
Ba1–Se1	3.341(2)	I1 × 2	3.506(2)
Ba1–Se2	3.260(2)	I1 × 2	3.773(2)
Ba1–Se2	3.279(2)	Se1–Se1	2.384(3)
Ba1–Se2	3.351(2)	Se2–Se2	2.394(3)
Ba1–I1	3.526(2)
Ba1–I1	3.654(2)

---

Diffuse reflectance spectroscopy

UV-Vis diffuse reflectance spectra of Ba₃S₄I₂ were recorded using a Thermo Scientific Evolution 220 Spectrometer equipped with an integrating sphere. The reflectance data were converted to the Kubelka–Munk function, f(R) = (1 − R)²(2R)⁻¹. The sample was sealed inside a polypropylene bag under Ar atmosphere to prevent exposure to ambient conditions. An empty polypropylene bag was used for the baseline scan.

Quantum-chemical calculations

The electronic structure calculations and bonding analyses were carried out using the tight binding, linear muffin-tin orbital, atomic sphere approximation (TB–LMTO–ASA) program.¹⁷ The Barth–Hedin exchange potential was employed for the LDA calculations. The radial scalar-relativistic Dirac equation was solved to obtain the partial waves. A basis set containing Ba(6s,5d,4f), I(5p), and S(3s,3p)/Se(4s,4p) orbitals with downfolded Ba(6p) and I(6s,5d) orbitals were used for Ba₃S₄I₂ and Ba₃Se₄I₂, respectively. The density of states (DOS), band structure, and electron localization function (ELF, η)^18–21 were calculated after converging the total energies on a k-mesh grid with 12 × 12 × 12 points with 476 irreducible k-points. The Paraview program was used to visualize the ELF isosurfaces.²²

Results and discussion

Structure description

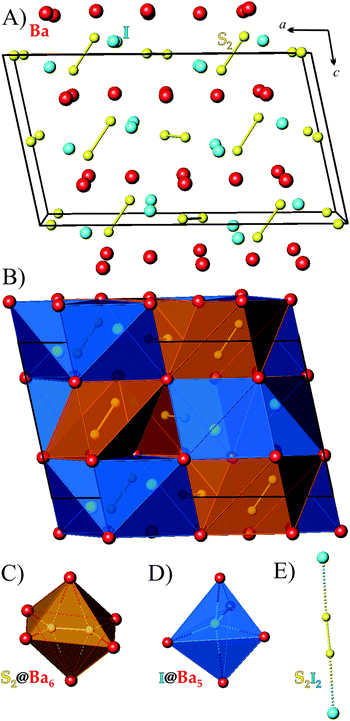
Both title compounds are isostructural and crystallize in the monoclinic space group C2/c (no. 15). For clarity only the crystal structure of Ba₃S₄I₂ is discussed (Fig. 1).

Fig. 1 The crystal structure of Ba₃S₄I₂: (A) general view of the unit cell; (B) polyhedral representation of the unit cell; (C) distorted Ba₆ octahedron around the S₂²⁻ dumbbell; (D) distorted Ba₅ trigonal bipyramid around I¹⁻; (E) a nearly linear S₂I₂ fragment. Ba: red; I: cyan; S: yellow. Unit cell is shown as black lines.

In the asymmetric unit of Ba₃S₄I₂ there are two Ba, one I, and two S sites that are crystallographically independent. All of the sulfur atoms are paired forming S₂²⁻ dumbbells (Fig. 1). Each sulfur atom is additionally coordinated by four Ba atoms forming a distorted tetrahedron. Two such tetrahedra share a common edge in such a way that the S₂²⁻ dumbbells reside inside distorted Ba₆ octahedra (orange polyhedra in Fig. 1B and C). Each iodine atom is surrounded by five Ba atoms forming a distorted trigonal bipyramid (blue polyhedra in Fig. 1B and D). Both types of Ba atoms are surrounded by a distorted capped trigonal prisms composed of eight or nine atoms: Ba1 is surrounded by six sulfur atoms and three I atoms and Ba2 is coordinated to four sulfur atoms and four I atoms (Fig. S2†).

In Ba₃S₄I₂, the S–S distances are 2.107(2) and 2.121(2) Å, which are close to the sum of the corresponding Pauling radius of S (1.05 Å)²³ and comparable to S–S distances in barium polysulfides, such as BaS₂ (2.12 Å),²⁴ BaS₃ (2.08 Å),²⁵ Ba₃Sb₂S₇ (2.09 Å),²⁶ and Sr₆Sb₆S₁₇ (2.09 Å).²⁷ The Ba–S distances in Ba₃S₄I₂ (3.11–3.28 Å) are similar to the distances found in Ba₃Sb₂S₇ (3.14–3.35 Å),²⁶ BaSb₂S₄ (3.13–3.35 Å),²⁸ and Ba₄Fe₂S₄I₅ (3.20–3.30 Å).¹² The Ba–I distances (3.51–3.69 Å) are similar to the Ba–I distances in Ba₄Fe₂S₄I₅, which contains I atoms that are coordinated by four or five Ba atoms (3.51–3.73 Å).¹²

The bond distances in Ba₃Se₄I₂ exhibit similar trends as those in Ba₃S₄I₂ (Table 3). The Se–Se bonds in the Se₂²⁻ dimers, 2.384(3) and 2.394(3) Å, are much longer than the S–S bonds. These distances are close to the sum of the corresponding Pauling radius of Se (1.20 Å)²³ and comparable to Se–Se distances in elemental selenium and other reported compounds: 2.45 Å in Se₁,²⁹ 2.36 Å in Se₆,³⁰ 2.33–2.35 Å in Se₈,³¹ 2.37 Å in Ba₃Sb₂Se₇,²⁶ and 2.37–2.42 Å in Ba₄Sb₄Se₁₁.³² The Ba–Se distances in Ba₃Se₄I₂, 3.22–3.35 Å, are similar to the distances in various Ba selenides, such as 3.29 Å in BaSe,³³ 3.30–3.56 Å in Ba₂Sb₂Se₅,³⁴ 3.29–3.41 Å in Ba₄Sb₄Se₁₁,³² and 3.26–3.60 Å in BaSb₂Se₄.³⁵ The Ba–I distances in Ba₃Se₄I₂, 3.51–3.77 Å, are longer than those in Ba₃S₄I₂, but are comparable to distances found in Ba₃SiI₂ (3.54–3.81 Å),³⁶ Ba₃GeI₂ (3.55–3.81 Å),³⁶ and Ba₄Fe₂S₄I₅ (3.51–3.73 Å).¹²

The shortest Q–I distances are nearly identical in both compounds with distances of 3.55 Å (Q = S) and 3.56 Å (Q = Se). Half of the Q₂²⁻ dumbbells are coordinated by iodine atoms forming almost linear Q₂I₂ fragments with angles of ∠I–Q–Q of 172.0° (Q = S) and 170.4° (Q = Se) (Fig. 1E), while the remaining Q₂²⁻ dumbbells are coordinated by Ba atoms only.

Ba₃Q₄I₂ (Q = S, Se) are the first reported ternary mixed anions alkaline-earth sulfide- or selenide-halides. The isolated I¹⁻ anions combined with Q₂²⁻ dumbbells, which have intrinsic covalent interactions, compensate the positive charge of the Ba²⁺ cations. Ba₃Q₄I₂ compounds exhibit two distict types of chemical bonding: covalent bonding in the Q₂²⁻ dumbbells and ionic bonding between cations and anions. Assuming +2 and −1 formal oxidation states for Ba and I, respectively, and a −1 oxidation state for all chalcogen atoms in the Q₂ dumbbells, total electroneutrality is achieved: (Ba²⁺)₃(Q₂²⁻)₂(I¹⁻)₂. The ionic interactions among the Ba²⁺, I⁻, and S₂²⁻ ions result in a charge-balanced compound making Ba₃S₄I₂ a large band gap semiconductor, which was predicted from electronic structure calculations. Optical measurements and the translucent yellow color of Ba₃S₄I₂ further support such a description. Mercury-thallium chalcogenides with compositions similar to the title compounds, Hg₃Q₄Tl₂, also crystallize in C2/c space group. In these compounds, the chalcogen atoms are well separated from each other as Q²⁻ anions and instead of I¹⁻ anions the Tl¹⁺ cations are present, maintaining total electroneutrality as (Hg²⁺)₃(Q²⁻)₄(Tl¹⁺)₂.³⁷

UV–Vis diffuse-reflectance spectroscopy

Solid-state UV-Visible Kubelka–Munk (KM) diffuse reflectance spectroscopy was employed to determine the bandgap of Ba₃S₄I₂. The UV–Vis spectrum exhibits apparent absorption edges around 490 nm (Fig. 2). According to the Tauc plots, the indirect and direct band gaps of Ba₃S₄I₂ are 2.45(5) and 2.6(1) eV, respectively (Fig. 2, bottom). The calculated value of the direct bandgap depends on the range selected for the linear fit, which resulted in high standard deviations. This result is in good agreement with the observed yellow-translucent color and calculated direct bandgap of 2.50 eV (vide infra). The combination of spectroscopic and computational approaches indicates that Ba₃S₄I₂ is a wide bandgap semiconductor.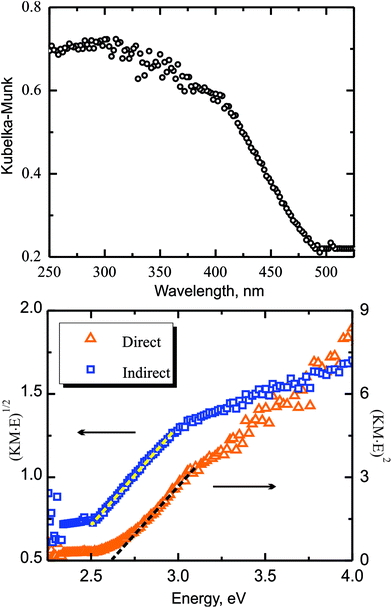

Fig. 2 (Top) Solid-state UV-Visible Kubelka–Munk diffuse reflectance spectrum of Ba₃S₄I₂. (Bottom) Tauc plots for allowed direct (orange triangles) and indirect (blue squares) transitions.

Electronic structure

Quantum chemical calculations show that both compounds are wide bandgap semiconductors with bandgaps of 2.50 and 2.06 eV for Ba₃S₄I₂ and Ba₃Se₄I₂, respectively (Fig. 3, left). Calculations suggest that Ba₃S₄I₂ is a direct bandgap semiconductor, while Ba₃Se₄I₂ is an indirect bandgap semiconductor. Ba₃S₄I₂ has direct bandgaps of 2.50 eV at both the L and Z points of the Brillouin zone. Unlike Ba₃S₄I₂, Ba₃Se₄I₂ has an indirect bandgap of 2.06 eV with a direct bandgap of slightly higher energy (2.18 eV) at the V point. The direct transitions for Ba₃Se₄I₂ at the L, Z, and Γ points of 2.20, 2.27, and 2.26 eV, respectively, are very similar in energy to the energy difference at the V point.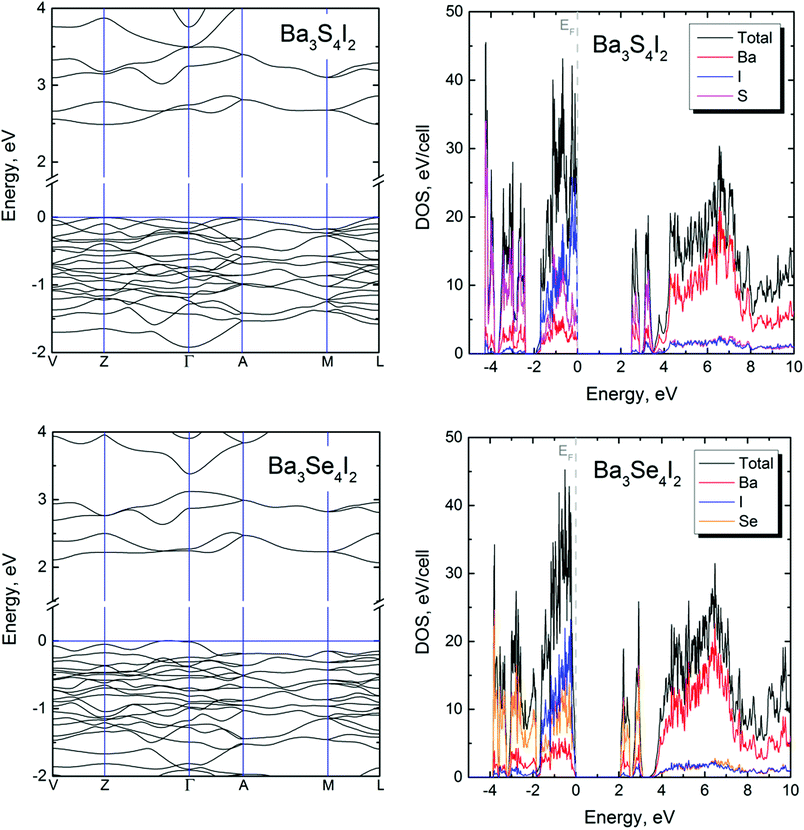

Fig. 3 Band structures (left) and density of states (DOS) (right) for Ba₃S₄I₂ (top) and Ba₃Se₄I₂ (bottom).

Elemental projections of the density of states diagram (Fig. 3, right) indicate that for both compounds, the states near the top of the valence band are composed from orbital contributions from all three elements, with dominating contributions from S (or Se) and I. Contributions from Ba orbitals dominate in the states at the conduction band at energies >+4 eV. The bottom of the conduction band is composed of two sharp peaks, which are essentially S (or Se) orbitals with minimal contributions from Ba and I.

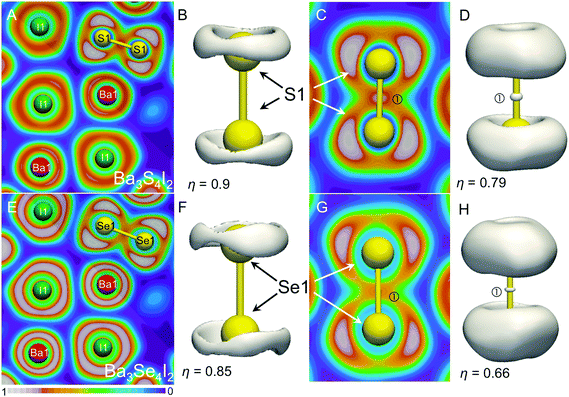
Chemical bonding in Ba₃Q₄I₂ was analyzed with the help of electron localization function (ELF) analysis. The bonding analysis confirmed the expected covalent Q–Q bonding for the Q₂ dumbbells (attractor ①) with torus-like attractors on the terminal Q atoms (Fig. 4). Similar torus-like distributions for ELF were found for the S₂²⁻ units in Ba₃Sb₂S₇.²⁶ According to Zintl or Lewis descriptions the Q¹⁻ atoms in the isolated Q₂²⁻ dumbbells should have three electron lone pairs, and the spatial arrangements of those lone pairs should conform with D_∞h local symmetry. The observed torus-like arrangement is in accordance with those expectations. Similar ELF distributions were observed for the three lone-pairs located on O atoms in the OH¹⁻ hydroxyl anions with C_∞h symmetry.³⁸ For the linear fragments with two terminal electron lone pairs, such as C₃⁴⁻, different, an umbrella-like ELF distribution was reported.³⁹ In turn, for linear Sb₃⁷⁻ and isoelectronic ClF₂¹⁻ where three electron lone pairs are expected on the central atoms, the ELF distribution is also torus-like.^20,40

Fig. 4 ELF slices and isosurfaces for Ba₃S₄I₂ (top) and Ba₃Se₄I₂ (bottom). (A and E) slices showing the ELF for Ba1, I, and the Q₂ dumbbells with the scale bar shown at the bottom left. (C and G) slices of the Q₂ dumbbells. (B, D, F, H) isosurfaces for the Q₂ dumbbell at different values of ELF, η, indicated in the figure.

The ELF slices show deep minima surrounding the Ba and I atoms indicating no covalent bonds between the ions. However, significant structuring of both iodine and barium cores were observed, indicating the participation of 5^th shell electrons of Ba and I in bonding interactions.

Conclusions

Two new ternary chalcogenide iodides, Ba₃S₄I₂ and Ba₃Se₄I₂, have been synthesized and structurally characterized. Both compounds crystallize in the monoclinic space group C2/c (no. 15). The crystal structure of these compounds is comprised of Q₂²⁻ dumbbells and isolated I¹⁻ anions surrounded by Ba²⁺ cations. Ba₃S₄I₂ is a wide bandgap semiconductor with an optical bandgap of 2.45(5) eV, which is supported by electronic band structure calculations. ELF analyses indicate covalent bonding within the Q₂²⁻ dumbbells as well as polarization of the barium and iodine 5^th electronic shells.

Acknowledgements

The authors would like to thank Prof. F. Osterloh for access to the UV-visible diffuse reflectance spectrometer. K. L. acknowledges the GAANN and ARCS fellowships. This research is supported by the U.S. Department of Energy, Office of Basic Energy Sciences, Division of Materials Sciences and Engineering under Award DE-SC0008931.

References

1. K. Ishizaka, M. S. Bahramy, H. Murakawa, M. Sakano, T. Shimojima, T. Sonobe, K. Koizumi, S. Shin, H. Miyahara, A. Kimura, K. Miyamoto, T. Okuda, H. Namatame, M. Taniguchi, R. Arita, N. Nagaosa, K. Kobayashi, Y. Murakami, R. Kumai, Y. Kaneko, Y. Onose and Y. Tokura, Nat. Mater., 2011, 10, 521–526.
2. J. S. Lee, G. A. H. Schober, M. S. Bahramy, H. Murakawa, Y. Onose, R. Arita, N. Nagaosa and Y. Tokura, Phys. Rev. Lett., 2011, 107, 117401.
3. M. Ryazanov, A. Simon and H. Mattausch, Inorg. Chem., 2006, 45, 10728–10733.
4. T. Nilges, S. Nilsen, A. Pfitzner, T. Doert and P. Böettcher, Chem. Mater., 2004, 16, 806–812.
5. T. Nilges, C. Dreher and A. Hezinger, Solid State Sci., 2005, 7, 79–88.
6. S. Lange and T. Nilges, Chem. Mater., 2006, 18, 2538–2544.
7. S. Lange, M. Bawohl, D. Wilmer, H. W. Meyer, H. D. Wiemhöefer and T. Nilges, Chem. Mater., 2007, 19, 1401–1410.
8. T. Nilges, O. Osters, M. Bawohl, J. L. Bobet, B. Chevalier, R. Decourt and R. Weihrich, Chem. Mater., 2010, 22, 2946–2954.
9. P. M. Carkner and H. M. Haendler, J. Solid State Chem., 1976, 18, 183–189.
10. C. M. Schurz, S. Frunder and T. Schleid, Eur. J. Inorg. Chem., 2013, 2923–2929.
11. M. Larres, I. Pantenburg and G. Meyer, Z. Anorg. Allg. Chem., 2013, 639, 2744–2747.
12. D. L. Gray, G.-J. Long, F. Grandjean, R. P. Hermann and J. A. Ibers, Inorg. Chem., 2008, 47, 94–100.
13. R. L. Gitzendanner and F. J. DiSalvo, Inorg. Chem., 1996, 35, 2623–2626.
14. P. Yu, L. J. Zhou and L. Chen, J. Am. Chem. Soc., 2012, 134, 2227–2235.
15. Bruker APEX2, Bruker AXS Inc., Madison, WI, 2005.
16. G. M. Sheldrick, Acta Crystallogr., Sect. A: Fundam. Crystallogr., 2008, 64, 112–122.
17. O. Jepsen, A. Burkhardt and O. K. Andersen, The Program TB-LMTO-ASA, Version 4.7, Max-Planck-Institut für Festkörperforschung, Stuttgart, Germany, 1999.
18. A. D. Becke and K. E. Edgecombe, J. Chem. Phys., 1990, 92, 5397–5403.
19. A. Savin, O. Jepsen, J. Flad, O. K. Andersen, H. Preuss and H. G. Von Schnering, Angew. Chem., 1992, 104, 186–188 ( Angew. Chem., Int. Ed. Engl. , 1992 , 31 , 187–188 ).
20. A. Savin, R. Nesper, S. Wengert and T. F. Fässler, Angew. Chem., 1997, 109, 1892–1918 ( Angew. Chem., Int. Ed. Engl. , 1997 , 36 , 1808–1832 ).
21. Yu. Grin, A. Savin and B. Silvi, in The Chemical Bond : Fundamental Aspects of Chemical Bonding, ed. G. Frenking and S. Shaik, Wiley-VCH, Weinheim, 2014, pp. 345–382.
22. (a) Paraview: Parallel visualization application, version 4.1.0 64 bit, Sandia National Labs, Kitware Inc, Los Alamos National Labs, USA; http://paraview.org; (b) A. I. Baranov, Visualization plugin for ParaView, Version 4.1.0, 2014.
23. L. Pauling, The Nature of the Chemical Bond, Cornell University Press, Ithaca, NY, 3rd edn, 1960.
24. I. Kawada, K. Kato and S. Yamaoka, Acta Crystallogr., Sect. B: Struct. Crystallogr. Cryst. Chem., 1975, 31, 2905–2906.
25. S. Yamaoka, J. T. Lemley, J. M. Jenks and H. Steinfink, Inorg. Chem., 1975, 14, 129–131.
26. J. Wang, K. Lee and K. Kovnir, Z. Anorg. Allg. Chem., 2015, 641, 1087–1092.
27. K.-S. Choi and M. G. Kanatzidis, Inorg. Chem., 2000, 39, 5655–5662.
28. G. Cordier, C. Schwidetzky and H. Schaefer, J. Solid State Chem., 1984, 54, 84–88.
29. Y. Akahama, M. Kobayashi and H. Kawamura, Phys. Rev. B: Condens. Matter, 1993, 47, 20–26.
30. Y. Miyamoto, J. Appl. Phys., 1980, 19, 1813–1819.
31. P. Cherin and P. Unger, Acta Crystallogr., Sect. B: Struct. Crystallogr. Cryst. Chem., 1972, 28, 313–317.
32. G. Cordier, R. Cook and H. Schäfer, Angew. Chem., 1980, 92, 310 ( Angew. Chem., Int. Ed. Engl. , 1980 , 19 , 324–325 ).
33. Y. B. Lyskova and A. V. Vakhobov, Inorg. Mater., 1975, 11, 1784–1785.
34. J. Wang, K. Lee and K. Kovnir, J. Mater. Chem. C, 2015, 3, 9811–9818.
35. G. Cordier and H. Schäfer, Z. Naturforsch., B: Anorg. Chem. Org. Chem., 1979, 34, 1053–1056.
36. S. Wengert and R. Nesper, J. Solid State Chem., 2000, 152, 460–465.
37. S. Johnsen, S. C. Peter, S. L. Nguyen, J.-H. Song, H. Jin, A. J. Freeman and M. G. Kanatzidis, Chem. Mater., 2011, 23, 4375–4383.
38. M. E. Tuckerman, D. Marx and M. Parrinello, Nature, 2002, 417, 925–929.
39. D. A. Lang, J. V. Zaikina, D. D. Lovingood, T. E. Gedris and S. E. Latturner, J. Am. Chem. Soc., 2010, 132, 17523–17530.
40. Y. Hu, J. Wang, A. Kawamura, K. Kovnir and S. M. Kauzlarich, Chem. Mater., 2015, 27, 343–351.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c5qi00225g

---