Frustrated van der Waals heterostructures

Abstract

Geometrical frustration results from the packing of constituents in a lattice, where the constituents have conflicting forces. The phenomenon is known in glass materials, and this work expands the concept of geometrical frustration into the realm of van der Waals two-dimensional materials. Using density functional theory with the r²SCAN + rVV10 exchange–correlation potential, we find a number of two-dimensional heterostructures with alternating strains, where one layer is strained and the adjacent layer is compressed. We adopted three structural stability criteria to find synthesisable candidate materials: phonon dispersion of the individual layers, comparing the thermodynamic stability of this class of materials, frustrated van der Waals heterostructures, with the non-frustrated counterparts, and ab initio molecular dynamics simulations. These criteria were applied to 7 frustrated van der Waals heterostructures, identifying one material that is potentially stable. We discuss possible fabrication pathways for creating this class of materials.

---

1 Introduction

The potential landscape of materials is complicated by geometrical frustration. Geometrical frustration is defined by conflicting forces that arise among neighboring components within a structure, leading to the emergence of nontrivial ground states. This phenomenon has been known in bulk metallic glasses,¹ colloidal glasses² and magnets.^3,4 Spin alignment in magnetic systems⁴ and suspension of colloidal particles^2,5 have demonstrated geometrical frustration in various settings. This work introduces a new type of bulk material frustration that is driven by conflicting strains in layered materials: frustrated van der Waals heterostructures (FvdWHs). Hybrid two-dimensional materials are typically synthesized by layering commensurate 2D materials on top of each other, where none of the layers suffer from lateral strains.⁶ In a 2D van der Waals (2DvdW) bilayer, straining one layer while leaving the other layer in its ground state will lead to an accumulation of energy that will be released mechanically by the strained layer, potentially curving the originally unstrained layer. With the image of a the basic freezer thermostat in mind, conflicting strains in bilayers is a common mechanism in flexible bilayers.⁷

Our work was motivated by the question: what if one layer was strained, while the other was compressed? Naively speaking, in such a configuration, one layer will laterally force the crystal to expand, while the other layer will force it to contract. We present a schematic for this setup in Fig. 1. Would such conflicting strain, or strain frustration, bring about equilibrium structures? That is, would strain frustration reduce the total energy of the unstrained (non-frustrated) bilayer? We attempt to answer this question by generating a number of hybrid 2DvdW materials and optimizing their atomic and lattice structures using density functional theory (DFT). To attain high accuracy in predicting the total energies of van der Waals systems, we apply the r²SCAN + rVV10 exchange–correlation potential,⁸ which combines the r²SCAN meta-GGA⁹ and the rVV10 van der Waals non-local correlation correction. The r²SCAN meta-GGA has been the method of choice for the online materials database Materials Project¹⁰ because of its accuracy advantage compared with SCAN and rSCAN. The combination r²SCAN + rVV10 has demonstrated particularly high accuracy for a range of 2D materials.⁸ The recent work by Kothakonda et al.¹¹ has shown that r²SCAN + rVV10 is generally recommended for the discovery of new layered materials.

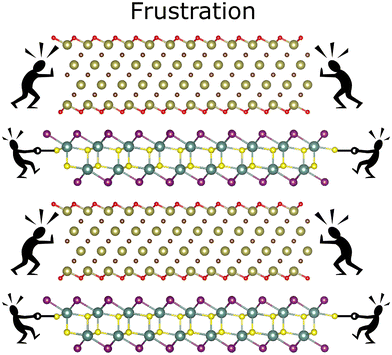Fig. 1 A schematic illustration of the structure of a frustrated van der Waals heterostructure: it is possible to achieve stability when alternating layers experience opposing strains.

We screened the C2DB database for highly stable monolayers (thermodynamically and dynamically stable) and re-optimized the monolayers using DFT as implemented in the VASP version 5.4.4 code within the r²SCAN meta-GGA exchange–correlation potential. All of our optimisation calculations are spin-polarized, with a plane-wave basis set cut-off of 520 eV, the energy tolerance of 10⁻⁶ eV, and the force tolerance of 10⁻² eV Å⁻¹. We searched for bilayers where the lattice mismatch is less than 1% for the creation of both the non-frustrated (zero strain) and the frustrated bilayers. In frustrated bilayers, one layer is strained by +5% while the other by −5%. We apply the following procedure to find pairs of frustrated and non-frustrated bilayers: we retrieve all pairs of 2D materials from the C2DB database that can form commensurate supercells with a lattice mismatch <1% for the unstrained layers, as well as the same layers with strains of +5% and −5%. The maximum size of the supercell for each layer in the bilayer is 5 × 5 to limit the computational complexity.

We applied three criteria to identify stable FvdWHs:

1. For each of the monolayers obtained thus far, we compute the phonon dispersion for the 2 × 2 using the finite difference approach, and compute the dynamical matrix and the phonon band structure using the phonopy package.¹² We then exclude monolayers that have imaginary modes within the Brillouin zone for strained and unstrained monolayer unit cells. The computed phonon band structures are displayed in Table S1 in the ESI,† both for the dynamically stable and unstable monolayers.

2. For the bilayers formed from dynamically stable monolayers, we have computed the difference in total energy/atom, ΔE_f–n, between the frustrated and the non-frustrated supercells, which is given by ΔE_f–n = E_f/n_f − E_n/n_n where E_f, E_n are the total energy of the frustrated and non-frustrated supercells, respectively, and n_f and n_n are the number of atoms in the frustrated and non-frustrated supercells, respectively. The results are displayed in Table 1.

Table 1 The difference in total energy/atom, ΔE_f–n (meV per atom), between the frustrated and the non-frustrated supercells; the binding energy between the two layers in frustrated, E^f_b (meV per atom), and the non-frustrated, Eⁿ_b (meV per atom); the electronic band gaps in the frustrated, E^f_g (eV), the non-frustrated, Eⁿ_g (eV), supercells; the optimised lattice constants in Layer 1, Layer 1 a_opt and Layer 1 b_opt, and in Layer 2, Layer 2 a_opt and Layer 2 b_opt; and the qualification of whether structural integrity is maintained after the AIMD simulation

Layer 1 (+5%)	Layer 2 (−5%)	ΔEf–n	E b ^f	E b ^n	E g ^f	E g ^n	Layer 1 aopt	Layer 1 bopt	Layer 2 aopt	Layer 2 bopt	AIMD
BaICl	Hf3Se2	−241	−60	−76	0	0	12.1	12.1	0.6	0.6	No
BiSI	Ti3C2O2	−182	−29	−54	0	0	10.8	10.9	−0.6	−0.5	No
YSI	Hf4C3O2	−180	−2	−37	0	0	10.7	10.7	−0.8	−0.7	Yes
BaICl	ZrSiSe	−169	−52	−64	0	0	12.2	12.2	0.6	0.6	No
BaICl	MnSe2	−123	−56	−75	0	0	9.5	9.5	−1.7	−1.7	No
PbSe2	Y2NCl2	−115	−42	−83	0	0	9.8	9.8	−1.5	−1.5	No
YSI	VSe2	+37	−30	−79	0	0	7.6	7.6	−3.4	−3.4	Yes

---

3. For the above bilayers, we have further scrutinised the stability of the materials by performing ab initio molecular dynamics (AIMD) simulations. We perform the AIMD simulations using the spin-unpolarised PBE GGA exchange correlation potential¹³ with a plane-wave basis set cut-off of 450 eV, and at the Γ point. The simulation temperature was 300 K, using the Nose–Hoover thermostat^14,15 and a time-step of 1 fs. We display the evolution of the temperature as a function of time for each of the bilayers is in Table S2 of the ESI.†

All of the monolayers in Table 1 are dynamically stable in the unstrained and ±5% strained states except for Ti₃C₂O₂, which is dynamically stable in the unstrained and +5% strained states, but is dynamically unstable in the −5% strained case, as shown in Table S1 in the ESI.† We have provided results for bilayers with dynamically unstable monolayers in Table S3 in the ESI.†

To examine the impact of frustration on the van der Waals force, we compute the van der Waals binding energy of a frustrated bilayer E^f_b per atom using the formula E^f_b = (E_f − E^f₁− E^f₂)/n where E^f₁ is the total energy of Layer 1, and E^f₂ is the total energy of Layer 2. Likewise, for the non-frustrated bilayers, Eⁿ_b is calculated using Eⁿ_b = (E_f − Eⁿ₁− Eⁿ₂)/n. The values of E^f_b and Eⁿ_b are displayed in Table 1.

Indeed, we find a number of FvdWHs that are energetically more stable than their corresponding non-frustrated structures. Table 1 displays the values of ΔE_f–n for the bilayers, showing that strain frustration can either enhance the thermodynamic stability (top part of Table 1) or reduce it (bottom part of Table 1). At the top of the table, frustration significantly contributes to the stability of the BaICl|Hf₃Se₂ bilayer, which has the lowest energy/atom (−241 meV per atom) and displayed in Fig. 2(a and b). The frustration did not have a large impact on the nature of the interlayer vdW binding, given that this binding in the frustrated case is −60 meV per atom compared to the value of −76 meV per atom in the non-frustrated case. However, frustration had varied consequences on the stability as well as the magnitude of the vdW binding in the rest of the materials in Table 1.

Fig. 2 The atomic structure of example stable bilayers. BaICl|Hf₃Se₂ frustrated (a) and non-frustrated (b), BaAgBrO|Sc₃N₂F₂ frustrated (c) and non-frustrated (d), and YSI|Hf₄C₃O₂ frustrated (e) and non-frustrated (f).

Starting from a frustrated configuration where both lattice constants a and b of Layer 1 is strained by +5% and Layer 2 by −5% (biaxial strain), the lattice optimisation of the frustrated bilayers resulted in deviation of the lattice parameters of the individual layers from the initial strained values. The strain on a and b lattice parameters of the individual layers (Layer 1 and Layer 2) after optimisation are displayed in Table 1, which we identify as a_opt and b_opt. Extreme deviations were observed in the case of BaICl|Hf₃Se₂ and BaICl|ZrSiSe, where BaICl, which was initially strained by +5%, was further stretched, or over-powered, by Layer 2. The fragility of BaICl can be observed in Fig. 2(a), showing that the excessive stretching has resulted in the breakdown of the lattice structure of BaICl. In the case of YSI|VSe₂, the two layers reached a trade-off strain that is close to the initial ±5%: the initially compressed layer released its compression by stretching the other layer, reaching a biaxial −3.4% strain, while the other layer reached a biaxial strain of +7.6%. Only the bilayer BaAgBrO|Sc₃N₂F₂ exhibited a deviation from biaxial strain: the strain on a was different from that on b for both layers. For the rest of the bilayers, Layer 1 was over-powered by Layer 2; the layer that was initially compressed has released its compression, resulting in over-straining the other layer.

The results in Table 1 show that strain frustration can enhance the thermodynamic stability while enhancing the interlayer binding, such as in the case of BaAgBrO|Sc₃N₂F₂, or it can enhance the thermodynamic stability while reducing the interlayer binding energy, such as in all other 6 cases. BaAgBrO|Sc₃N₂F₂, displayed in Fig. 2(c and d) is particularly interesting because the non-frustrated structure is slightly repulsive, where the interlayer binding energy is +16 meV per atom. However, by inspecting the frustrated and non-frustrated structures, the weakened interlayer binding could be caused by the significant structural disorder in BaICl, as shown in Fig. 2.

The overall result of Table 1 is the emergence of two stable FvdWHs: BaAgBrO|Sc₃N₂F₂ and YSI|Hf₄C₃O₂, which meet the three stability criteria. We display the structure of these two bilayers in Fig. 3.

Fig. 3 The atomic structure of the most stable bilayers in their frustrated state: (a) BaAgBrO|Sc₃N₂F₂ and (b) YSI|Hf₄C₃O₂, showing the temperature T and the duration of the AIMD simulation (t).

Is it possible to realise frustrated 2D materials? In flexible electronics, a recently implemented frustrated-bilayer structure has applied a similar concept: one wavey layer is a stretchable elastomer layer, which is pre-stretched before a 2D material is transferred to it.⁷ When the stretching is released, the combined bilayer develops an out-of-plane wavey structure. This wavey structure is essentially a frustrated bilayer in which the elastomer layer's tendency to contract is resisted by the target 2D material's tendency to expand. However, unlike the frustration examined in this work, the bilayer preserves it flat structure, except for a few cases where the structural integrity is compromised, such as in Fig. 2(a). For the frustrated bilayers in this work to be stacked, one 2D layer, layer A, must be pre-stretched by a biaxial strain of a% and then stacked on the pre-compressed second 2D layer, layer B, which is compressed by a biaxial strain of b% (noting that b = −a). Lateral compression of a 2D material (layer B) might lead to buckling, and hence a more suitable scheme would be to stack the pre-stretched layer (layer A) on an unstrained layer B, such as layer A is pre-stretched by a biaxial strain of a + |b|%. This setup assumes that layer A can actually be stretched by a + |b|%, which amounts to ∼10% for the bilayers in Table 1. Biaxial strain of MoS₂ up to 8% was reported by Blundo et al.,¹⁶ which is close to its rapture strain, Zhang et al.¹⁷ realised a strain of 8% the Zr₂CO₂ MXene structure, and a number of other strains and straining setups were reviewed in ref. 18. Deep elastic strain, which is overstraining 2D materials beyond ½ of the material's theoretical strength, was realised for graphene, hexagonal boron nitride, MoS₂ and WoS₂.¹⁹ Thus, it is technologically possible to overstrain a 2D layers by a + |b|% before binding it to another 2D layer. Note that, generally, a does not necessarily have to be equal to −b; the strain values a and b should be obtained by DFT computations, such as in the present work. Additionally, the layers do not necessarily have to be different; it might be possible that applying opposing strains to multilayer graphene or hexagonal boron nitride might result in a FvdWH. Also, different straining schemes, whether uniaxial, biaxial, or shear strains, should be examined. The fact that 6 out of 7 structures in Table 1 are more energetically stable when frustrated means that frustration can be a novel approach for creating new stable layered materials. Given that, in principle, the frustrated bilayer can have a different electronic bandgap from the unfrustrated bilayer, as examined in Fig. S1 and Table S3 of the ESI,† FvdWHs can be a platform for electronic bandgap tuning.

While the above setup can be feasible for stacking bilayers, it might not be directly applicable for bulk structures, as is the case in the structures predicted in the present work. Generally, the synthesis of hybrid 2DvdW bulk materials, or 2DvdW superlattices, is indeed more challenging than stacking 2DvdW bilayers.^20–24 A range of techniques have been developed over the last decade, with reported success in making graphene-based bulk 2DvdW materials. The key experimental procedure that is established as the ideal synthesis approach is the electrostatic layer-by-layer (LbL) assembly.^25–27 LbL assembly assumes that the two 2D layers have opposing electric polarisation, and the adsorption of the layers takes place in solution. Hence, it is not possible to control the strain of the constituent layers while in solution. There is a suite of alternative dry approaches for stacking or synthesising multilayers, as reviewed by Guo et al.²⁰ A particularly promising approach is mechanical stacking. Using a micromanipulation platform, one layer is transferred onto the other, and then they are left to self-assemble by the van der Waals interlayer force. Once they self-assemble, the top layer is detached. If the two layers should have opposing strains, then the micromanipulation platform should strain each of the two layers: the bottom layer must be strained, and the top layer must be strained during transfer and up until it is detached. Once both layers are detached, they should remain at their strained configurations. If the top layer remains strained after detachment, then the process can be repeated by stacking more strained layers on top of the detached bilayer. Hence, this approach can be tested by experimentalists for testing the stacking of the FvdWHs predicted in this work.

In conclusion, we explored a new type of layered heterostructures, frustrated van der Waals heterostructures (FvdWHs). A FvdWH is a layered material with alternating strains and compressions, where one layer is strained and the adjacent layer is compressed. Using density functional theory with the r²SCAN + rVV10 exchange–correlation potential, we explored the stability of 9 FvdWHs that are made from dynamically stable monolayers, and found 6 FvdWHs that are more thermodynamically stable with alternating strains and compressions than the corresponding material without any strains. Two of these materials were found to be maintain their structures after ab initio molecular dynamics simulations at 300 K. We presented a scheme for the potential stacking of strained/compressed 2D materials to build FvdWHs, and expect this class of materials to harbour novel physical and chemical phenomena.

Data availability

The structures explored in this work have been deposited in the public repository https://github.com/sheriftawfikabbas/hybrid_vdW_heterostructures. The POSCAR files of these structures, as well as the POSCAR files of the frustrated and non-frustrated bilayers that were simulated using AIMD, are provided in this repository.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This work was supported by computational resources provided by the Australian Government through the National Computational Infrastructure National Facility and the Pawsey Supercomputer Centre.

References

1. T. Egami, V. Levashov, R. Aga and J. Morris, Metall. Mater. Trans. A, 2008, 39, 1786 . ISSN 1073-5623, 1543-1940. https://link.springer.com/10.1007/s11661-008-9555-9.
2. K. Zhao and T. G. Mason, Proc. Natl. Acad. Sci. U. S. A., 2015, 112, 12063 . ISSN 0027-8424, 1091-6490. https://pnas.org/doi/full/10.1073/pnas.1507897112.
3. S. C. Glotzer and A. Coniglio, Comput. Mater. Sci., 1995, 4, 325 . ISSN 09270256. https://linkinghub.elsevier.com/retrieve/pii/0927025695000425.
4. A. P. Ramirez, Annu. Rev. Mater. Sci., 1994, 24, 453.
5. P. N. Pusey and W. Van Megen, Nature, 1986, 320, 340 . ISSN 0028-0836, 1476-4687, https://www.nature.com/articles/320340a0.
6. A. K. Geim and I. V. Grigorieva, Nature, 2013, 499, 419 . ISSN 0028-0836, 1476-4687, https://www.nature.com/articles/nature12385.
7. A. K. Katiyar, A. T. Hoang, D. Xu, J. Hong, B. J. Kim, S. Ji and J.-H. Ahn, Chem. Rev., 2024, 124, 318 . ISSN 0009-2665, 1520-6890, https://pubs.acs.org/doi/10.1021/acs.chemrev.3c00302.
8. J. Ning, M. Kothakonda, J. W. Furness, A. D. Kaplan, S. Ehlert, J. G. Brandenburg, J. P. Perdew and J. Sun, Phys. Rev. B: Condens. Matter Mater. Phys., 2022, 106, 075422 . ISSN 2469-9950, 2469-9969, https://link.aps.org/doi/10.1103/PhysRevB.106.075422.
9. J. W. Furness, A. D. Kaplan, J. Ning, J. P. Perdew and J. Sun, J. Phys. Chem. Lett., 2020, 11, 8208 . ISSN 1948-7185, 1948-7185, https://pubs.acs.org/doi/10.1021/acs.jpclett.0c02405.
10. A. Jain, S. P. Ong, G. Hautier, W. Chen, W. D. Richards, S. Dacek, S. Cholia, D. Gunter, D. Skinner and G. Ceder, et al. , APL Mater., 2013, 1, 011002 . ISSN 2166-532X, https://aip.scitation.org/doi/10.1063/1.4812323.
11. M. Kothakonda, A. D. Kaplan, E. B. Isaacs, C. J. Bartel, J. W. Furness, J. Ning, C. Wolverton, J. P. Perdew and J. Sun, ACS Mater. Au, 2023, 3, 102 . ISSN 2694-2461, 2694-2461, https://pubs.acs.org/doi/10.1021/acsmaterialsau.2c00059.
12. A. Togo, L. Chaput, T. Tadano and I. Tanaka, J. Phys.: Condens. Matter, 2023, 35, 353001.
13. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865 . ISSN 0031-9007, 1079-7114, https://link.aps.org/doi/10.1103/PhysRevLett.77.3865.
14. S. Nosé, Prog. Theor. Phys. Suppl., 1991, 103, 1 . ISSN 0375-9687, https://academic.oup.com/ptps/article-lookup/doi/10.1143/PTPS.103.1.
15. W. G. Hoover, Phys. Rev. A, 1985, 31, 1695 . ISSN 0556-2791, https://link.aps.org/doi/10.1103/PhysRevA.31.1695.
16. E. Blundo, C. D. Giorgio, G. Pettinari, T. Yildirim, M. Felici, Y. Lu, F. Bobba and A. Polimeni, Adv. Mater. Interfaces, 2020, 7, 2000621 . ISSN 2196-7350, 2196-7350, https://onlinelibrary.wiley.com/doi/10.1002/admi.202000621.
17. H. Zhang, X.-H. Li, R.-Z. Zhang and H.-L. Cui, Mol. Catal., 2023, 547, 113330 . ISSN 24688231, https://linkinghub.elsevier.com/retrieve/pii/S2468823123004145.
18. Y. Qi, M. A. Sadi, D. Hu, M. Zheng, Z. Wu, Y. Jiang and Y. P. Chen, Adv. Mater., 2023, 35, 2205714 . ISSN 0935-9648, 1521-4095. https://onlinelibrary.wiley.com/doi/10.1002/adma.202205714.
19. Y. Han, L. Gao, J. Zhou, Y. Hou, Y. Jia, K. Cao, K. Duan and Y. Lu, ACS Appl. Mater. Interfaces, 2022, 14, 8655 . ISSN 1944-8244, 1944-8252, https://pubs.acs.org/doi/10.1021/acsami.1c23431.
20. H. Guo, Z. Hu, Z. Liu and J. Tian, Adv. Funct. Mater., 2021, 31, 2007810 . ISSN 1616-301X, 1616-3028, https://onlinelibrary.wiley.com/doi/10.1002/adfm.202007810.
21. M. Zhao, N. Trainor, C. E. Ren, M. Torelli, B. Anasori and Y. Gogotsi, Adv. Mater. Technol., 2019, 4, 1800639 . ISSN 2365-709X, 2365-709X, https://onlinelibrary.wiley.com/doi/10.1002/admt.201800639.
22. P. Xiong, R. Ma, N. Sakai and T. Sasaki, ACS Nano, 2018, 12, 1768 . ISSN 1936-0851, 1936-086X, https://pubs.acs.org/doi/10.1021/acsnano.7b08522.
23. P. Xiong, R. Ma, N. Sakai, L. Nurdiwijayanto and T. Sasaki, ACS Energy Lett., 2018, 3, 997 . ISSN 2380-8195, 2380-8195, https://pubs.acs.org/doi/10.1021/acsenergylett.8b00110.
24. M. Yang, Y. Hou and N. A. Kotov, Nano Today, 2012, 7, 430 . ISSN 17480132, https://linkinghub.elsevier.com/retrieve/pii/S1748013212000990.
25. K. Kang, K.-H. Lee, Y. Han, H. Gao, S. Xie, D. A. Muller and J. Park, Nature, 2017, 550, 229 . ISSN 0028-0836, 1476-4687, https://www.nature.com/articles/nature23905.
26. F. Wu, J. Li, Y. Su, J. Wang, W. Yang, N. Li, L. Chen, S. Chen, R. Chen and L. Bao, Nano Lett., 2016, 16, 5488 . ISSN 1530-6984, 1530-6992, https://pubs.acs.org/doi/10.1021/acs.nanolett.6b01981.
27. J. Lipton, G.-M. Weng, J. A. Rohr, H. Wang and A. D. Taylor, Matter, 2020, 2, 1148 . ISSN 25902385, https://linkinghub.elsevier.com/retrieve/pii/S2590238520301259.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d4nr03416c

---