Structure and dynamics of titania – poly(N-vinyl caprolactam) composite hydrogels

Abstract

The morphologies and dynamics of poly(N-vinyl caprolactam) (PVCL) based hydrogels with titania nanoparticles in different states (native, air-dried to a constant weight and swollen in H₂O or D₂O) are studied by a combination of complementary techniques: wide angle X-ray scattering, small angle neutron scattering, neutron spin echo spectroscopy, differential scanning calorimetry. The results suggest the presence of different structural types of water leading to different properties of the hydrogels. We propose a hierarchical structure of hydrogels spanning from the molecular to the microscopic scale consistent with both the static structure (polymer mesh size, association of the nodes of crosslinks and microchains of PVCL) and the dynamics (rate of relaxation of polymer chains, hydrodynamic polymer-polymer correlation length). The presence of nanoscale titania does not change the molecular structure and nanostructure due to its aggregation into meso-domains, but does affect the microstructure, changing the response rate to a temperature jump from 20 to 50 °C. Titania nanoparticles do not change the equilibrium swelling degree of hydrogels.

---

Introduction

Hydrogels are water-based polymeric networks physically (molecular weaves, hydrophobic interactions, ionic or hydrogen bonds) or chemically (covalent bonds) crosslinked. Smart hydrogels, that respond to stimuli such as pH, temperature, electric field, etc. are the most interesting. Poly(N-vinyl caprolactam) (PVCL) is a temperature sensitive water-soluble polymer with a lower critical solution temperature (32–34 °C) near that of the human body,¹ which has better biocompatibility compared to the well-studied thermo-sensitive poly(N-isopropyl acrylamide) (pNIPAM) polymer, as it does not decompose into a large number of low molecular weight amines during hydrolysis.²

The design of stimuli-responsive hydrogels with specific properties can be achieved chemically, via the synthesis of new polymers, or much simpler by forming hybrid composites, enhancing existing hydrogels via additives. Composite PVCL-based hydrogels with different functional groups are used for medical purposes:^3,4 antibacterial microgels (PVCL/Ag),⁵ antitumor drug delivery by (PVCL/N-(2-hydroxypropyl)methacrylamide nanogels loaded with doxorubicin),⁶ cartilage tissue engineering (PVCL hydrogel loaded with stem cells),⁷ immobilization of enzymes and cells (PVCL/calcium alginate hydrogels).⁸ Solid inorganic nanoparticles are a typical example of such additive, and in particular titania nanoparticles (TiO₂, named NT in the following) have been often studied for their photocatalytic^9,10 and antimicrobial activities.¹¹ Thus, we are interested in studying how the temperature-sensitive PVCL will be affected by the presence of titania.

Such properties of hydrogels as elasticity, transparency, and response to external influences are determined by their composition, morphology and dynamics. But there are only few works on the study of the elements of the static structure of PVCL-based hydrogels by microscopy, differential scanning calorimetry, small-angle X-ray and neutron scattering, IR spectroscopy, two-dimensional correlation spectroscopy, dynamic light scattering.^12–18 In addition, among the literature sources, there is no information about the dynamics of PVCL hydrogels and their response to temperature effects, which is the subject of this work. There are some works on other thermosensitive polymer pNIPAM based hydrogels with titania nanoparticles.^19,20 But, dynamics of polymer matrix was not studied. The aim is to prepare physical hydrogels by mixing titania nanoparticles to poly(N-vinyl caprolactam) and tetraethoxysilane as nominally pure and identify their structure and dynamics. The prepared pure gels and their titania nanoparticles containing derivates (NT/PVCL) are investigated by X-ray diffraction, differential scanning calorimetry, scanning electron microscopy, small-angle neutron scattering, and neutron spin-echo spectroscopy.

Materials and methods

Hydrogel synthesis

PVCL-based hydrogels were synthesized by sol–gel technology using 10 wt% PVCL ((C₈H₁₃NO)_n with molecular weight M_w = 1 × 10⁶ Da,²¹ overlap concentration – where η is the intrinsic viscosity²² of 10 wt% PVCL aqueous solution at 25 °C) tetraethoxysilane (TEOS, SiC₈H₂₀O₄, reagent grade, Sigma Aldrich, USA), dimethylaminoethanol catalyst (DMAE, C₄H₁₁NO, ≥99.5%, Sigma Aldrich, USA) and macadamia oil Glycereth-8 Esters (Glycereth-8, ResPharma, Italy) with the weight ration of TEOS : Glycereth-8 : DMAE = 140 : 2 : 1. The mixture was stirred at room temperature (22 ± 1 °C) for 30 minutes and poured into a mold. For the preparation of composite hydrogels TiO₂ (commercial sample Hombifine N, Sachtleben Chemie GmbH, in anatase form) was added in amounts of 0.25, 0.50 and 1.00 wt% before the introduction of TEOS (Table 1). These hybrid samples are respectively referred to as 0.25, 0.50 or 1.0NT/PVCL. The TiO₂ nanoparticles have been previously characterized by scanning electron microscopy, which revealed that the primary particle size is about 100–150 Å (86%), while approximately 14% of the particles are aggregated in larger structure with ∼200–800 Å or larger diameter.²²

Table 1 The composition of the hydrogels

Name	NT content (wt%)	PVCL content (wt%)	TEOS (wt%)	DMAE (wt%)	Macadamia oil Glycereth-8 esters (wt%)
PVCL	—	92.85	7.00	0.05	0.1
0.25NT/PVCL	0.25	92.60
0.50NT/PVCL	0.50	92.35
1.0NT/PVCL	1.00	91.85

---

The synthesis conditions of hydrogels PVCL and NT/PVCL are the same (there is a slight change in TEOS/PVCL ration (<1%), see Table 1 and Fig. S1, ESI†). Pure PVCL hydrogel is transparent, in contrast to the composite hydrogels NT/PVCL, which have a white opaque appearance due to multiple light scattering by titania nanoparticles.

There are several studies on the synthesis of PVCL-based hydrogels using the sol–gel method,^16,18 where tetramethoxysilane (TMOS, Si(CH₃OH)₄) is used as a crosslinking agent. Hydrolysis of TMOS followed by condensation of hydrolysis products occurs under mild conditions in the absence of a catalyst. As a result of such reactions, methanol is formed as a byproduct, which is unacceptable for the use of such hydrogels in medicine and cosmetology. In this regard, the synthesis of organo-inorganic hydrogels using tetraethoxysilane (TEOS, Si(C₂H₅OH)₄) is preferable, because the byproduct is ethanol, which gives the hydrogel antiseptic and protective properties.

Acid hydrolysis of TEOS is used to obtain stable sols, and inorganic acids (HCl, HNO₃, etc.) are used as acid catalysts. There are two different ways to perform sol–gel synthesis: one-stage (acid) or two-stage (acid/acid) hydrolysis of TEOS.²³ In both cases, the first stage is often carried out with a lack of water. TEOS is a crosslinking agent for the formation of hydrogels, hydrolyzed in the presence of an acid catalyst. Silanol reactive groups (Si–OH) of hydrolyzed alkoxide silicon are subjected to reactions of condensation, which depend on the pH and temperature of the solution, with the formation of siloxane (Si–O–Si) linkages, forming a 3D porous structure of the gel.

Wide angle X-ray scattering

X-ray measurements were carried out on PVCL and NT/PVCL air-dried gel disks of 1 mm thickness and taken in a rotation mode on an HZG-4 diffractometer (graphite monochromator) using CuKα radiation in 0.02 Å steps with counting times of 10 s per step over the angle range 2θ = 2–50°. We used the “Program for processing diffractograms with the possibility of adjusting the original data”.²⁴ The average crystallite sizes of NT were calculated by the formula

D = Kλ/β cos θ, (1)

where λ(CuK_α) = 1.54051 Å, 2θ ∼ 25°, β is the integral peak width, and the empirical coefficient K = 0.9. The standard deviation was 5%.

Differential scanning calorimetry

Differential scanning calorimetry (DSC) of native (as-prepared) PVCL and 0.50NT/PVCL hydrogels was performed on Netzsch DSC 204 (Phoenix, Germany). A sample with a weight of 22.5 mg for PVCL or 13 mg for 0.50NT/PVCL was placed in a standard aluminum sample pan with a vented cover. The scanning was performed at a rate of 10° min⁻¹ under an argon atmosphere (99.99%) at a flow rate of 100 mL min⁻¹ in the temperature range from −80 to 600 °C. The results (peak area, extreme temperatures) were processed with the Netzsch Proteus software. The temperature was determined with an accuracy of 0.1 °C.

Small angle neutron scattering (SANS)

SANS was applied to investigate structure of the gels on the nanometric lengthscale. SANS data²⁵ were acquired on the small angle diffractometer D11 at the Institut Laue-Langevin, Grenoble, France (DOI: http://10.5291/ILL-DATA.9-12-510). For these experiments, air-dried gel disks were swollen in D₂O in order to enhance the scattering contrast between the polymer and the matrix. The scattering length densities (SLD) of the main components of the systems are listed in Table 2.

Table 2 Neutron scattering length densities (SLD) of the components

Component	SLD, Å^2
PVCL (C8H13NO)n	0.939 × 10^−6
SiO2	4.18 × 10^−6
SiOOH	3.14 × 10^−6
NT (TiO2)	2.628 × 10^−6
D2O	6.335 × 10^−6

---

Samples of ∼1.5 mm nominal thickness and 10 mm diameters were placed in sandwich cells of 1.5 mm pathway. Three configurations were used with sample-to-detector distances of 1.4, 8 and 39 m, with collimations at 5.5, 8 and 40.5 m respectively, at a constant wavelength λ = 5.0 Å (fwhm 9%) leading to a q-range of 0.0016–0.53 Å⁻¹, where q is the magnitude of the wavevector

where θ is the scattering angle. Data were reduced with the program Lamp,²⁶ using a flat field, taking into account transmissions and thicknesses, and subtracting the scattering from a D₂O-filled cell as a background. Data were acquired in 256 × 256 pixels mode. The absolute scale was obtained from the attenuated transmitted beam. The data modeling was done with the SASfit²⁷ and the SasView programs (http://www.sasview.org/).

Neutron spin-echo (NSE) measurements

The microscopic dynamics of the PVCL matrix in the pure PVCL and NT/PVCL composite hydrogels were explored by NSE spectroscopy. NSE measurements were done on the IN11 spectrometer at the Institut Laue-Langevin (Grenoble, France) in the IN11A high-resolution set-up. For these experiments, gel films of 4 mm nominal thickness and 3 × 4 cm cross-section were used. Prior to the measurements, the gel samples were air-dried and then reswollen in excess D₂O for at least 3 days at 22.0 ± 0.1 °C. The swollen samples were placed in 4 mm thick sandwich-type cells with quartz windows. The remaining space in the sample holder was filled with D₂O to exclude air and avoid deswelling. Measurements were performed at 22.0 ± 0.1 °C, in the transfer vector q-range 0.042 Å⁻¹ ≤ q ≤ 0.219 Å⁻¹. The incident neutron wavelength was 8 Å. The resolution functions of the instrument were determined for the different experimental conditions using the elastic scattering of graphite. The NSE method measures directly the intermediate scattering function I(q,τ) as a function of q and the Fourier time τ, i.e., it yields directly the time dependence of the density–density autocorrelation function.²⁸ The resulting intermediate scattering functions were corrected for the D₂O background dynamics.

Equilibrium swelling degree

Equilibrium swelling degree (ESD) of the samples was measured on air-dried gel disks with a diameter of 9 mm and thicknesses of 1 and 2 mm. A known weight of the hydrogels was taken and immersed in an excess of water for fixed time intervals at 25.0 ± 0.2 °C and then the polymers were removed after a certain point in time, wiped with tissue paper to remove excess water, and weighed immediately during ∼11 000 min for 2 mm thickness and ∼6500 min for 1 mm thickness hydrogels. After reaching swelling equilibrium in distilled water at 25.0 ± 0.2 °C, the ESD was determined as , where m and m₀ are the mass of the swollen and dry samples, respectively. The measurements were performed three times leading to a typical standard-deviation of ca. 5%.

The kinetics of the temperature shrinkage 25 → 50 °C

To examine the response of hydrogels to temperature influence hydrogel disks in an equilibrium swelling state (after the ESD experiment) were plunged into a water bath at 50.0 ± 0.1 °C. The thermally induced shrinkage was tracked by regularly measuring the mass of the disks until they reached equilibrium. Three parallel samples were measured in each case. The measured data were fitted to a bi-exponential function:where m_τ – measured mass at time τ, g; m_i – mass of the initial gel fully swollen at 25 °C, g; m_∞ – mass of the deswollen gel at the end of the experiment, g; τ – observation time, min; τ₁ and τ₂ – fitted time constants, min; A₁ and A₂ – fitted pre-exponential constants.

Fig. 1 shows the sample preparation scheme of PVCL and NT/PVCL samples for the research methods used in this work.

Fig. 1 The sample preparation scheme.

Results and discussions

Swelling degree

The swelling process of air-dried PVCL and NT/PVCL hydrogels in H₂O obeys the second-order kinetics:where ESD is the equilibrium swelling degree (%), SD is the swelling degree at a given time t (%). The experimental data plotted on the t/SD − t coordinates are described by the linear functionThe latter was used to determine the swelling rate constant (K, min⁻¹) (Table 3).

Table 3 Swelling rate constants (K, min⁻¹) and swelling degrees (ESD, %) for air-dried PVCL and NT/PVCL hydrogels in H₂O

Sample	2 mm thickness		1 mm thickness
	K, min^−1	ESD, %	K, min^−1	ESD, %	Syneresis ratio, %	The duration of syneresis, h
a Syneresis was not established. b The sample has not been studied.
PVCL	2.1 × 10^−7	1135	1.98 × 10^−5	1144	—^a
0.25NT/PVCL	2.3 × 10^−7	1160	3.09 × 10^−5	1052	135	6
0.50NT/PVCL	3.7 × 10^−7	1074	7.52 × 10^−5	1088	120	6
1.0NT/PVCL	2.2 × 10^−7	1094	—^b

---

All studied hydrogels are characterized by a high swelling degree (Fig. 2). The presence of NT in NT/PVCL composite hydrogels slightly changes their swelling degree compared to pure PVCL hydrogel. Titania nanoparticles was not released from NT/PVCL composite hydrogel during the swelling process (as controlled spectrophotometrically (Akvilon SF 103, Russia) and visually (absence of NT sediment)). The analysis of water content after hydrogel swelling showed that less than 1 wt% of PVCL is washed out from the hydrogels (probably fractions of polymer with the lowest molecular weights). It indicates that almost all TEOS and PVCL are incorporated into the hydrogel structure.

Fig. 2 Swelling curves for air-dried pure PVCL and NT/PVCL composite gels with (a) 2 mm and (b) 1 mm thicknesses. The inset shows an enlarged view of the time range of 10–40 hours.

The thickness of the initial air-dried hydrogels influences their swelling rate, but not the swelling degree. For hydrogels with a thickness of 1 mm, the rate of swelling is larger than for ones with a thickness of 2 mm (Table 3). For the achievement of a full equilibrium state, hydrogels with a thickness of 2 mm need 6000 min, while hydrogels with a smaller thickness of 1 mm only needs 600 min (Fig. 2).

For composite hydrogel samples with a thickness of 1 mm, syneresis is characteristic during the swelling process (Fig. 2(b), inset). Syneresis is an instability phenomenon described as a “spontaneous contraction of a gel accompanied by the expulsion of liquid from the pores”.²⁹ The syneresis ratio was calculated using the following equation:

where m_i and m_f are the masses of the hydrogel at the beginning and the end of syneresis.

In composite hydrogels of 1 mm thickness, while the initial equilibrium swelling in water took place in about 10 h, a fluctuation in mass was observed 10 h later, lasting for 3 h (Table 1). We hypothesize that an increase in NT content in PVCL hydrogels reduces the syneresis ratio during swelling. The syneresis may depend on a form and thickness of samples. It should be noted that in the work³⁰ syneresis was not observed.

As SANS and NSE experiments were performed in D₂O, the kinetics of swelling process in D₂O of air-dried PVCL and NT/PVCL hydrogels with a thickness of 1 mm was checked (Fig. S2, ESI†). The equilibrium swelling degree of PVCL and NT/PVCL hydrogels in D₂O was found to be marginally higher than in H₂O.

The synthesized hydrogels have a higher swelling degree in water compared to samples in the literature: 750% (PVCL hydrogel synthesized by free radical cross-linking polymerization),³¹ 550% (chitosan-graft-poly(N-vinylcaprolactam) hydrogel, 25 °C, pH = 7.4).³² Makhaeva et al. Synthesized the PVCL-based hydrogels, for which swelling in water is accompanied by a 2500% increase in the initial mass.³³

The kinetics of the temperature shrinkage 25 → 50 °C

After placing the pure PVCL hydrogel in a water bath at 50 °C, it changed its color from transparent to white. It indicates the phase separation of the hydrogel. Observation of such a phenomenon for composite hydrogels was not possible as all hydrogels with NT initially have a white color; the photographs of dried/swollen samples are shown in Fig. S3 (ESI†). Phase separation happens at first at the local level, and two phases exist at the same time: polymer-rich and solvent-rich.³⁴ When observed with pNIPAM hydrogels, it was suggested that the rapid shrinking may accompany a characteristic change in morphology, such as bubble formation.³⁵ The bubble formation is evidence of inhomogeneous network structure characterized by a large population of loops and dangling chains. It allows the solvent to find many paths outgoing from the gel. Some of the fluxes may be captured by the outmost layer of the network, resulting in bubble formation.

The deswelling kinetics of polymer hydrogels depends sensitively on the number of structural inhomogeneities present in the network. The inhomogeneities can also be generated by drying these gels and allowing them to reswell.³⁶

All PVCL and NT/PVCL hydrogels have a multi-exponential dependence of loss of weight on time at temperature jump of 25 → 50 °C and reach the final state at 2000 min (Fig. 3). It should be noted that in the work³⁷ PVCL-based hydrogels with clay nanosheets as a crosslinker reach their deswelling equilibrium state after ∼12 min of rapidly increasing temperature (25 → 45 °C). The difference in data can be due to the different methods for producing hydrogels.

Fig. 3 Plot of the temperature jump 20 → 50 °C of PVCL, 0.25NT/PVCL, 0.50NT/PVCL and 1.0NTPVCL hydrogels. Lines are fits to eqn (3).

The obtained data in this work were described by eqn (3). A bi-exponential function approximate the experimental data and reveals uneven release of solvent from hydrogels.

The kinetic curve for 1.0NT/PVCL hydrogel has a more complex shape in comparison with other samples (Fig. 3 and Table 4). Table 4 also lists the goodness-of-fit χ² and R² ( where dof – degrees of freedom, O_i – data value, C_i – function value; where R – the coefficient of determination, n – the number of points in the data sample, k – is the number of independent regressors). For 1.0NT/PVCL hydrogel χ² = 8.04, which shows the limit of a 2-rate process for this particular sample.

Table 4 Fit parameters of kinetic curves during temperature jump 25 → 50 °C PVCL, 0.25NT/PVCL, 0.50NT/PVCL and 1.0NT/PVCL hydrogels

Sample	, %	A 1	τ 1, min	A 2	τ 2, min	χ ^2	R adj ^2
PVCL	11.92	15.71	4.31	69.07	339.37	1.46	0.9974
0.25NT/PVCL	11.42	16.55	12.53	61.96	249.54	2.89	0.9936
0.50NT/PVCL	25.03	51.86	14.03	51.10	263.59	2.53	0.9938
1.0NT/PVCL	23.71	30.16	22.33	29.85	778.02	8.04	0.9630

---

All these findings show that adding titania nanoparticles in PVCL hydrogels leads to a significant change in the relaxation of the system, composite hydrogels exhibiting a much faster response to the temperature.

Wide angle X-ray scattering

In swollen state, the diffraction pattern of NT/PVCL, similarly to other hydrogels (PVP,³⁸ pNIPAM³⁹) corresponds essentially to the signal from water, presenting two shallow peaks. The diffraction pattern of the fully swollen (ESD = 1160%) 0.25NT/PVCL hydrogel is shown on Fig. 4(a) (the sample underwent the following preparation: native → air-drying → swelling in water).

Fig. 4 X-ray diffraction patterns of (a) 0.25NT/PVCL composite gel (air-dried with following swelling in H₂O) and (b) air-dried PVCL and NT/PVCL gels. Reflections labeled * are anatase reflections (JCPDS No. 89-4921).

Based on the literature,^38–40 water in PVCL hydrogels can be classified in 4 categories: free water (fills the pores of all sizes and the space between the polymer chains and can be easily removed from the hydrogels under mild conditions) (I), bound water (water attached by hydrogen bonds –N–C=O⋯H–O–H with hydrophilic functional group –N–C=O of the PVCL side chain is an integral part of the hydrogel structure and can be removed at elevated temperatures) (II), intermediate water (water not connected to the hydrogel network, but physically trapped between polymer chains: cluster water) (III), semi-bound water (a type of water with intermediate bound and free water properties) (IV).

The 2nd diffraction peak of PVCL comes from correlations between side chains, similar to PVP⁴¹ For the swollen hydrogel 0.25NT/PVCL, it is strongly shifted towards large angles (Fig. 4(a); 2θ = 27.32° with d₂ = 3.153 Å) compared to air-dried samples (Fig. 4(b); 2θ ∼ 20° with d₂ ∼ 4.5 Å), which indicates the presence of water I and water II.

The high content of water I in the swollen 0.25NT/PVCL leads to a strong increase in the average distance between the backbone C–C chains (Fig. 5),^38,42i.e., significantly increases the interplanar distance d₁ beyond the diffractogram (Fig. 4(a) and 6(a)). In the hydrogels, crosslinking points are clusters of variable composition [Si_xO_y(OH)_z(H₂O)_vR_w] (R – C₂H₅) (Fig. 6(b)), formed by the products of hydrolytic polycondensation of TEOS (Fig. S1, ESI†). The diffuse peak at 2θ = 40.39° (d = 2.231 Å) for 0.25NT/PVCL (Fig. 4(a)), absent on the diffractograms of the dried samples (Fig. 4(b)), is responsible for water clusters (water III) in the PVCL – water system, which are trapped between polymer chains, similar to PVP⁴¹ (Fig. 5).

Fig. 5 Schematic connection of PVCL structure with its diffraction pattern.

Fig. 6 The proposed schematic illustrations of the internal network structure of PVCL-based hydrogels: (a) crosslinking of the PVCL chains, (b) primary and (c) secondary nanostructure, (d) microstructure.

It should be noted that no other diffraction peaks were recorded either due to the small number of phases outside the sensitivity of the WAXS technique or because of their X-ray amorphous state.

The 1st diffraction peak of PVCL is split for PVCL hydrogel (solid red line in Fig. 4(b) and Table 5) and asymmetric for 0.50NT/PVCL (dashed red line in Fig. 4(b) and Table 5), which can also be associated with the action of water. This type of water can be interpreted based on the cluster model of polymers.⁴³ The chain entanglement (physical crosslink) of the polymer network⁴⁴ are clusters with different content of bound water molecules (Fig. 5), and therefore different interplanar distances lead to the splitting of the 1st peak of PVCL and asymmetry for 0.50NT/PVCL (Fig. 4(b)). Since this bound water is structurally not the same as water II (Fig. 5), and the structural meaning of water IV is not clear, bound water in ordered regions is caused by water IV, otherwise, a new type of water should be introduced.

Table 5 X-ray data of air-dried PVCL and NT/PVCL gels

PVCL			0.25NT/PVCL			0.50NT/PVCL			Assignment of peaks in Fig. 4
2θ, °	d,^a Å	I, %	2θ, °	d,^a Å	I, %	2θ, °	d,^a Å	I, %
a d, Å = λ/2 sin θ – interplanar distance. b The peak is asymmetric, the angle shown corresponds to Imax.
9.9	8.9	70	11.68	7.8	55	8.6	10.3	50	1 (splitting)
11.32	7.8	65				12.00	7.3	70
19.88^b	4.46	100	20.94	4.24	100	21.14	4.20	90	2
			25.04	3.55	90	25.08	3.55	100
			37.52	2.395	5	37.48	2.398	5
			48.44	1.878	2	47.72	1.904	3

---

The PVCL and NT bind to each other via hydrogen bonds between amide –N–C=O group, which can bind no more than two water molecules¹ (bound water) and water molecules and OH-groups on the NT surface.

The introduction of NT has a different effect on the diffraction behavior of the 2nd peak of PVCL depending on the concentration (dashed black line in Fig. 4(b)): the peak is shifted towards large angles for air-dried 0.25NT/PVCL (green line in Fig. 4(b)), indicating a higher content of water II in this sample compared to 0.50NT/PVCL (solid black line in Fig. 4(b)).

The average crystallite size of nanosized anatase in the composite hydrogel is substantially smaller (D = 42 ± 2 Å for 0.25NT/PVCL and D = 54 ± 3 Å for 0.50NT/PVCL) than for the original Hombifine N (D = 82 ± 4 Å) (Table 5), indicating an amorphization of the nanoparticles (greater for 0.25NT/PVCL) caused by hydration of their shell.

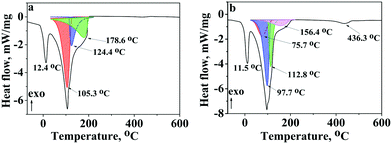
Differential scanning calorimetry (DSC)

Fig. 7 shows thermograms of native PVCL and 0.50NT/PVCL hydrogels.

Fig. 7 The thermograms of native (a) PVCL and (b) 0.50NT/PVCL hydrogels.

To describe the effects on thermograms (Fig. 7), it is necessary to refer to the properties of structural water:⁴⁵water I – free water (does not form hydrogen bonds with the polymer, NT and TEOS derivatives and behaves similarly to pure water with respect to freezing and melting), water II – non-freezing water (strongly associated with polymer chains hydrogen bonds; will not freeze but will evaporate at the highest temperature), water III – freezing water (weakly interacts with polymer chains; the reduced hydrogen bonding with the solvent implies a higher freezing temperature and a lower boiling temperature than pure water). We expect water IV,⁴⁶ as intermediate between I and III, to be indistinguishable from water I and/or III.

Based on this systematization and the data of X-ray diffraction and DSC, Table 6 presents the endothermic peaks assigned with possible water types in PVCL and 0.50NT/PVCL hydrogels.

Table 6 Assignment of the endothermic peaks in PVCL-based hydrogels

PVCL		0.50NT/PVCL		Assignment of peaks
T, °C	ΔH, J g^−1	T, °C	ΔH, J g^−1
12.4	242.0	11.5	270.5	Water III (freezing)
		75.7	386.7	The removal of physically adsorbed water from the surface of titania nanoparticles
105.3	1088.9	97.7	977.4	Water I
124.4	397.9	112.8	421.0	Water III (evaporation)
178.6	765.4	156.4	251.4	Water II
434.9		436.3		Decomposition of PVCL

---

It is possible that the endothermic peak at 75.7 °C is caused by the removal of water due to the presence of NT in the system. We do not consider water related to Si–O formations due to their small amounts.

Small angle neutron scattering

Small angle neutron scattering reveals that the structure of the hydrogels is practically not modified by the presence of the TiO₂ nanoparticles (Fig. 8). The intensity upturn at low q-values in the cases of NT/PVCL samples follows a q⁻³ trend and indicates the presence of large fractal aggregates, most probably of poorly dispersed TiO₂, larger than the window of observation (2π/q_min = 3700 Å). The region 0.015 Å⁻¹ ≤ q ≤ 0.4 Å⁻¹ has been fitted to the 1-level Beaucage model^47–49 (eqn (7)) which models the Guinier and Porod regions with a smooth transition between them and yields a radius of gyration and a Porod exponent (Table 7):where I_inc is the incoherent background intensity, G is the Guinier scaling factor, R_g is the Guinier radius, B is the Porod-prefactor and P is the Porod exponent. We refrained from using a 2-level Beaucage model, as the second level requires a second gyration radius (that impacts the first Porod term), while such a value is not accessible with our window of observation. Consequently, the low q upturn is not included in the fit. The low q trend for the pure hydrogel is q^−0.7 and is preserved upon addition of NT.

Fig. 8 SANS response curves of PVCL (red), 0.25NT/PVCL (blue) and 0.50NT/PVCL (green) hydrogels. Broken lines are fits to Beaucage unified model (eqn (7)). Inset shows the Kratky plots. Data with NT were shifted in intensity to match those of pure PVCL where the polymer scatters, i.e., at mid and high q (factors 1.12 and 0.79 for 0.25NT/PVCL and 0.50NT/PVCL respectively).

Table 7 Fit parameters from eqn (7) applied to the SANS curves of the hydrogels

	R g, Å	P	B, cm^−1	G, cm^−1	G/B
PVCL	70	2.20	2.3 × 10^−3	34.0	15 × 10^4
0.25NT/PVCL	70	2.20	2.0 × 10^−3	30.4	15 × 10^4
0.50NT/PVCL	74	2.23	2.7 × 10^−3	46.6	17 × 10^4

---

The fitting results confirm that the presence of TiO₂ hardly affects the structure of the gels (Fig. 6(c)): the value of R_g is unchanged at low NT concentration, and increases only marginally at higher NT content. The Porod exponents (P) do not differ significantly. This non-effect of the NT is likely due to the fact that NT is present in the form of very large aggregates. The position of the maxima at q ∼0.023 Å⁻¹ visible on the Kratky plots (Fig. 8 inset) is unaffected by the NT as well. The differences in G and B values are caused by small differences in swelling degree (variation of the concentration of PVCL), as shown by the rather constant ratio G/B.

Neutron spin echo measurements (NSE)

The NSE method measures the energy transfer of neutrons at an extremely high-energy resolution as a phase shift in the Larmor precession of the neutron spins in a magnetic field.²⁸ The I(q,t) curves obtained for the pure PVCL and NT/PVCL composite hydrogels normalized by the signal I(q,0) of a fully elastic scatterer (graphite), are shown in Fig. 9 at q = 0.042, 0.110, 0.137 and 0.192 Å⁻¹.

Fig. 9 Experimental intermediate scattering functions from NSE with the corresponding single exponential fits for (a) the pure PVCL hydrogel and (b) 0.50NT/PVCL composite hydrogel, measured at 22 °C.

The curves I(q,τ)/I(q,0) can be fitted by an exponential function

where Γ = 1/τ₀ is defined as the relaxation rate, with τ₀ being the relaxation time, or decaying time of the normalized intermediate scattering function.⁵⁰

It is obvious that at low q-values (q = 0.042–0.137 Å⁻¹) the measurable Fourier time is too small to obtain the complete decay of I(q,τ)/I(q,0). But at q-value 0.192 Å⁻¹, the scattering functions decay to zero (Fig. 9). For the investigated gels, pure PVCL and composite 0.50NT/PVCL, the intermediate scattering functions appear to decay to zero at infinite time, indicative of practically or pseudo-ergodic behavior, i.e., there is no frozen-in component.^50,51

The measured relaxation rates (Γ = 1/τ) are proportional to q² (Fig. 10), characteristic of the diffusive motion of polymer chains. The diffusion coefficient (D_diff) is obtained directly from the linear fit.

Γ = D_diffq² (9)

Fig. 10 Relaxation rates (Γ) vs. q² for the PVCL and 0.50NT/PVCL hydrogels. Solid lines are linear fits.

The hydrodynamic correlation length (ξ_H) is determined from the Stokes–Einstein relation

where k_B is the Boltzmann constant, T is the absolute temperature (K), and η is the viscosity of the medium (η_D2O,22°C = 1.175 × 10⁻³ N s m⁻²; interpolation of values given in ref. 52). The obtained Diffusion constants are (3.7 ± 0.1) × 10⁻¹¹ m² s⁻¹ and (3.3 ± 0.1) × 10⁻¹¹ m² s⁻¹ for PVCL and 0.50NT/PVCL, respectively, and the derived hydrodynamic correlation lengths (ξ_H) are 50 ± 0.1 Å for PVCL and 56 ± 0.1 Å for 0.50NT/PVCL. The results suggest a very small difference between the samples, however more samples should be measured to conclude definitely on the significance of this difference.

According to SEM data for the 0.25NT/PVCL hydrogel,³⁷ NT nanoparticles are covered and settled in pores of the PVCL hydrogel and form aggregates of 200–600 Å sizes, reducing the PVCL pore sizes from ∼14 000 Å for PVCL to ∼3000 Å for 0.25NT/PVCL (Fig. 6(d) and Fig. S5, ESI†).

Thus, titania nanoparticles does not affect the atomic structure (Fig. 6(a)) and nanostructure (Fig. 6(b) and (c)) of hydrogels.

Conclusions

Poly(N-vinyl caprolactam) (PVCL) based hydrogels with titania nanoparticles were synthesized by admixing TiO₂ nanoparticles with PVCL and tetraethoxysilane (TEOS), then characterized by wide angle X-ray scattering, differential scanning calorimetry, small angle neutron scattering, and neutron spin echo spectroscopy. The hydrogels structure consists of hydrophobic PVCL backbone chains with side chains with hydrophilic groups –N–C=O binding no more than two water molecules.¹ Titania nanoparticles and PVCL are bonded via hydrogen bonding between –N–C=O group and water molecules and OH-groups on the surface of NT.

The primary nanostructure is a spatial molecular PVCL network of PVCL crosslinking points consisting of clusters of variable composition [Si_xO_y(OH)_z(H₂O)_vR_w] (R – C₂H₅), formed by the products of hydrolytic polycondensation of TEOS. Free and semi-bound types of water are located in a hydrogel 3D-network. The presence of cluster (freezing) water is likely due to hydrophobic interactions.

However, neutron scattering measurements revealed that the structure of the PVCL hydrogels is not affected by the presence of NTs in the investigated lengthscale, possibly due to the fact that NTs form very large aggregates. The microscopic dynamics of the gel is marginally affected, as revealed by neutron spin-echo spectroscopy.

Titania nanoparticles do not change the equilibrium swelling degree of PVCL-based hydrogels but affect their response rate to the temperature jump 20 → 50 °C. Moreover, the NT concentration changes the form of the response curve.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This study was financially supported by the Russian Foundation for Basic Research (Project No. 18-03-00330). We also acknowledge ILL for the beamtime allocation and the staff of IN11 and D11 for the support on data analysis. This work benefited from the use of the SasView application, originally developed under NSF award DMR-0520547. SasView contains code developed with funding from the European Union's Horizon 2020 Research and Innovation Programme under the SINE2020 project, grant agreement no. 654000.

References

1. Yu. E. Kirsh, Water soluble poly-N-vinylamides. Synthesis and physicochemical properties, John Wiley and Sons, Chichester, 1998.
2. L. K. Kostanski, R. Huang, R. Ghosh and C. D. M. Filipe, J. Biomater. Sci., Polym. Ed., 2008, 19, 275.
3. A. Imaz and J. Forcada, J. Polym. Sci., Part A: Polym. Chem., 2010, 48, 1173.
4. A. K. Gaharwar, N. A. Peppas and A. Khademhosseini, Biotechnol. Bioeng., 2014, 111, 441.
5. N. Hantzschel, R. D. Hund, H. Hund, M. Schrinner, C. Luck and A. Pich, Macromol. Biosci., 2009, 9, 444.
6. Y. Tian, Y. Wang, S. Shen, X. Jiang, Y. Wang and W. Yang, Part. Part. Syst. Charact., 2015, 32, 1092.
7. R. L. Sala, M. Y. Kwon, M. Kim, S. E. Gullbrand, E. A. Henning, R. L. Mauk, E. R. Camargo and J. A. Burdick, Tissue Eng., 2017, 23, 935.
8. S. V. Kuptsova, T. Y. Mareeva, A. A. Vikhrov, T. N. Dugina, S. M. Strukova, Y. N. Belokon, K. A. Kochetkov, E. N. Baranova, V. P. Zubov, D. Poncelet, V. S. Parmar, R. Kumar and L. D. Rumsh, Appl. Biochem. Biotechnol., 2000, 88, 145.
9. Sh. M. Gupta and M. Tripathi, Chin. Sci. Bull., 2011, 56, 1639.
10. A. Mills and S. L. Hunte, J. Photochem. Photobiol., A, 1997, 108, 1.
11. H. Mahipati, Ya. Jung-Sik Kim and Sh. H. Pawar, Korean J. Chem. Eng., 2016, 33, 1989.
12. G. J. Schneider, A. Pich, F. Schneider, Y. B. Melnichenko, A. Balaceanu, J. Allgaier, D. Richter and Z. Di, Soft Matter, 2017, 13, 2738.
13. G. Su, T. Zhou, X. Liu and Y. Zhang, Phys. Chem. Chem. Phys., 2017, 19, 27221.
14. B. Xue, V. Kozlovskaya and E. Kharlampieva, J. Mater. Chem. B, 2017, 5, 9.
15. E. Gau, F. Flecken, A. N. Ksiazkiewicz and A. Pich, Green Chem., 2018, 20, 43.
16. I. V. Bakeeva, L. A. Ozerina, A. N. Ozerin and V. P. Zubov, Polym. Sci., Ser. A, 2010, 52, 496.
17. K. M. Rao, K. S. V. K. Rao and C. Ha, Gels, 2016, 2, 19.
18. W. Loos, S. Verbrugghe, E. J. Goethals, F. E. D. Prez, I. V. Bakeeva and V. P. Zubov, Macromol. Chem. Phys., 2003, 204, 98.
19. G. Huerta-Angeles, K. Hishchak, A. Strachota, B. Strachota, M. Slouf and L. Matejka, Eur. Polym. J., 2014, 59, 341.
20. L. Zhang, S. Zhang, B. He, Zh. Wu and Zh. Zhang, Z. Naturforsch., B: J. Chem. Sci., 2008, 63, 973.
21. Yu. E. Kirsh, T. M. Karaputadze and V. I. Shumskii, USSR Inventor's Certificate no. 1613446 A1, Byull. Izobret., 1990, no. 46.
22. O. Timaeva, I. Chihacheva, G. Kuzmicheva, N. Ivanovskaya and A. Dorohov, J. Mater. Res., 2018, 33, 1475.
23. P. Kakkar and B. Madhan, Mater. Sci. Eng., 2016, 66, 178.
24. Russia ‘Program for processing of diffraction patterns of nanosized and amorphous substances and calculation of the substructure’, 2017610699, 2017.
25. https://dx.doi.org/10.5291/ILL-DATA.9-12-510 .
26. D. Richard, M. Ferrand and G. J. Kearley, J. Neutron Res., 1996, 4, 33.
27. I. Brebler, J. Kohlbrecher and A. F. Thunemann, J. Appl. Crystallogr., 2015, 48, 1587.
28. Neutron Spin Echo Spectroscopy – Basics, Trends and Applications, ed. F. Mezei, C. Pappas and T. Gutberlet, Springer, Heidelberg, 2003.
29. G. W. Scherer, J. Non-Cryst. Solids, 1989, 108, 18.
30. O. I. Timaeva, I. I. Pashkin, G. M. Kuz’micheva and N. V. Sadovskaya, Mendeleev Commun., 2019, 29, 647.
31. S. Cavus, E. Cakal and L. M. Sevgili, Chem. Pap., 2015, 69, 1367.
32. M. Prabaharan, J. J. Grailer, D. A. Steeber and S. Gong, Macromol. Biosci., 2008, 8, 843.
33. E. M. Makhaeva, L. T. M. Thanh, S. G. Starodoubtsev and A. R. Khokhlov, Macromol. Chem. Phys., 1996, 197, 1973.
34. T. Takigawa, T. Yamawaki, K. Takahashi and T. Masuda, Polym. J., 1999, 31, 595.
35. M. Shibayama and K. Nagai, Macromolecules, 1999, 32, 7461.
36. K. Laszlo, A. Fluerasu, A. Moussaid and E. Geissler, Soft Mater., 2010, 6, 4335.
37. K. Shi, Z. Liu, C. Yang, X. Y. Li, Y. M. Sun, Y. Deng, W. Wang, X. J. Ju, R. Xie and L. Y. Chu, ACS Appl. Mater. Interfaces, 2017, 9, 21979.
38. J. Teng, S. Bates, D. Engers, K. Leach, P. Schields and Y. Jang, J. Pharm. Sci., 2010, 99, 3815.
39. R. Naohara, K. Narita and T. Ikeda-Fukazawa, Chem. Phys. Lett., 2017, 670, 84.
40. Introduction to Hydrogels. In Biomedical Applications of Hydrogels Handbook, ed. R. M. Ottenbrite, Springer, London, 2010.
41. T. Matsunaga, T. Sakai, Y. Akagi, U. Chung and M. Shibayama, Macromolecules, 2014, 47, 763.
42. R. Busselez, A. Arbe, S. Cerveny, S. Capponi, J. Colmenero and B. Frick, J. Chem. Phys., 2012, 137, 084902.
43. M. Arzhakov, Relaxation in Physical and Mechanical Behavior of Polymers, CRC Press, Taylor & Francis Group Boca Raton, London, New York, 2019.
44. J. M. Uruena, A. A. Pitenis, R. M. Nixon, K. D. Schulze, T. E. Angelini and W. G. Sawyer, Biotribology, 2015, 1-2, 24.
45. B. Cursaru, P. O. Stanescu and M. Teodorescu, Sci. Bull. - Univ. “Politeh.” Bucharest, Ser. B, 2010, 72, 99.
46. B. Hammouda, D. L. Ho and S. Kline, Macromolecules, 2004, 37, 6932.
47. G. Beaucage and D. Schaefer, J. Non-Cryst. Solids, 1994, 797, 172.
48. G. Beaucage, J. Appl. Crystallogr., 1995, 28, 717.
49. G. Beaucage, J. Appl. Crystallogr., 1996, 29, 134.
50. B. Berke, O. Czakkel, L. Porcar, E. Geissler and K. Laszlo, Soft Matter, 2016, 12, 7166.
51. T. Hellweg, K. Kratz, S. Pouget and W. Eimer, Colloids Surf., A, 2002, 202, 223.
52. Water in biology, chemistry, and physics: Experimental overviews and computational methodologies, ed. G. W. Robinson, S. B. Zhu, S. Singh and M. W. Evans, World Scientific, Singapore, 1996.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c9sm01619h

---