Open Access Article
Open Access ArticleA chiral metal cluster triggers enantiospecific electronic transport†
Omar
Hernández-Montes
 a,
Ignacio L.
Garzón
a,
Ignacio L.
Garzón
 b and
J. Eduardo
Barrios-Vargas
b and
J. Eduardo
Barrios-Vargas
 *a
*a
aDepartamento de Física y Química Teórica, Facultad de Química, Universidad Nacional Autónoma de México, Ciudad de México 04510, Mexico. E-mail: omar.hdz.mon@gmail.com; j.e.barrios@gmail.com
bInstituto de Física, Universidad Nacional Autónoma de México, Apartado Postal 20-364, Ciudad de México 01000, Mexico. E-mail: garzon@fisica.unam.mx
First published on 11th January 2024
Abstract
Chirality is a geometric property of matter that can be present at different scales, especially at the nanoscale. Here, we investigate the manifestation of chirality in electronic transport through a molecular junction. Spinless electronic transport through a chiral molecular junction is not enantiospecific. However, when a chiral metal cluster, C3-Au34, is attached to the source electrode, a different response is obtained in spinless electronic transport between R and L systems: this indicates the crucial role of chiral clusters in triggering enantiospecific spinless electronic transport. In contrast, when an achiral metal cluster, C3v-Au34, is attached, no change in conductance occurs between enantiomeric systems. Using the non-equilibrium green's function method, we characterized this phenomenon by calculating the transmission and conductance of spin-unpolarized electrons. Our theoretical results highlight the importance of metal clusters with specific sizes and chiral structures in electronic transport and support previously published experimental results that exhibited enantiospecific scanning tunneling measurements with intrinsically chiral tips.
Chirality is a geometric property of matter present at every scale in the universe. At the nanoscale, it has been studied in bare and ligand-protected metal clusters and nanoparticles.1,2 In these studies, chirality has been detected through its physical and chemical manifestations. In the first case, chiral nanostructures may interact with circularly polarized light to display a nonzero circular dichroism spectrum.2 Chirality is also chemically expressed when two chiral systems interact with each other, inducing the phenomenon of chiral molecular recognition.2,3 In this work, a third mechanism of manifestation of chirality is proposed by utilizing a chiral bare metal cluster to modify a molecular junction that triggers enantiospecific electronic transport.
Electronic transport at the nanoscale has been broadly investigated, partly motivated by the miniaturization of electronic devices and the need to disclose novel phenomena in such devices.4–6 On the one hand, the conductance in metallic short wires is quantized and depends on the length and cross section.7–9 On the other hand, novel phenomena have been discovered when a molecule is used instead of a metallic nanostructure. For example, when a current passes through chiral molecules, transport depends on the spin of the electron, which is determined by the handedness of the molecule and the direction of motion.10 This effect, called chiral-induced spin selectivity (CISS), shows the importance of molecular chirality in molecular electronics and spintronics.11
One question to investigate is whether enantiospecific electronic transport could be achieved as a result of the presence of chiral clusters at molecular junctions. This work theoretically investigates this possibility by calculating the transmission coefficient of spin-unpolarized electrons moving through a chiral-modified molecular junction. Indeed, our results show that enantiospecific transport is obtained for electrons independently of their spin when the first electrode (source) is modified by attaching a chiral metal cluster to its surface before the electron current passes through chiral molecules towards the second (drain) electrode. In this way, the wave function amplitude and phase of the departing electrons from one side of the junction are modified when they interact with the chiral electron density of the attached cluster.
This type of enantiospecific electron transport has not been widely discussed before. However, a related proposal has been made by Liu et al. in ref. 12. They achieved real-time chiral recognition using the enantiospecific interaction of the β-cyclodextrin molecular machine with different amino acids, manifesting as a different electrical response when the system is connected to graphene electrodes. Also, Tierney et al. in ref. 13 suggested the existence of intrinsic chirality in regular scanning tunneling microscope (STM) tips, which leads to different electron tunneling efficiencies through left- and right-handed molecules, with no need for a magnetized STM tip, as required in the CISS effect. More recently, a theoretical study reported a systematic study on the emergence of spin-polarization caused by electrodes in molecular junctions with reduced (chiral) symmetries.14
Chiral molecule
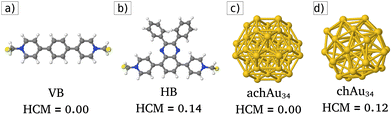
The present study focuses on electronic transport through a chiral viologen molecule (see Fig. 1a and b). Viologen molecules form a set of organic molecules that have been used as linkers of nitrogenous bases and amino acids,15 to anchor Ag nanoparticles to graphene oxide,16 also widely used as building blocks of molecular machines,17 and more recently in STM breaking junctions for molecular electronics studies.18–20 Specifically, we consider the chiral HB viologen molecule, which has recently been synthesized.21 Its structure can be decomposed into an achiral backbone (VB, viologen backbone) displayed in Fig. 1a. The chiral HB viologen is formed by the VB plus two phenyl rings attached to the VB's middle part by a quinoxaline bridge. These phenyl rings have different orientations to each other, providing chirality to the HB viologen molecule, as depicted in Fig. 1b. This chirality can be measured by calculating the Hausdorff Chirality Measure (HCM).22 The HCM quantifies the degree of overlap between enantiomers using the Hausdorff distance between their sets of atoms. Comparisons between chiral cluster structures with different HCM values have been made for bare and ligand-protected gold clusters.23 In this way, HCM allows us to verify that VB is achiral (HCM = 0.0) and to assess the degree of chirality of the chiral HB viologen (HCM = 0.14).Modified molecular junctions
These devices are composed of two crystalline gold electrodes at the ends of the molecular junction, then an achiral (or a chiral) Au34 gold cluster is attached to the left (source) electrode, and finally, at the middle part of these molecular devices, viologen-like molecules (VB and both HB enantiomers) are connected on their left side to the achiral (or chiral) cluster and their right side to the drain electrode, and also, the electrodes were modeled with a FCC lattice exposing the surface (100). For the achiral gold cluster C3ν-Au34 (here labeled achAu34, see Fig. 1c), a HCM = 0.0 value is obtained. Unlike that, the chiral metal gold cluster C3-Au34 (here named chAu34, see Fig. 1d), has a non-zero index of chirality: HCM = 0.12.2,24–27 In this work, we focus on three different molecular junctions to study electronic transport systematically: (1) an unmodified pristine molecular junction (no clusters are attached), (2) an achiral-modified molecular junction (with the presence of achAu34), and finally (3) a chiral-modified molecular junction (with the presence of chAu34).‡Electronic transport through the modified molecular junctions described above was characterized by calculating the transmission function, I–V curves, and conductance using the TranSIESTA28 module integrated into the ab initio materials program based on the density functional theory called SIESTA.29,30 TranSIESTA enables the modeling of nonequilibrium electronic transport using the Green's function formalism31–33 (further details about the calculation of electronic transport quantities are shown in S1 ESI†).
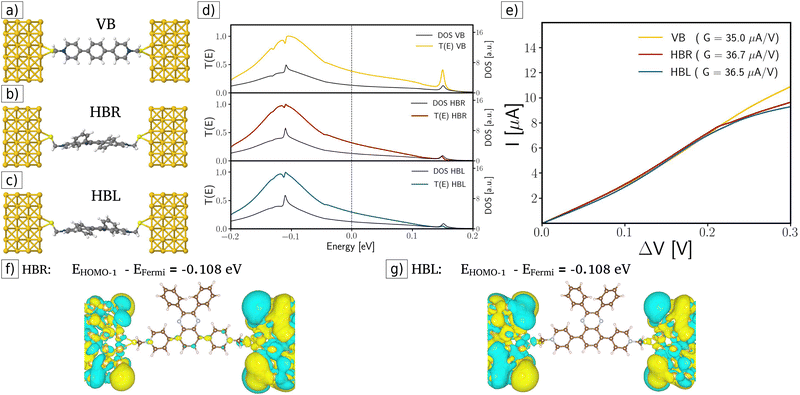
In the first case (see Fig. 2a–c), we calculate the transmission function of single molecular junctions considering the current traveling from the pristine source electrode through the VB and the R and L enantiomers of the chiral HB molecule (HBR and HBL). Fig. 2d displays the corresponding transmission functions: the yellow curve corresponds to the VB case, the red curve for HBR, and the blue curve for HBL. These functions are similar in every case (see Fig. 2d); consequently, this similarity manifests itself as similar slopes (or conductance G) in the ohmic region (linear dependence region in the range 0–0.25 V) in the I–V curves (see Fig. 2e). After linear fitting the I–V curves for the two enantiomers HBR and HBL, their corresponding conductance is G = 36.7 μA V−1 and G = 36.5 μA V−1, respectively. These values indicate that, for the pristine molecular junction case, in the ohmic region, both enantiomers have a very similar electronic transport behavior without any enantiospecific effect. In other words, enantiospecific electronic transport is not present for single-molecular junctions. This result is consistent with previous calculations of molecule carbon rings; further details of the calculation parameters are available in S2 ESI.†
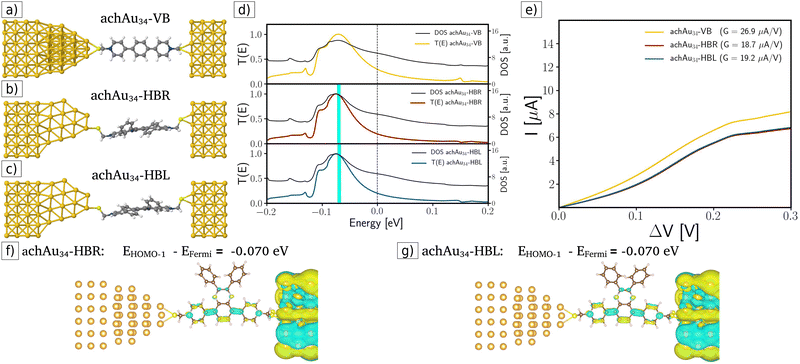
In the second case, an achiral achAu34 gold cluster is placed on the surface of the source electrode, considering an Au–Au distance equal to 2.5 Å, without performing any geometric optimization. Furthermore, the VB, HBR, and HBL molecules are also connected to the achAu34 cluster with an Au–S distance (2.60 Å) in the bridge configuration, which has been previously obtained in other calculations of Au clusters protected by thiol.3,27,34 These achiral-modified molecular junctions are displayed in Fig. 3a–c. Also, Fig. 3d displays their respective transmission functions, indicating a behavior similar to that obtained for the pristine source electrode. Therefore, in this achiral-modified electrode configuration, no enantiospecific electronic transport was achieved; however, lower conductance values were obtained compared to the pristine case (see the corresponding slopes in the I–V curves in Fig. 3e).
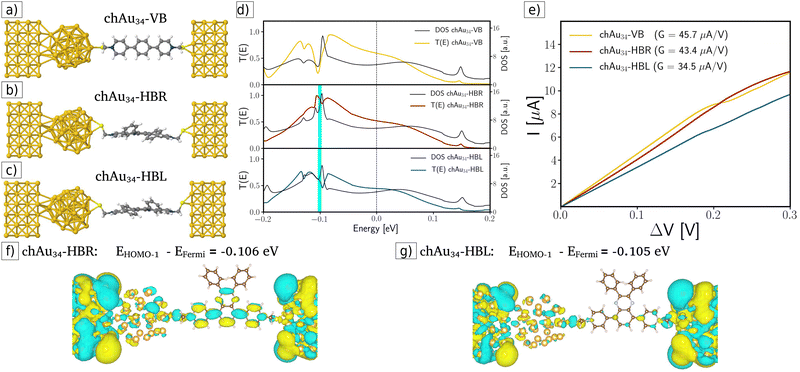
In the third case, chiral-modified molecular junctions were built by attaching the chiral metal cluster chAu34 to the surface of the left-handed electrode (see Fig. 4a–c). By analyzing the transmission coefficients, DOS, and I–V curves displayed in Fig. 4d and e, clear evidence for enantiospecific electronic transport was obtained. For example, in the ohmic region (see Fig. 4e) the transport is favored for the chAu34-HBR system, which increases the conductance by 20% with respect to the chAu34-HBL case: GchAu34-HBR (43.4 μA V−1) > GchAu34-HBL (34.5 μA V−1). The origin of this enantiospecific electronic transport is related to a significant difference in transmission functions and DOS at energies around −0.1 eV. For example, the transmission drop-off in the chAu34-HBL case and the maximum transmission for the chAu34-HBR enantiomer occurs at energies around −0.1 eV, between the energy values of the HOMO and HOMO−1 eigenstates. Fig. 4d shows a plot of the transmission function for the chiral-modified case, in which it is indicated with a cyan bar the position of the HOMO−1 eigenstate. Fig. 4f and g show the amplitude of the HOMO−1 molecular orbitals for each case. These figures show that the HOMO−1 of the chAu34-HBL enantiomer is more localized than the HOMO−1 for the chAu34-HBR one. Then, the drop in the transmission function for the chAu34-HBL case, around −0.1 eV, is due to a higher localization of its molecular orbital HOMO−1 mainly along the backbone region (see Fig. 4f). Moreover, Fig. 4g shows that the amplitude of the HOMO−1 orbital is highly delocalized for the chAu34-HBR enantiomer, which is consistent with a maximum of the transmission function, in contrast with the chAu34-HBL case in which transmission decreases. It should be noted that these results are a consequence of the chAu34 presence in the chiral-modified case since the delocalized orbital was not obtained in the achiral-modified case (see Fig. 3f and g). Therefore, the molecule's handedness induced a higher delocalization in the HBR enantiomer than in the HBL one.
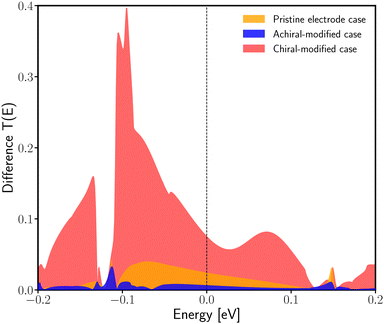
Another quantitative way to prove the enantiospecific effect in electronic transport is through the difference in the transmission as an energy function between the R- and L-enantiomeric systems. Fig. 5 shows this difference for the pristine case (orange curve), the achiral-modified case (blue curve), and the chiral-modified one (red curve). From the graph previously mentioned, it is possible to compare the three setups here analyzed and see that, definitively, the chAu34 metal cluster brings out a significant difference in the probability of transmission of electrons in chiral systems. Additionally, the degree of localization/delocalization of the HOMO−1 orbitals provides a simple explanation for the origin of enantiospecific electronic transport in the chiral-modified molecular junction.
To provide additional insights into the mechanisms responsible for the enantiospecific electron transport described above, the orbital occupancy of the carbon and sulfur atoms in the achiral- and chiral-modified molecular junctions was investigated by calculating the difference in the projected density of states (PDOS) between the HBR and HBL cases (see S3 ESI† section for a description and figures of the PDOS). From these calculations, it was found that, for the achiral case, the p-like orbitals of the carbon and sulfur atoms do not show a significant difference between the HBL and HBR cases (see Fig. S3c and d, respectively, ESI†). On the contrary, in the case of the chiral-modified molecular junctions, there is a significant difference in PDOS between the HBR and HBL cases, particularly for the C 2py and S 3py orbitals (see Fig. S4c and d, respectively, ESI†). Therefore, these results indicate that the p-like orbitals of the carbon and sulfur atoms have a similar orbital occupancy when an achiral cluster is present, but the opposite behavior (different orbital occupancy) is obtained when a chiral cluster modifies the gold electrode. These results provide further insights into the relationship of the enantiospecific electron transport with the different orbital angular momentum of the transmitted electrons. This orbital-related mechanism is consistent with recent results claiming that the orbital occupancy (polarization), caused by a lowering of the symmetry or a distortion of the atomic structure, determines chemical and physical properties such as electron transport (see, for example: Lee et al. 2021 in ref. 35 and Do and Lee 2022 in ref. 36).
One remaining question concerns the relationship between the present results and those obtained using chiral STM tips.13 The chiral-modified molecular junction investigated in this study could be proposed as a theoretical model for the experimental setup discussed in ref. 13. On the basis of the above results, the chiral-modified molecular junction better describes the experimental situation. This indicates that the STM tip requires a chiral shape at the very end of the tip to obtain enantiospecific electron transmission efficiencies.
Final remarks
By attaching a chiral metal cluster Au34 to the surface of a pristine source electrode and, at the same time, connecting to a chiral HB molecule, enantiospecific electronic transport was achieved. As exposed, the localization/delocalization of the principal transmission channel (HOMO−1) was the key feature that underlies the enantiospecific transport. For this achievement, it was necessary to maintain the chiral structure of the C3-Au34 cluster attached to the source electrode.It is worth mentioning that the enantiospecific electron transport predicted in this study is independent of spin, in contrast to the CISS effect. However, in both cases, molecular chirality plays an important role, either by modifying the electron wave function passing through a chiral metal cluster or coupling the spin to the electron density of a chiral molecule.
The present study is expected to motivate further theoretical studies looking for additional features related to enantiospecific electronic transport and cluster electronics applications.37 At the same time, further experimental work that clarifies the role of chiral STM tips in enantioselective electron tunneling efficiencies would be desirable.
Author contributions
All the authors contributed to the planning of the investigation. OHM carried out the numerical calculations and wrote a draft of the main results and the manuscript, which all the authors improved.Conflicts of interest
There are no conflicts to declare.Acknowledgements
OHM acknowledges CONAHCYT for a graduate student scholarship. JEBV acknowledges funding from CONAHCYT Frontera 428214 and PAIP-FQ(UNAM) 5000-9173. ILG thanks the funding of CONAHCYT-Mexico under Project 285821, DGAPA-UNAM-Mexico under Project IN106021, and DGTIC-UNAM-Mexico under Project LANCAD 049.Notes and references
- J. M. Hicks, The Physical Chemistry of Chirality, Chirality: Physical Chemistry, American Chemical Society, 2002, pp. 2–16 Search PubMed.
- J. J. Pelayo, I. Valencia, A. P. Garcáa, L. Chang, M. López, D. Toffoli, M. Stener, A. Fortunelli and I. L. Garzón, Adv. Phys.: X, 2018, 3, 1509727 Search PubMed.
- X. López-Lozano, L. A. Pérez and I. L. Garzón, Phys. Rev. Lett., 2006, 97, 233401 CrossRef PubMed.
- A. Nitzan and M. A. Ratner, Science, 2003, 300, 1384–1389 CrossRef CAS PubMed.
- M. L. Perrin, E. Burzurí and H. S. J. van der Zant, Chem. Soc. Rev., 2015, 44, 902–919 RSC.
- F. Evers, R. Korytár, S. Tewari and J. M. van Ruitenbeek, Rev. Mod. Phys., 2020, 92, 035001 CrossRef CAS.
- J. I. Pascual, J. Méndez, J. Gómez-Herrero, A. M. Baró, N. Garcia, U. Landman, W. D. Luedtke, E. N. Bogachek and H. P. Cheng, Science, 1995, 267, 1793–1795 CrossRef CAS PubMed.
- M. Brandbyge, J. Schiøtz, M. R. Sørensen, P. Stoltze, K. W. Jacobsen, J. K. Nørskov, L. Olesen, E. Laegsgaard, I. Stensgaard and F. Besenbacher, Phys. Rev. B: Condens. Matter Mater. Phys., 1995, 52, 8499–8514 CrossRef PubMed.
- N. Agraït, A. L. Yeyati and J. M. van Ruitenbeek, Phys. Rep., 2003, 377, 81–279 CrossRef.
- R. Naaman and D. H. Waldeck, J. Phys. Chem. Lett., 2012, 3, 2178–2187 CrossRef CAS PubMed.
- R. Naaman and D. H. Waldeck, Annu. Rev. Phys. Chem., 2015, 66, 263–281 CrossRef CAS PubMed.
- Z. Liu, X. Li, H. Masai, X. Huang, S. Tsuda, J. Terao, J. Yang and X. Guo, Sci. Adv., 2021, 7, eabe4365 CrossRef CAS PubMed.
- H. L. Tierney, C. J. Murphy and E. C. H. Sykes, Phys. Rev. Lett., 2011, 106, 010801 CrossRef PubMed.
- W. Dednam, M. A. García-Blázquez, L. A. Zotti, E. B. Lombardi, C. Sabater, S. Pakdel and J. J. Palacios, ACS Nano, 2023, 17, 6452–6465 CrossRef CAS PubMed.
- L. Barravecchia, I. Neira, E. Pazos, C. Peinador and M. D. García, J. Org. Chem., 2022, 87, 760–764 CrossRef CAS PubMed.
- S. Krishnamurthy, I. V. Lightcap and P. V. Kamat, J. Photochem. Photobiol., A, 2011, 221, 214–219 CrossRef CAS.
- H. Chen and J. Fraser Stoddart, Nat. Rev. Mater., 2021, 6, 804–828 CrossRef CAS.
- A. Borges, E.-D. Fung, F. Ng, L. Venkataraman and G. C. Solomon, J. Phys. Chem. Lett., 2016, 7, 4825–4829 CrossRef CAS PubMed.
- Q. V. Nguyen, P. Martin, D. Frath, M. L. Della Rocca, F. Lafolet, S. Bellinck, P. Lafarge and J.-C. Lacroix, J. Am. Chem. Soc., 2018, 140, 10131–10134 CrossRef CAS PubMed.
- H. Chen, H. Zheng, C. Hu, K. Cai, Y. Jiao, L. Zhang, F. Jiang, I. Roy, Y. Qiu, D. Shen, Y. Feng, F. M. Alsubaie, H. Guo, W. Hong and J. F. Stoddart, Matter, 2020, 2, 378–389 CrossRef CAS.
- Z. Ding, H. Chen, Y. Han and J. Liu, J. Mol. Struct., 2022, 1262, 133073 CrossRef CAS.
- I. L. Garzón, J. A. Reyes-Nava, J. I. Rodríguez-Hernández, I. Sigal, M. R. Beltrán and K. Michaelian, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 66, 073403 CrossRef.
- J. J. Pelayo, R. L. Whetten and I. L. Garzón, J. Phys. Chem. C, 2015, 119, 28666–28678 CrossRef CAS.
- I. E. Santizo, F. Hidalgo, L. A. Pérez, C. Noguez and I. L. Garzón, J. Phys. Chem. C, 2008, 112, 17533–17539 CrossRef CAS.
- A. Lechtken, D. Schooss, J. R. Stairs, M. N. Blom, F. Furche, N. Morgner, O. Kostko, B. von Issendorff and M. M. Kappes, Angew. Chem., Int. Ed., 2007, 46, 2944–2948 CrossRef CAS PubMed.
- X. Gu, S. Bulusu, X. Li, X. C. Zeng, J. Li, X. G. Gong and L.-S. Wang, J. Phys. Chem. C, 2007, 111, 8228–8232 CrossRef CAS.
- J. J. Pelayo, I. Valencia, G. Díaz, X. Lopez-Lozano and I. L. Garzón, Eur. Phys. J. D, 2015, 69, 1–7 CrossRef CAS.
- M. Brandbyge, J.-L. Mozos, P. Ordejón, J. Taylor and K. Stokbro, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 65, 165401 CrossRef.
- J. M. Soler, E. Artacho, J. D. Gale, A. García, J. Junquera, P. Ordejón and D. Sánchez-Portal, J. Condens. Matter Phys., 2002, 14, 2745 CrossRef CAS.
- A. García, N. Papior, A. Akhtar, E. Artacho, V. Blum, E. Bosoni, P. Brandimarte, M. Brandbyge, J. I. Cerdá, F. Corsetti, R. Cuadrado, V. Dikan, J. Ferrer, J. Gale, P. García-Fernández, V. M. García-Suárez, S. García, G. Huhs, S. Illera, R. Korytár, P. Koval, I. Lebedeva, L. Lin, P. López-Tarifa, S. G. Mayo, S. Mohr, P. Ordejón, A. Postnikov, Y. Pouillon, M. Pruneda, R. Robles, D. Sánchez-Portal, J. M. Soler, R. Ullah, V. W.-Z. Yu and J. Junquera, J. Chem. Phys., 2020, 152, 204108 CrossRef PubMed.
- S. Datta, Electronic Transport in Mesoscopic Systems, Cambridge University Press, 1995 Search PubMed.
- S. Datta, Nanotechnology, 2004, 15, S433 CrossRef CAS.
- S. Datta, Quantum transport: atom to transistor, Cambridge University Press, 2005 Search PubMed.
- R. J. C. Batista, M. S. C. Mazzoni, I. L. Garzón, M. R. Beltrán and H. Chacham, Phys. Rev. Lett., 2006, 96, 116802 CrossRef PubMed.
- A. T. Lee, H. Park and S. Ismail-Beigi, Phys. Rev. B: Condens. Matter Mater. Phys., 2021, 103, 125105 CrossRef CAS.
- V.-H. Do and J.-M. Lee, ACS Nano, 2022, 16, 17847–17890 CrossRef CAS PubMed.
- T. C. Siu, J. Y. Wong, M. O. Hight and T. A. Su, Phys. Chem. Chem. Phys., 2021, 23, 9643–9659 RSC.
Footnotes |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d3cp04581a |
| ‡ The atomic coordinates of bonded viologens to chAu34 are available in: for HBR https://github.com/jebarriosvargas/PCCP_D3CP04581A/blob/2ac6373d2bffaf2a9e8e92ef24416b3a99a05376/au34_hb3R.pdb and HBL https://github.com/jebarriosvargas/PCCP_D3CP04581A/blob/2ac6373d2bffaf2a9e8e92ef24416b3a99a05376/au34_hb3L.pdb. |
| This journal is © the Owner Societies 2024 |