Kinetics of the reaction of CO₃˙⁻(H₂O)_n, n = 0, 1, 2, with nitric acid, a key reaction in tropospheric negative ion chemistry

Abstract

A significant fraction of nitrate in the troposphere is formed in the reactions of HNO₃ with the carbonate radical anion CO₃˙⁻ and the mono- and dihydrated species CO₃˙⁻(H₂O)_1,2. A reaction mechanism was proposed in earlier flow reactor studies, which is investigated here in more detail by quantum chemical calculations and experimental reactivity studies of mass selected ions under ultra-high vacuum conditions. Bare CO₃˙⁻ forms NO₃⁻(OH˙) as well as NO₃⁻, with a total rate coefficient of 1.0 × 10⁻¹⁰ cm³ s⁻¹. CO₃˙⁻(H₂O) in addition affords stabilization of the NO₃⁻(HCO₃˙) collision complex, and thermalized CO₃˙⁻(H₂O) reacts with a total rate coefficient of 6.3 × 10⁻¹⁰ cm³ s⁻¹. A second solvent molecule quenches the reaction, and only black-body radiation induced dissociation is observed for CO₃˙⁻(H₂O)₂, with an upper limit of 6.0 × 10⁻¹¹ cm³ s⁻¹ for any potential bimolecular reaction channel. The rate coefficients obtained under ultra-high vacuum conditions are smaller than in the earlier flow reactor studies, due to the absence of stabilizing collisions, which also has a strong effect on the product branching ratio. Quantum chemical calculations corroborate the mechanism proposed by Möhler and Arnold. The reaction proceeds through a proton-transferred NO₃⁻(HCO₃˙) collision complex, which can rearrange to NO₃⁻(OH˙)(CO₂). The weakly bound CO₂ easily evaporates, followed by evaporation of the more strongly attached OH˙, if sufficient energy is available.

---

Introduction

The CO₃˙⁻ radical anion is an important intermediate in the tropospheric chemistry of anions.^1,2 Quantitative modeling by Kawamoto et al.³ places the fractional abundance of CO₃˙⁻ core ions in the troposphere in the range of 0.9–2.3%, quite comparable to HSO₄⁻ core ions close to the ground, while the overwhelming majority of negative ions have an NO₃⁻ core. However, a significant fraction of NO₃⁻(H₂O) is formed in the reaction of CO₃˙⁻(H₂O) with HNO₃.³ In recent years, ions in the troposphere have received increased attention in the form of charged aerosol particles.⁴ In a series of laboratory experiments, the CLOUD collaboration at CERN has recently shown that ions play an important role for aerosol nucleation and cloud formation in the troposphere.^5–8

The formation of CO₃˙⁻ in atmospheric conditions was described by Fehsenfeld, Ferguson and co-workers in the 1970s.⁹ In the first step electrons react with O₂ and an additional collision partner M to form O₂⁻, reaction (1). Ozonide O₃˙⁻ is formed by charge transfer to an ozone molecule, reaction (2). The ozonide anion finally transfers O⁻ to a carbon dioxide molecule, forming CO₃˙⁻ and O₂, reaction (3).

e⁻ + O₂ + M → O₂˙⁻ + M, M = O₂, N₂, Ar, H₂O,… (1)

O₂˙⁻ + O₃ → O₂ + O₃˙⁻ (2)

O₃˙⁻ + CO₂ → CO₃˙⁻ + O₂ (3)

The properties of CO₃˙⁻ itself were intensively studied by spectroscopy. Matrix isolation techniques were applied by Jacox et al.,¹⁰ and a number of other groups used photodissociation techniques to characterize CO₃˙⁻.^11–14 The O₂C–O⁻ bond dissociation energy was determined by Johnson, Viggiano and co-workers via photodissociation and high level quantum chemical calculations to be 269 ± 5 kJ mol⁻¹.^15–17

Despite its key role in tropospheric anion chemistry,¹⁸ only a small number of gas phase reactions were studied.^19–23 These are mostly reactions with nitrogen oxides, leading to formation of nitrate NO₃⁻.^3,24–27 Reactions of CO₃⁻(H₂O)_0,1,2 with formic, acetic, propionic, butyric, glyoxylic, pyruvic and pinonic acid were investigated in a flow tube experiment by Arnold et al.^28,29 They reported as initial step mostly proton transfer giving the deprotonated acids as products. For propionic acid and larger, they also observed clustering with CO₃˙⁻ and formation of radical species, e.g. CH₃CH(˙)COO⁻ from propionic acid.^28,29 However, we recently showed that the reaction with formic acid proceeds mostly via oxidation of formic acid to carbon dioxide and water.³⁰ The deprotonation product HCOO⁻ was re-assigned to the reaction of formic acid with HCOO⁻(OH˙), an intermediate in the oxidation reaction that is formed in small concentrations.

A similar pattern emerged for the reaction of CO₃˙⁻ with HCl. A flow tube study by Dotan et al.²⁴ established an upper limit for the rate of 3 × 10⁻¹¹ cm³ s⁻¹, without observing any products. Recent studies in our laboratory reveal that this reaction proceeds via formation of a short lived, very reactive Cl⁻(OH˙) intermediate that reacts fast with a second HCl molecule, producing HCl₂⁻ and Cl₂˙⁻ as final products.³¹ The rate of the first step is 4.2 × 10⁻¹² cm³ s⁻¹, consistent with the upper limit given by Dotan et al.²⁴ Interestingly, hydration dramatically accelerates the rate to 2.7 × 10⁻¹⁰ cm³ s⁻¹ for CO₃˙⁻(H₂O), resulting in the reactive Cl⁻(HCO₃˙) radical species.³¹

Nitric acid^21–23,32 and methane sulfonic acid²⁰ show efficient reactions with CO₃˙⁻. The gas phase ion chemistry of HNO₃ was extensively investigated by Fehsenfeld and coworkers.²¹ They reported a reaction rate coefficient of 8 × 10^-10 cm³ s⁻¹ for reaction (4), measured by the flowing afterglow technique.

CO₃˙⁻ + HNO₃ → NO₃⁻ + [CO₂,OH˙] (4)

Möhler and Arnold investigated the reaction of HNO₃ with CO₃˙⁻ and CO₃˙⁻(H₂O) in a flow-reactor triple quadrupole mass spectrometer in the presence of 1.7 mbar N₂ and 0.3 mbar O₂ as buffer gas.²² For bare CO₃˙⁻ they observed NO₃⁻, [NO₃,OH˙]⁻ and [CO₃˙,HNO₃]⁻ as primary products, followed by clustering reactions with HNO₃.²² No [NO₃,OH˙]⁻ was observed when 0.03 mbar H₂O was added to the buffer gas to study the hydrated species, while [CO₃˙,HNO₃]⁻ was the dominant product.²² A mechanism via an excited collision complex [(CO₃˙HNO₃)⁻]* was proposed. The complex is de-exited by collisions, or decays into NO₃⁻ or [NO₃,OH˙]⁻.²² Catoire and co-workers used the CO₃˙⁻ + HNO₃ reaction for testing their flowing afterglow setup and observed the same primary products as Möhler and Arnold.^22,32 Since no mass selection was available for the reactant ions, the exact sequence of reactions generating the observed products, in particular the role of the [NO₃,OH˙]⁻ and the influence of an additional water molecule on the reactivity, remained unclear.

Because of its central role in tropospheric negative ion chemistry,^1,3 we investigated the reaction of CO₃˙⁻(H₂O)_n, n = 0, 1, 2, with HNO₃ by FT-ICR mass spectrometry and quantum chemical calculations. Each cluster size was studied separately with mass-selected ions. The extremely low pressure in the FT-ICR instrument leads to product distributions different from the flow reactor studies, due to the absence of stabilizing collisions. A key problem in gas-phase reactivity studies with HNO₃ is the inherent instability of this substance. HNO₃ decomposes in concentrated aqueous solutions. In earlier studies on the reactivity of ionic water clusters,^33–35 we worked with aqueous concentrated solutions of HNO₃, which yields a mixture of HNO₃ and H₂O in the reaction region, with traces of the decomposition product HONO. The observed reaction products confirmed that the abundance of HONO was less than 3% of the HNO₃ partial pressure. To identify reactions where HONO might play a role, we use quantum chemical calculations to identify thermochemically allowed reaction pathways. In addition, structural as well as thermochemical information is obtained for stationary points on the reaction potential energy surface. Together, a consistent picture of the reaction mechanism evolves.

Computational and experimental details

The ion–molecule reaction profiles were simulated with density functional theory at the M06-2X/6-311++G(d,p) level of theory employing the Gaussian09 program suite.³⁶ The energies of all optimized geometries were corrected with zero-point energy obtained from harmonic vibration analyses. Local minima and transition structures on the potential energy surface were confirmed with absence of and presence of one imaginary frequency, respectively. The local minima associated with each transition structure were verified by the intrinsic reaction coordinate (IRC) method. Spin density distributions were evaluated at the same level of theory and shown as isosurfaces at a value of 0.01 a.u. Table S1 in ESI,† compares the used methods/basis sets with experimental values. The coordinates are given in Table S4 (ESI†).

The experiments were performed on a modified Bruker/Spectrospin CMS47X FT-ICR mass spectrometer as described in detail elsewhere.^37–39 The spectrometer is equipped with a Bruker Infinity Cell, an APEX III data station, a 4.7 Tesla magnet and an external laser vaporization ion source. CO₃˙⁻ was produced in the laser vaporization ion source^39–42via expansion of a helium/water/oxygen/carbon dioxide mixture using a zinc target for the production of electrons. As vaporization laser a frequency doubled Nd:YAG laser at a pulse energy of ∼5 mJ at 10 Hz was used. All produced anionic species were transferred into the ICR cell, where they can be stored for several seconds or minutes. The ion of interest, CO₃˙⁻ or CO₃˙⁻(H₂O)_1,2, was then isolated via resonant excitation of unwanted ions prior to measuring the kinetics. A constant background pressure of HNO₃ and H₂O (concentrated aqueous solution, 70% HNO₃, Sigma-Aldrich) was introduced into the ultrahigh vacuum region via a leak valve. Due to the inherent instability of HNO₃, also traces of HONO were present, which are formed from the NO₂˙ decomposition product on thin films of H₂O and HNO₃, as present in the vacuum system.⁴³ Mass spectra were taken after different reaction delays relative to the end of the fill and isolation cycle. The intensities were extracted from the mass spectra. A rate coefficient matrix defines the allowed reaction channels and the data was fitted using a genetic algorithm that optimizes the rates from the matrix based on a pseudo-first order rate law. Relative rate coefficients were extracted from this fit. The stability of the fit was tested by systematically changing each parameter in 5 to 10 steps of ±5% from its optimized value, and re-optimizing all other parameters. The resulting error was plotted against the modified parameter. These plots are available as supporting information in Fig. S8 and S9 (ESI†). All rate coefficients reported in the results section showed stable, well-converged minima.

These pressure-dependent pseudo-first order rate coefficients are converted to pressure-independent bimolecular rate coefficients. An aqueous 70% HNO₃ solution is close to the azeotropic point and the chemical composition in the gas phase is therefore close to 70% HNO₃ and 30% water. As the cold cathode pressure gauge shows different sensitivity toward HNO₃ and H₂O, the measured pressure was corrected taking the different sensitivity into account. The HNO₃ partial pressure was taken as 70% of the corrected total pressure. More details on the kinetic analysis and pressure correction are available in ESI.† The accuracy of the absolute rates is estimated to be ±40% due to uncertainties in the pressure determination. The noise level is derived from the baseline of each mass spectrum.

Results and discussion

Calculated reaction potential energy surface

The potential energy surface (PES) corresponding to the reaction mechanisms suggested by Möhler and Arnold²² is shown in Fig. 1. The calculations show that a barrierless proton transfer takes place upon formation of the collision complex 1, which can be written as NO₃⁻(HCO₃˙). Rearrangement of the HCO₃˙ unit to CO₂ + OH˙ within the complex faces a significant barrier of 81 kJ mol⁻¹viaTS1 located at −63 kJ mol⁻¹ relative to the separated reactants. Evaporation of CO₂ from complex 2, which can be written as NO₃⁻(OH˙)(CO₂), requires only 33 kJ mol⁻¹, leading to the observed NO₃⁻(OH˙) product. Further evaporation of the OH˙ radical requires 68 kJ mol⁻¹, making the overall reaction with −3 kJ mol⁻¹ below the entrance channel almost thermoneutral.

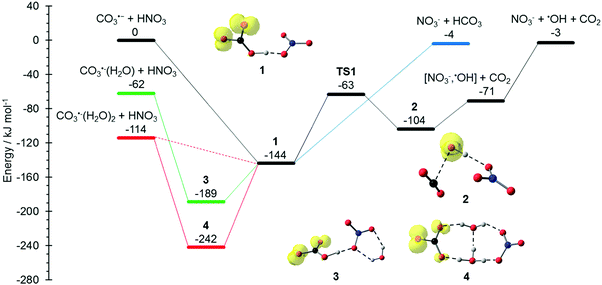Fig. 1 Reaction potential energy surface (PES) calculated on the M06-2X/6-311++G(d,p) level of theory, with zero-point corrected energies given in kJ mol⁻¹. More detailed results on the influence of solvation and additional reaction channels are given in the ESI,† Fig. S1–S4.

The water binding energies in CO₃˙⁻(H₂O)_1,2 are 62 kJ mol⁻¹ and 52 kJ mol⁻¹ for the first and second molecule, respectively. Upon collision with HNO₃, the water molecules from CO₃⁻(H₂O) and CO₃⁻(H₂O)₂ evaporate due to the energy released upon formation of the collision complex, since the transition states for rearrangement prior to water evaporation lie significantly higher in energy, see Fig. S1 (ESI†). For CO₃˙⁻(H₂O), the pathway to NO₃⁻(OH˙) + CO₂ formation is still accessible, while bare NO₃⁻ formation is clearly out of reach, lying 59 kJ mol⁻¹ above the CO₃˙⁻(H₂O) + HNO₃ entrance channel. The second water molecule reduces the available energy further, only the formation of the NO₃⁻(HCO₃˙) complex 1 is thermochemically allowed, with a moderate exothermicity of −30 kJ mol⁻¹.

Experimental results and discussion

CO₃⁻. The kinetics of the reaction of CO₃˙⁻ with HNO₃ is displayed in Fig. 2. A mass spectrum is available in Fig. S5 (ESI†). The decay of the CO₃˙⁻ ion intensity follows a pseudo-first order kinetics behavior. Primary reactions for pure CO₃˙⁻ with the reaction mixture lead to formation of HCO₃⁻, NO₃⁻, and NO₃⁻(OH˙). Rate coefficients for all reactions are given in Table 1. Compared to the flow reactor studies, the reaction is an order of magnitude slower, with an overall rate for all reaction channels of 1.0 × 10⁻¹⁰ cm³ s⁻¹. Fehsenfeld et al.²¹ reported a value of 8 × 10⁻¹⁰ cm³ s⁻¹ in their flowing afterglow, while Möhler and Arnold²² agreed with their value of 1.3 × 10⁻⁹ cm³ s⁻¹ within error limits with Guimbaud et al.,³² who reported 1.2 ± 0.3 × 10⁻⁹ cm³ s⁻¹. Obviously, stabilizing collisions with the buffer gas in the flow reactor studies increase the efficiency of the reaction. The nitrate–hydroxyl radical complex arises from the reaction with gaseous HNO₃ according to reaction (5). Formation of HCO₃⁻ requires a hydrogen atom transfer (HAT), which is significantly endothermic for HNO₃, reaction (6). We therefore assign this small product to traces of HONO, reaction (7), which is exothermic. The calculations show a barrierless HAT from HONO to CO₃⁻. If we assume collision efficiency for reaction (7), a partial pressure below 1 × 10⁻¹⁰ mbar of the HONO background is sufficient to explain the observed abundance of HCO₃⁻.

CO₃˙⁻ + HNO₃ → NO₃⁻(OH˙) + CO₂ ΔH₀ = −71 kJ mol⁻¹ (5)

CO₃˙⁻ + HNO₃ → HCO₃⁻ + NO₃˙ ΔH₀ = +48 kJ mol⁻¹ (6)

CO₃˙⁻ + HONO → HCO₃⁻ + NO₂˙ ΔH₀ = −68 kJ mol⁻¹ (7)

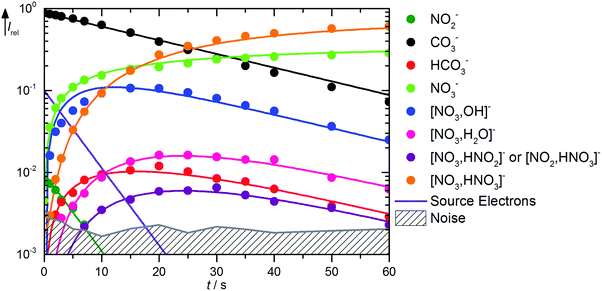Fig. 2 Kinetics of the reaction of CO₃˙⁻ with HNO₃ and H₂O, with traces of HONO, at a HNO₃ partial pressure of 1.4 × 10⁻⁸ mbar. The lines represent the fit with the rate coefficients from Table S1 (ESI†). Selected mass spectra are shown in Fig. S5 (ESI†).

Table 1 Bimolecular rate coefficients k_abs for the reactions discussed in the text

Reaction		Rate coefficient kabs/cm^3 s^−1
a Assuming either reaction (11) or (12). b Thermalized fraction of CO3˙^−(H2O). c Cold fraction of CO3˙^−(H2O); the accuracy of the absolute rates is estimated to be ±40% due to uncertainties in the pressure determination.
(5)	CO3˙^− + HNO3 → NO3^−(OH˙) + CO2	8.9 × 10^−11
(8/9)	CO3˙^− + HNO3 → NO3^− + [CO2,OH˙]	2.1 × 10^−11
(10)	HCO3^− + HNO3 → NO3^− + CO2 + H2O	2.8 × 10^−10
(11)	NO3^−(OH˙) + H2O → NO3^−(H2O) + OH˙	1.2 × 10^−10^a
(12)	NO3^−(OH˙) + HNO3 → NO3^−(H2O) + NO3	5.3 × 10^−11^a
(14)	NO3^−(OH˙) + HNO3 → NO3^−(HNO3) + OH˙	3.6 × 10^−10
(16)	NO2^− + HNO3 → NO3^− + HONO	7.2 × 10^−9
(18)	CO3˙^−(H2O) + HNO3 → NO3^−(OH˙) + CO2 + H2O	1.4 × 10^−10^b
(19)	CO3˙^−(H2O) + HNO3 → NO3^−(HCO3˙) + H2O	9.4 × 10^−11^c
		1.4 × 10^−10^b
(20)	CO3˙^−(H2O) + HNO3 → NO3^− + CO2 + OH˙ + H2O	3.8 × 10^−10^b

---

Formation of NO₃⁻viareactions (8) or (9) with HNO₃ as neutral reactant is only slightly exothermic. Given that both reactants have low-lying vibrational modes, some extra thermal energy is available, which makes the reactions entirely plausible.

CO₃˙⁻ + HNO₃ → NO₃⁻ + CO₂ + OH˙ ΔH₀ = −3 kJ mol⁻¹ (8)

CO₃˙⁻ + HNO₃ → NO₃⁻ + HCO₃˙ ΔH₀ = −4 kJ mol⁻¹ (9)

According to our kinetics fit, several secondary reactions are observed. HCO₃⁻ reacts to NO₃⁻, most likely via a straightforward proton transfer from HNO₃, reaction (10).

HCO₃⁻ + HNO₃ → NO₃⁻ + CO₂ + H₂O ΔH₀ = −88 kJ mol⁻¹ (10)

NO₃⁻(OH˙) either undergoes ligand exchange with H₂O or HAT from HNO₃ to form NO₃⁻(H₂O), reactions (11) or (12), respectively. Ligand exchange is also possible with HONO as well as HNO₃, reactions (13) and (14), respectively. Since reactions (12) and (14) compete with each other, with reaction (14) being both more exothermic and mechanistically favorable, ligand exchange with water is the most plausible origin of the NO₃⁻(H₂O) product, reaction (11). Reaction (13) validates the presence of HONO in the reaction mixture.

NO₃⁻(OH˙) + H₂O → NO₃⁻(H₂O) + OH˙ ΔH₀ = +3 kJ mol⁻¹ (11)

NO₃⁻(OH˙) + HNO₃ → NO₃⁻(H₂O) + NO₃ ΔH₀ = −34 kJ mol⁻¹ (12)

NO₃⁻(OH˙) + HONO → NO₃⁻(HONO) + OH˙ ΔH₀ = −40 kJ mol⁻¹ (13)

NO₃⁻(OH˙) + HNO₃ → NO₃⁻(HNO₃) + OH˙ ΔH₀ = −75 kJ mol⁻¹ (14)

We also observe traces of NO₂⁻, which are assigned to dissociative electron attachment. When working with negative ions, some free electrons are usually trapped in the ICR cell,⁴⁴ which react with HNO₃ according to reaction (15).²¹ In turn, NO₂⁻ is converted with near collision rate to NO₃⁻via proton transfer from HNO₃, reaction (16).

e⁻ + HNO₃ → NO₂⁻ + OH˙ (15)

NO₂⁻ + HNO₃ → NO₃⁻ + HONO (16)

CO₃˙⁻(H₂O). Fig. 3 shows the kinetics of the monohydrated species, mass spectra are displayed in Fig. S6 (ESI†). The scenario is quite complex, with a pronounced deviation of the CO₃˙⁻(H₂O) intensity from pseudo-first order behavior, for which a linear graph in the semi-logarithmic plot is expected. To describe the observed curvature, the population of this species is divided into two fractions, which correspond to the initially cold ions directly from the supersonic expansion, and the thermalized ions after heating by ambient black-body radiation and collision with the reaction gas. These effects are included in the kinetic model by allowing the cold fraction to be converted to the thermalized fraction, with a unimolecular rate treated as a fit parameter. Heating ultimately leads to formation of CO₃˙⁻via black-body infrared radiative dissociation (BIRD),^45–58reaction (17), with a lifetime of 3 s for the thermalized fraction. This reaction is not allowed for the cold fraction in the kinetic model, see Table S2 (ESI†). Since the major part of the CO₃˙⁻(H₂O) population is already thermalized at nominally t = 0 s, and the thermalized fraction reacts overall much faster than the cold fraction, the intensity drop of CO₃˙⁻(H₂O) is faster in the beginning, and levels off at later times because the conversion from the cold to the hot fraction becomes rate limiting, which explains the observed curvature.

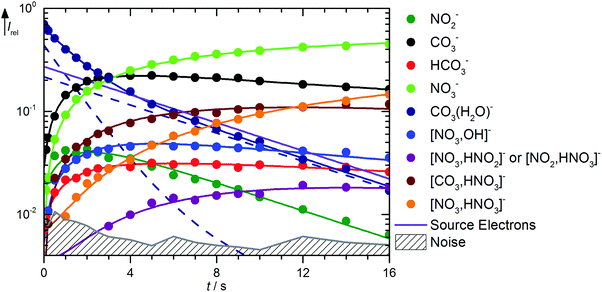Fig. 3 Kinetics of the reaction of CO₃˙⁻(H₂O) with HNO₃ and H₂O, with traces of HONO, at a HNO₃ partial pressure of 2.1 × 10⁻⁸ mbar. The lines represent the fit with the rate coefficients from Table S2 (ESI†). Selected mass spectra are shown in Fig. S6 (ESI†).

The formation of NO₃⁻(OH˙) is observed with an appreciable rate, and can be assigned to collisions with HNO₃, reaction (18). Also the transition state for CO₂ formation is below the entrance channel, see Fig. 1, thus reaction (18) is fully consistent with the calculations. The same is true for the ligand exchange product NO₃⁻(HCO₃˙) formed viareaction (19). NO₃⁻ is exclusively formed from the thermalized fraction of CO₃˙⁻(H₂O) as a primary product in the kinetics. With HNO₃ as the reaction partner, reaction (20) is with ΔH₀ = +59 kJ mol⁻¹ significantly endothermic. However, the thermalized fraction of CO₃˙⁻(H₂O) already contains almost enough energy for dissociation. Together with the internal energy of the HNO₃ collision partner, the energy required for reaction (20) is available in the system. Reactions (18)–(20) yield a total rate coefficient of 6.3 × 10⁻¹⁰ cm³ s⁻¹ for the reaction of thermalized CO₃˙⁻(H₂O) with HNO₃, 37% of the flow reactor value of 1.7 × 10⁻⁹ cm³ s⁻¹ reported by Möhler and Arnold.²² All secondary reactions as well as NO₂⁻ formation via dissociative electron attachment proceed as discussed above.

CO₃˙⁻(H₂O) → CO₃˙⁻ + H₂O ΔH₀ = +62 kJ mol⁻¹ (17)

CO₃˙⁻(H₂O) + HNO₃ → NO₃⁻(OH^•) + CO₂ + H₂O ΔH₀ = −9 kJ mol⁻¹ (18)

CO₃˙⁻(H₂O) + HNO₃ → NO₃⁻(HCO₃˙) + H₂O ΔH₀ = −83 kJ mol⁻¹ (19)

CO₃˙⁻(H₂O) + HNO₃ → NO₃⁻ + CO₂ + OH˙ + H₂O ΔH₀ = +59 kJ mol⁻¹ (20)

CO₃˙⁻(H₂O)₂. Solvation of CO₃˙⁻ with two water molecules leads to a significantly reduced reactivity with HNO₃. The kinetics is shown in Fig. 4, mass spectra are available in Fig. S7 (ESI†). The major reaction channel is, as might be expected, loss of a water molecule due to BIRD, reaction (21). There is again formation of NO₂⁻viareaction (15), but otherwise no primary products are observed from CO₃˙⁻(H₂O)₂. The noise level of the kinetics, however, places a high upper limit of 6.0 × 10⁻¹¹ cm³ s⁻¹ on the rate coefficient for the direct reaction of CO₃˙⁻(H₂O)₂ with HNO₃. According to the thermochemical arguments discussed above, the most likely product of such a reaction would be NO₃⁻(HCO₃˙), followed by NO₃⁻(OH˙), while direct NO₃⁻ formation this time is clearly out of reach.

CO₃˙⁻(H₂O)₂ → CO₃˙⁻(H₂O) + H₂O ΔH₀ = +52 kJ mol⁻¹ (21)

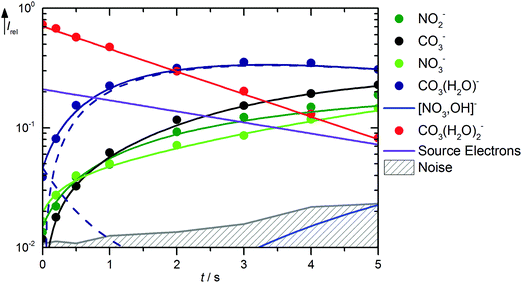Fig. 4 Kinetics of the reaction of CO₃˙⁻(H₂O)₂ with HNO₃ and H₂O, with traces of HONO, at a HNO₃ partial pressure of 8.1 × 10⁻⁹ mbar. The lines represent the fit with the rate coefficients from Table S3 (ESI†). Selected mass spectra are shown in Fig. S7 (ESI†).

Conclusions

The mechanism of the reaction of HNO₃ with CO₃˙⁻(H₂O)_n, n = 0, 1, 2, is very similar to the previously studied HCl reaction.³¹ With bare CO₃˙⁻, the reaction is relatively slow, and the proton-transfer intermediate NO₃⁻(OH˙) is the unambiguously identified primary product, corresponding to Cl⁻(OH˙) in the HCl reaction. Also NO₃⁻ is directly formed, but with even lower rate. Formation of NO₃⁻(OH˙) is accelerated for CO₃˙⁻(H₂O), but again slowed down by a second water molecule in the hydration shell. The rate is clearly dependent on the number of water molecules solvating CO₃˙⁻, and overall significantly lower than in the flow reactor studies previously reported in the literature, indicating a strong pressure dependence.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

M. K. B. acknowledges startup funds from the University of Innsbruck. C. K. S. thanks Research Grants Council, Hong Kong SAR (Project no. 11300917) and City University of Hong Kong (CityU) (Project no. 7004401) for their financial support. W. K. T. acknowledges Chow Yei Ching School of Graduate Studies, CityU for his postgraduate studentships, outstanding academic performance awards, research tuition scholarships and overseas research activities award.

References

1. G. Beig and G. P. Brasseur, J. Geophys. Res.: Atmos., 2000, 105, 22671–22684.
2. M. L. Huertas, J. Fontan and J. Gonzalez, Atmos. Environ., 1978, 12, 2351–2362.
3. H. Kawamoto and T. Ogawa, Planet. Space Sci., 1986, 34, 1229–1239.
4. A. Hirsikko, T. Nieminen, S. Gagné, K. Lehtipalo, H. E. Manninen, M. Ehn, U. Hõrrak, V.-M. Kerminen, L. Laakso, P. H. McMurry, A. Mirme, S. Mirme, T. Petäjä, H. Tammet, V. Vakkari, M. Vana and M. Kulmala, Atmos. Chem. Phys., 2011, 11, 767–798.
5. J. Kirkby, J. Duplissy, K. Sengupta, C. Frege, H. Gordon, C. Williamson, M. Heinritzi, M. Simon, C. Yan, J. Almeida, J. Tröstl, T. Nieminen, I. K. Ortega, R. Wagner, A. Adamov, A. Amorim, A.-K. Bernhammer, F. Bianchi, M. Breitenlechner, S. Brilke, X. Chen, J. Craven, A. Dias, S. Ehrhart, R. C. Flagan, A. Franchin, C. Fuchs, R. Guida, J. Hakala, C. R. Hoyle, T. Jokinen, H. Junninen, J. Kangasluoma, J. Kim, M. Krapf, A. Kürten, A. Laaksonen, K. Lehtipalo, V. Makhmutov, S. Mathot, U. Molteni, A. Onnela, O. Peräkylä, F. Piel, T. Petäjä, A. P. Praplan, K. Pringle, A. Rap, N. A. D. Richards, I. Riipinen, M. P. Rissanen, L. Rondo, N. Sarnela, S. Schobesberger, C. E. Scott, J. H. Seinfeld, M. Sipilä, G. Steiner, Y. Stozhkov, F. Stratmann, A. Tomé, A. Virtanen, A. L. Vogel, A. C. Wagner, P. E. Wagner, E. Weingartner, D. Wimmer, P. M. Winkler, P. Ye, X. Zhang, A. Hansel, J. Dommen, N. M. Donahue, D. R. Worsnop, U. Baltensperger, M. Kulmala, K. S. Carslaw and J. Curtius, Nature, 2016, 533, 521–526.
6. K. Lehtipalo, L. Rondo, J. Kontkanen, S. Schobesberger, T. Jokinen, N. Sarnela, A. Kürten, S. Ehrhart, A. Franchin, T. Nieminen, F. Riccobono, M. Sipilä, T. Yli-Juuti, J. Duplissy, A. Adamov, L. Ahlm, J. Almeida, A. Amorim, F. Bianchi, M. Breitenlechner, J. Dommen, A. J. Downard, E. M. Dunne, R. C. Flagan, R. Guida, J. Hakala, A. Hansel, W. Jud, J. Kangasluoma, V.-M. Kerminen, H. Keskinen, J. Kim, J. Kirkby, A. Kupc, O. Kupiainen-Määttä, A. Laaksonen, M. J. Lawler, M. Leiminger, S. Mathot, T. Olenius, I. K. Ortega, A. Onnela, T. Petäjä, A. Praplan, M. P. Rissanen, T. Ruuskanen, F. D. Santos, S. Schallhart, R. Schnitzhofer, M. Simon, J. N. Smith, J. Tröstl, G. Tsagkogeorgas, A. Tomé, P. Vaattovaara, H. Vehkamäki, A. E. Vrtala, P. E. Wagner, C. Williamson, D. Wimmer, P. M. Winkler, A. Virtanen, N. M. Donahue, K. S. Carslaw, U. Baltensperger, I. Riipinen, J. Curtius, D. R. Worsnop and M. Kulmala, Nat. Commun., 2016, 7, 11594.
7. J. Tröstl, W. K. Chuang, H. Gordon, M. Heinritzi, C. Yan, U. Molteni, L. Ahlm, C. Frege, F. Bianchi, R. Wagner, M. Simon, K. Lehtipalo, C. Williamson, J. S. Craven, J. Duplissy, A. Adamov, J. Almeida, A.-K. Bernhammer, M. Breitenlechner, S. Brilke, A. Dias, S. Ehrhart, R. C. Flagan, A. Franchin, C. Fuchs, R. Guida, M. Gysel, A. Hansel, C. R. Hoyle, T. Jokinen, H. Junninen, J. Kangasluoma, H. Keskinen, J. Kim, M. Krapf, A. Kürten, A. Laaksonen, M. Lawler, M. Leiminger, S. Mathot, O. Möhler, T. Nieminen, A. Onnela, T. Petäjä, F. M. Piel, P. Miettinen, M. P. Rissanen, L. Rondo, N. Sarnela, S. Schobesberger, K. Sengupta, M. Sipilä, J. N. Smith, G. Steiner, A. Tomè, A. Virtanen, A. C. Wagner, E. Weingartner, D. Wimmer, P. M. Winkler, P. Ye, K. S. Carslaw, J. Curtius, J. Dommen, J. Kirkby, M. Kulmala, I. Riipinen, D. R. Worsnop, N. M. Donahue and U. Baltensperger, Nature, 2016, 533, 527–531.
8. E. M. Dunne, H. Gordon, A. Kürten, J. Almeida, J. Duplissy, C. Williamson, I. K. Ortega, K. J. Pringle, A. Adamov, U. Baltensperger, P. Barmet, F. Benduhn, F. Bianchi, M. Breitenlechner, A. Clarke, J. Curtius, J. Dommen, N. M. Donahue, S. Ehrhart, R. C. Flagan, A. Franchin, R. Guida, J. Hakala, A. Hansel, M. Heinritzi, T. Jokinen, J. Kangasluoma, J. Kirkby, M. Kulmala, A. Kupc, M. J. Lawler, K. Lehtipalo, V. Makhmutov, G. Mann, S. Mathot, J. Merikanto, P. Miettinen, A. Nenes, A. Onnela, A. Rap, C. L. S. Reddington, F. Riccobono, N. A. D. Richards, M. P. Rissanen, L. Rondo, N. Sarnela, S. Schobesberger, K. Sengupta, M. Simon, M. Sipilä, J. N. Smith, Y. Stozkhov, A. Tomé, J. Tröstl, P. E. Wagner, D. Wimmer, P. M. Winkler, D. R. Worsnop and K. S. Carslaw, Science, 2016, 354, 1119–1124.
9. N. G. Adams, D. K. Bohme, D. B. Dunkin, F. C. Fehsenfeld and E. E. Ferguson, J. Chem. Phys., 1970, 52, 3133–3140.
10. M. E. Jacox and D. E. Milligan, J. Mol. Spectrosc., 1974, 52, 363–379.
11. J. T. Moseley, J. Chem. Phys., 1976, 65, 2512–2517.
12. R. A. Beyer and J. A. Vanderhoff, J. Chem. Phys., 1976, 65, 2313–2321.
13. D. E. Hunton, M. Hofmann, T. G. Lindeman and A. W. Castleman, J. Chem. Phys., 1985, 82, 134–150.
14. J. T. Snodgrass, C. M. Roehl, P. A. M. van Koppen, W. E. Palke and M. T. Bowers, J. Chem. Phys., 1990, 92, 5935–5943.
15. J. C. Bopp, E. G. Diken, J. M. Headrick, J. R. Roscioli, M. A. Johnson, A. J. Midey and A. A. Viggiano, J. Chem. Phys., 2006, 124, 174302.
16. A. A. Viggiano, Phys. Chem. Chem. Phys., 2006, 8, 2557–2571.
17. A. A. Viggiano, A. J. Midey and A. Ehlerding, Int. J. Mass Spectrom., 2006, 255, 65–70.
18. G. Beig, S. Walters and G. Brasseur, J. Geophys. Res., 1993, 98, 12775.
19. I. Dotan, J. A. Davidson, G. E. Streit, D. L. Albritton and F. C. Fehsenfeld, J. Chem. Phys., 1977, 67, 2874–2879.
20. N. Schoon, C. Amelynck, P. Bultinck and E. Arijs, Int. J. Mass Spectrom., 2002, 221, 209–218.
21. F. C. Fehsenfeld, C. J. Howard and A. L. Schmeltekopf, J. Chem. Phys., 1975, 63, 2835–2841.
22. O. Möhler and F. Arnold, J. Atmos. Chem., 1991, 13, 33–61.
23. N. S. Shuman, D. E. Hunton and A. A. Viggiano, Chem. Rev., 2015, 115, 4542–4570.
24. I. Dotan, D. L. Albritton, F. C. Fehsenfeld, G. E. Streit and E. E. Ferguson, J. Chem. Phys., 1978, 68, 5414–5416.
25. M. L. Huertas and J. Fontan, Atmos. Environ., 1982, 16, 2521–2527.
26. H. Kawamoto and T. Ogawa, Planet. Space Sci., 1984, 32, 1223–1233.
27. K. Nagato, Y. Matsui, T. Miyata and T. Yamauchi, Int. J. Mass Spectrom., 2006, 248, 142–147.
28. J. Viidanoja, T. Reiner and F. Arnold, Int. J. Mass Spectrom., 1998, 181, 31–41.
29. J. Viidanoja, T. Reiner, A. Kiendler, F. Grimm and F. Arnold, Int. J. Mass Spectrom., 2000, 194, 53–68.
30. C. van der Linde, W. K. Tang, C.-K. Siu and M. K. Beyer, Chem. – Eur. J., 2016, 22, 12684–12687.
31. W. K. Tang, C. van der Linde, C.-K. Siu and M. K. Beyer, J. Phys. Chem. A, 2017, 121, 192–197.
32. C. Guimbaud, D. Labonnette, V. Catoire and R. Thomas, Int. J. Mass Spectrom., 1998, 178, 161–171.
33. J. Lengyel, J. Med, P. Slavíček and M. K. Beyer, J. Chem. Phys., 2017, 147, 101101.
34. J. Lengyel, M. Ončák, J. Fedor, J. Kočišek, A. Pysanenko, M. K. Beyer and M. Fárník, Phys. Chem. Chem. Phys., 2017, 19, 11753–11758.
35. J. Lengyel, C. van der Linde, A. Akhgarnusch and M. K. Beyer, Int. J. Mass Spectrom., 2017, 418, 101–106.
36. M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, G. A. Petersson, H. Nakatsuji, X. Li, M. Caricato, A. Marenich, J. Bloino, B. G. Janesko, R. Gomperts, B. Mennucci, H. P. Hratchian, J. V. Ortiz, A. F. Izmaylov, J. L. Sonnenberg, D. Williams-Young, F. Ding, F. Lipparini, F. Egidi, J. Goings, B. Peng, A. Petrone, T. Henderson, D. Ranasinghe, V. G. Zakrzewski, J. Gao, N. Rega, G. Zheng, W. Liang, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, K. Throssell, J. A. Montgomery, Jr., J. E. Peralta, F. Ogliaro, M. Bearpark, J. J. Heyd, E. Brothers, K. N. Kudin, V. N. Staroverov, T. Keith, R. Kobayashi, J. Normand, K. Raghavachari, A. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, J. M. Millam, M. Klene, C. Adamo, R. Cammi, J. W. Ochterski, R. L. Martin, K. Morokuma, O. Farkas, J. B. Foresman and D. J. Fox, Gaussian 09, Revision A.02, 2016.
37. R. F. Höckendorf, O. P. Balaj, C. van der Linde and M. K. Beyer, Phys. Chem. Chem. Phys., 2010, 12, 3772–3779.
38. P. Kofel, M. Allemann, H. Kellerhals and K. P. Wanczek, Int. J. Mass Spectrom. Ion Processes, 1986, 72, 53–61.
39. C. Berg, T. Schindler, G. Niedner-Schatteburg and V. E. Bondybey, J. Chem. Phys., 1995, 102, 4870–4884.
40. S. Maruyama, L. R. Anderson and R. E. Smalley, Rev. Sci. Instrum., 1990, 61, 3686–3693.
41. V. E. Bondybey and J. H. English, J. Chem. Phys., 1981, 74, 6978–6979.
42. T. G. Dietz, M. A. Duncan, D. E. Powers and R. E. Smalley, J. Chem. Phys., 1981, 74, 6511–6512.
43. D. de Jesus Medeiros and A. S. Pimentel, J. Phys. Chem. A, 2011, 115, 6357–6365.
44. A. Akhgarnusch, R. F. Höckendorf and M. K. Beyer, J. Phys. Chem. A, 2015, 119, 9978–9985.
45. O. P. Balaj, C. B. Berg, S. J. Reitmeier, V. E. Bondybey and M. K. Beyer, Int. J. Mass Spectrom., 2009, 279, 5–9.
46. T. E. Cooper, J. T. O’Brien, E. R. Williams and P. B. Armentrout, J. Phys. Chem. A, 2010, 114, 12646–12655.
47. R. C. Dunbar, J. Phys. Chem., 1994, 98, 8705–8712.
48. R. C. Dunbar, Mass Spectrom. Rev., 2004, 23, 127–158.
49. R. C. Dunbar and T. B. McMahon, Science, 1998, 279, 194–197.
50. B. S. Fox, M. K. Beyer and V. E. Bondybey, J. Phys. Chem. A, 2001, 105, 6386–6392.
51. A. Kamariotis, O. V. Boyarkin, S. R. Mercier, R. D. Beck, M. F. Bush, E. R. Williams and T. R. Rizzo, J. Am. Chem. Soc., 2006, 128, 905–916.
52. E. N. Kitova, D. R. Bundle and J. S. Klassen, J. Am. Chem. Soc., 2002, 124, 5902–5913.
53. S. W. Lee, P. Freivogel, T. Schindler and J. L. Beauchamp, J. Am. Chem. Soc., 1998, 120, 11758–11765.
54. T. Schindler, C. Berg, G. Niedner-Schatteburg and V. E. Bondybey, Chem. Phys. Lett., 1996, 250, 301–308.
55. P. D. Schnier, W. D. Price, R. A. Jockusch and E. R. Williams, J. Am. Chem. Soc., 1996, 118, 7178–7189.
56. M. Sena and J. M. Riveros, Rapid Commun. Mass Spectrom., 1994, 8, 1031–1034.
57. D. Thölmann, D. S. Tonner and T. B. McMahon, J. Phys. Chem., 1994, 98, 2002–2004.
58. R. L. Wong, K. Paech and E. R. Williams, Int. J. Mass Spectrom., 2004, 232, 59–66.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c7cp07773d

---