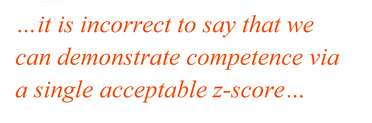z-Scores and other scores in chemical proficiency testing—their meanings, and some common misconceptions

Abstract

z-Scores were devised to provide a transparent but widely-applicable scoring system for participants in proficiency tests for analytical laboratories. The essential idea is to provide an appropriate scaling of the difference between a participant’s result and the ‘assigned value’ for the concentration of the analyte. Interpretation of a z-score is straightforward but some aspects need careful attention to avoid misconception. Over time several related scores have been devised to cope with a diversified range of applications. The main types of score have recently been codified in ISO 13528 (2015).

---

Proficiency tests are regular interlaboratory studies designed to identify a noteworthy inaccuracy in any participant's result. Wherever possible, results are converted into scores, the purpose of which is to provide a basis for instigating remedial action where necessary. Initially there was a diversity of scoring methods based on different arbitrary transformations of the result. However, it was soon evident that a single straightforward scoring method would allow analysts to interpret a score uniformly across different test materials, analytes, concentration ranges, and measurement principles, even across different proficiency testing schemes.¹ This insight gave rise to the ISO/IUPAC/AOAC Harmonised Protocol.^2,3 So in the beginning (or shortly after) there was the z-score and the q-score (or their equivalents) and everyone understood them.

The widespread proliferation of proficiency testing in the wake of accreditation, however, generated the need for some small variations on the z-scoring theme to cope with different applications. As an outcome there are now z-scores, z′-scores, zeta (ζ) scores, z_L-scores, D-scores and E_n scores. Successive authors and documents have used old names for new meanings and new names and symbols for old meanings. That's confusing. So let's have a quick look at the current state of play, as laid down in ISO 13528 (2015).⁴

The z-score

The z-score, probably the most widely used score, given by z = (x − x_pt)/σ_pt, is calculated from the participant's result x, the assigned value x_pt, and σ_pt, the ‘standard deviation for proficiency testing’ (SDPT). The scheme provider determines the numerical values for x_pt and σ_pt. z-Scores are typically interpreted as questionable outside the range ±2 and actionable outside the range ±3 (the rationale for this interpretation is described below in the section ‘What does a z-score tell us?’).

The assigned value is the provider's best available estimate of the true quantity value, often a participant consensus. An assumption underlying the z-score is that the uncertainty on the assigned value is negligible in comparison with that on the participant's result. The SDPT (originally called the ‘target value’) is best taken as the standard uncertainty that is regarded as optimally fit for purpose in the relevant sector (see AMCTB No. 68) and must be known in advance by the participants. Other options for evaluating the SDPT are recognised by the ISO standard but all have one or more practical shortcomings.

The D and D% score and δ_E

This score, D = (x − x_pt)/x_pt (and the derived D% = 100D), the relative difference of the result from x_pt, was until recently called the q-score.²D% is essentially the relative deviation from the assigned value, expressed as a percentage—familiar to analysts but lacking a ‘fitness-for-purpose’ interpretation unless the reader already understands typical performance in terms of the desired relative standard deviation. It has the benefit of simplicity but two drawbacks: (a) scores from different concentration ranges for the same determination may not be comparable, and (b) it does not address fitness for purpose. The latter shortcoming can be overcome by introducing an extra term δ_E, although that in effect merely converts the D-score into a z-score.

The z′-score

A modification to the z-score,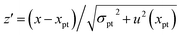 , is suggested for use when the uncertainty u(x_pt) on an independent assigned value is sufficiently large to affect the z-score appreciably. This is usually taken to happen when u(x_pt) > 0.3σ_pt, in which case the z-score would be reduced by more than 4% relative. The z′-score correctly serves to standardise the deviation from the assigned value, but fails to differentiate between a poor result and a poor assigned value.

The zeta-score and E_n

The zeta score, can be used in instances where the participant submits a result with an uncertainty estimate u(x), and the assigned value x_pt is a certified reference value with an uncertainty u(x_pt). Like z, zeta scores outside ±2 are often regarded as questionable and values outside ±3 are cause for action or at least concern.

Zeta scores increase as either the deviation from the assigned value increases or as the reported uncertainty gets smaller, so a larger zeta score can indicate a large error, an underestimated uncertainty, or both. This ambiguity leaves the zeta score open to improper manipulation should participants choose to reduce their score by overstating u(x).

E _n is essentially similar to the zeta score but replaces the standard uncertainties with expanded uncertainties. E_n scores are therefore about half of the corresponding zeta scores, so a value outside ±1 is usually taken as questionable. E_n is used more in calibration laboratories than analytical laboratories.

The z_L-score

This score, z_L = (x − x_pt)/u_f, was devised to accommodate participants for whom the scheme provider's SDPT was inappropriate for a particular customer's use of the result (see AMCTB No. 2). The essential idea is for the participant and customer jointly to set a different SDPT, a standard uncertainty u_f that is appropriate to the application, and to use that to calculate the modified score. Use of a z_L-score would be a scientifically sound practice so long as (a) its origin was made clear to third parties and (b) the PT scheme's published assigned value was used in the calculation (note: this score is not part of ISO 13528. It was originally called the ‘zeta (ζ)-score’, but that term was subsequently appropriated for other purposes (see above)).

What does a z-score tell us?

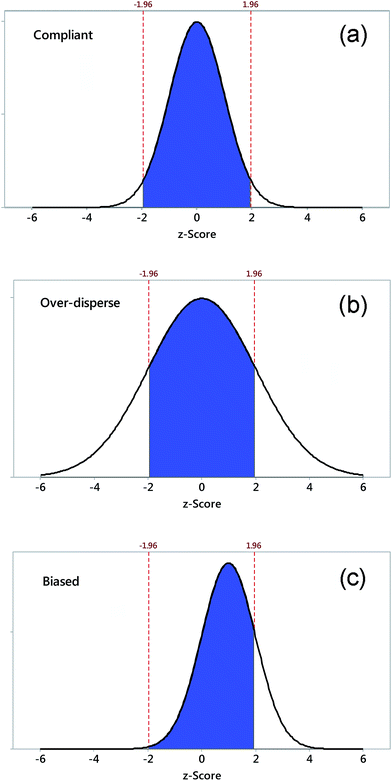
z-Scores are interpreted as if successive outcomes for a compliant participant laboratory were drawn at random from a normal distribution with zero mean and unit standard deviation. In such a laboratory, scores outside the range ±2 would occur with a probability of about 0.05, which, as an isolated event should be interpreted as no more than a warning limit. Scores outside the range ±3 would be much rarer under the standard normal assumption (p ≈ 0.003) and can safely be taken as action limits to instigate an investigation into the cause of the problem. Non-compliant laboratories, namely those with a dispersion greater than σ_pt or a biased mean, could expect a higher proportions of scores outside those ranges. Fig. 1 illustrates these possibilities.

Fig. 1 Distribution of z-scores expected from participant laboratories that are: (a) exactly compliant with the scheme's assigned value and SDPT criterion, (b) compliant with the assigned value but over-disperse, and (c) compliant with the scheme's SDPT criterion but biased. Areas shaded blue indicate the proportions of ‘satisfactory’ results, namely those with −1.96 < z < 1.96. The proportions are: (a) 0.95, (b) 0.67, and (c) 0.83.

It is essential to emphasise that interpreting z-scores thus does not assume the participants' results in a round are normally distributed. That is a common misconception among statisticians and regulators unfamiliar with proficiency testing. The interpretation of z-scores relies rather on the idea that, if all the laboratories performed similarly and exactly in accordance with the requirement set by the assigned value and the SDPT, their results would be approximately normally distributed with mean x_pt and standard deviation σ_pt. z-Scores would then show a normal distribution with zero mean and unit standard deviation. Notice that this does not assume that the actual participant results are normally distributed; only that idealised performance from all participants would have led to a standard normal distribution of scores. So over time, z-scores compare a participant with the PT provider's criterion of good performance.

In the longer term

Proficiency testing can readily demonstrate bad performance, but has far less power to demonstrate competence. While a z-score outside the range of ±3 clearly calls for action, a score within the range of ±2 does not in itself say that all is well. Fig. 1 shows why. Even with an uncertainty of twice σ_pt, a participant would still receive a z-score in the range of ±2 with a probability of about 0.67. With a compliant standard deviation but a bias equal to σ_pt, the probability of a score within the range of ±2 would be about 0.83. That is why it is incorrect to say that we can demonstrate competence via a single acceptable z-score, or even several in succession. We have to undertake a longer-term view of z-scores in combination with other factors to demonstrate competence.

A simple and effective long-term view for a participant is provided by plotting successive z-scores on a control chart based on a zero mean and unit standard deviation, either a Shewhart chart or, better still, a range chart (see AMCTB Nos. 12 and 16).

Michael Thompson (Birkbeck University of London)

This Technical Brief was prepared for the Analytical Methods Committee and approved on 14/05/16.

References

1. Analytical Methods Committee, Analyst, 1992, 117, 97–104.
2. M. Thompson and R. Wood, Pure Appl. Chem., 1993, 65, 2123–2144.
3. M. Thompson, S. L. R. Ellison and R. Wood, Pure Appl. Chem., 2006, 78, 145–196.
4. ISO 13528, 2015.

---