Tuning the optical, electronic and thermal properties of Cu3NbS4−xSex through chemical substitution†
Abstract
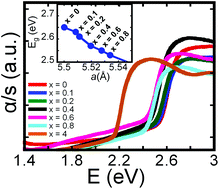
Several compositions of the Cu3NbS4−xSex (x = 0, 0.1, 0.2, 0.4, 0.6, 0.8, and 4) series were synthesized through combination of the elements using conventional solid-state reactions. X-ray powder diffraction patterns suggest that the synthesized polycrystalline powder samples are isostructural to sulvanite (Cu3VS4). However, plotting the refined lattice parameters as well as the measured “true” density as a function of Se content (x) showed deviations from linearity, suggesting that the Cu3NbS4−xSex series is not a “true” solid solution. Differential scanning calorimetry data showed that all compounds incongruently melt at ∼1100 K and recrystallize with a minor impurity phase. Optical diffuse reflectance UV/Vis/NIR spectroscopy revealed a nearly linear contraction of the optical band gap with increasing Se content. The direct band gap decreases from 2.53 eV for x = 0.1 to 2.43 eV for x = 0.8. Electronic transport data indicate p-type semiconducting behavior for all samples. Thermal conductivity data showed a rather surprising trend, with the substituted compositions exhibiting higher thermal conductivity than the two end members.
- This article is part of the themed collections: In honour of Mercouri G. Kanatzidis for his contributions to Inorganic Chemistry for over 30 years and Inorganic Chemistry Frontiers HOT articles for 2017


 Please wait while we load your content...
Please wait while we load your content...