Soft, skin-mounted microfluidic systems for measuring secretory fluidic pressures generated at the surface of the skin by eccrine sweat glands†
Abstract
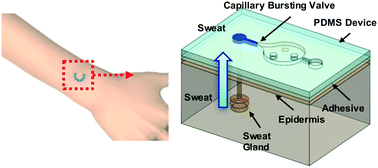
During periods of activity, sweat glands produce pressures associated with osmotic effects to drive liquid to the surface of the skin. The magnitudes of these pressures may provide insights into physiological health, the intensity of physical exertion, psychological stress factors and/other information of interest, yet they are currently unknown due to absence of means for non-invasive measurement. This paper introduces a thin, soft wearable microfluidic system that mounts onto the surface of the skin to enable precise and routine measurements of secretory fluidic pressures generated at the surface of the skin by eccrine sweat glands (surface SPSG, or s-SPSG) at nearly any location on the body. These platforms incorporate an arrayed collection of unit cells each of which includes an opening to the skin, an inlet through which sweat can flow, a capillary bursting valve (CBV) with a unique bursting pressure (BP), a corresponding microreservoir to receive sweat and an outlet to the surrounding ambient to allow release of backpressure. The BPs systematically span the physiologically relevant range, to enable a measurement precision approximately defined by the ratio of the range to the number of unit cells. Human studies demonstrate measurements of s-SPSG under different conditions, from various regions of the body. Average values in healthy young adults lie between 2.4 and 2.9 kPa. Sweat associated with vigorous exercise have s-SPSGs that are somewhat higher than those associated with sedentary activity. For all conditions, the forearm and lower back tend to yield the highest and lowest s-SPSGs, respectively.


 Please wait while we load your content...
Please wait while we load your content...